Talks of Representation Theory in Roma "Tor Vergata"

rotating-knot

- ARTS -
Algebra and Representation Theory Seminar

(ART home page) in Roma "Tor Vergata" (ART home page)

rotating-knot

☆ last updated: October 10th, 2025 - Fabio Gavarini ☆

Friday, December 5th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Valerio MELANI [t.b.c.]
(Università di Firenze)

"TBA"

Abstract: T.B.A.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, December 5th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Chris MILIONIS
(Newcastle University)

"TBA"

Abstract: T.B.A.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, November 21th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Bernard LECLERC
(Université de Caen)

"TBA"

Abstract: T.B.A.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, November 21th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Matilde MACCAN
(Ruhr-Universität Bochum)

"TBA"

Abstract: T.B.A.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, November 7th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Lucia BAGNOLI
("Sapienza" Università di Roma)

"TBA"

Abstract: T.B.A.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, November 7th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Alessio CIPRIANI
(Università di Verona)

"TBA"

Abstract: T.B.A.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, October 24th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Giovanni CERULLI IRELLI
("Sapienza" Università di Roma)

"TBA"

Abstract: T.B.A.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, October 24th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Antonio MITI
("Sapienza" Università di Roma)

"Construction and Reduction of the Lie infinity Algebra of Observables associated with a BV-Module"

Abstract: Multisymplectic manifolds generalize symplectic manifolds by featuring a closed nondegenerate differential form of degree higher than 2. Such structures are natural candidates for a geometric formalization of classical field theories. In this context, Rogers (2010) showed that just as a symplectic manifold yields a Poisson algebra of functions, an n-plectic manifold yields an n-terms Lie infinity algebra of observables.
The remarkable aspect of Rogers' construction is that it is essentially algebraic and relies only on the axioms of Cartan calculus, suggesting that this higher version of the "observable Poisson algebra" can be generalized beyond the realm of manifolds.
In this talk, we propose such a generalization in the setting of Gerstenhaber algebras and Batalin–Vilkovisky (BV) modules, which provide an algebraic formulation of Cartan calculus of interest in the context of non-commutative geometry. This framework allows us to construct Lie infinity algebras of observables in a purely algebraic way, without reference to an underlying manifold.
As an application, we turn to the problem of reducing multisymplectic observables in the presence of constraints or symmetries. Building on the work of Dippel, Esposito, and Waldmann, who introduced the notion of a "constraint triple" as a categorical package for coisotropic reduction, we adapt this formalism to our BV-module context and the associated Lie infinity algebras.
This construction provides a conceptual framework for the algebraic reduction procedure of multisymplectic observables, as developed in our recent joint work with Casey Blacker (SIGMA 2024).
The results presented here are part of a collaboration with Leonid Ryvkin, published in "Differential Geometry and its Applications" (2025).
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, October 10th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Jiefeng LIU
(Northeast Normal University, Changchun)

"Cohomology of restricted Poisson algebras in characteristic 2"

Abstract: In this talk, I will introduce restricted Poisson algebras in characteristic 2 and explore their connection with restricted Lie-Rinehart algebras. For the latter, a cohomology theory is developed and abelian extensions are investigated. I will also construct a cohomology complex for restricted Poisson algebras in characteristic 2 that controls formal deformations. This complex is shown to be isomorphic to the cohomology complex of a suitable restricted Lie-Rinehart algebra. Several examples are provided to illustrate the constructions.
This is a joint work with Sofiane Bouarroudj and Quentin Ehret.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, October 10th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Luca CASARIN
("Sapienza" Università di Roma)

"The factorizable Feigin-Frenkel center"

Abstract: Given a simple finite Lie algebra over the complex numbers, we can consider two other Lie algebras attached to it: its Langlands dual Lie algebra and the affine algebra at the critical level. It is a theorem of the nineties, by Feigin and Frenkel, that the center of the completed enveloping algebra of the affine algebra at the critical level is canonically isomorphic to the algebra of functions on the space of Opers on the pointed disk for the Langlands dual Lie algebra. These objects are actually pointwise instances of a more general picture: the space of opers for example enhances to a space which lives over an arbitrary smooth curve that is equipped with a natural factorization structure. This structure is fundamental for the Geometric Langlands community: factorization patterns allow for local to global arguments. In this talk I will explain the construction of the objects mentioned above and elaborate on a joint work with Andrea Maffei in which we prove the factorizable version of the Feigin-Frenkel theorem.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, September 26th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Francesca PAGANELLI
("Sapienza" Università di Roma)

"Quantum cluster algebras and representations of shifted quantum affine algebras"

Abstract: Shifted quantum affine algebras are quantum groups parameterized by a coweight of the underlying Lie algebra. In 2022, Hernandez introduced a category O of representations of these algebras, and in 2024, Geiss–Hernandez–Leclerc proved that the Grothendieck ring of this category O admits a cluster algebra structure. In this talk, after introducing the necessary background, I will explain how to construct a quantization of this cluster algebra, leading to a definition of the quantum Grothendieck ring for category O.
If time permits, I will also discuss some applications and directions for future research.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, September 26th, 2025
h. 14:30 - Room "Roberta Dal Passo"
(joint session with the "Topology Seminar")

Alberto AVITABILE
(Università di Roma "Tor Vergata")

"E_∞-algebra structure on singular cochains"

Abstract: Singular cohomology of a space carries a natural CDGA structure induced by cup product at the level of cochains. While cup product is a well-defined operation on singular cochains, commutativity only holds after passing to cohomology, due to the presence of non-trivial Steenrod operations. This fact will be the motivating example for introducing E_∞-algebras and to explain how the E_∞-structure on cochains encodes this phenomenon in a precise way. Since the theory of E_∞-algebras needs the notion of operad, general recollections on the basics of operad theory will be provided.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, June 20th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Nicolas RESSAYRE
(Université Lyon 1)

"An algorithm to compute the moment cones"

Abstract: Let V be a representation of a connected complex reductive group G. The group acts on the ring of regular functions on V: the asymptotic support of this representation is a closed convex polyhedral cone, called moment cone. We will present an algorithm that determines the minimal list of linear inequalities for this cone. Some aspects are relevant from algorithm and convex geometry and others from algebraic geometry.
Joint work with Michael Bulois and Roland Denis.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, June 20th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Thomas GERBER
(Université Lyon 1)

"Atomic length on Weyl groups"

Abstract: In this talk, I will introduce a new statistic on Weyl groups called the atomic length, and clarify this terminology by drawing parallels with the usual Coxeter length.
It turns out that the atomic length has a natural Lie-theoretic interpretation, based on crystal com-binatorics, that I will present.
Last but not least, I will explain how this can be used as a tool for tackling a broad range of enu-meration problems arising from modular representation theory (and related to the study of core partitions).
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Thursday, June 5th, 2025
(( beware of the change of day and room!!! ))
h. 14:30 - Room 1200 (Biblioteca Storica)
N.B.: joint session with the "Topology Seminar"

Waclaw MARZANTOWICZ
(University of Poznań)

"Reeb graphs and description of homomorphisms onto the free groups"

Abstract: The Reeb graph R(f) of a C¹-function f from M to the real numbers with isolated critical points is a quotient object by the identification of connected components of function levels which has a natural structure of graph. The quotient map p from M to R(f) induces a homomorphism p^* from the fundamental group of M to the fundamental group of R(f) which is equal to F_r, the free group on r generators. This leads to the natural question whether every epimorphism from a finitely presented group G to F_r can be represented as the Reeb epimorphism p^* for a suitable Reeb (or even Morse) function f. We present a positive answer to this question. This is done by use of a construction of correspondence between epimorphisms from the fundamental group of M to F_r and systems of r framed non-separating hypersurfaces in M, which induces a bijection onto their framed cobordism classes. As applications we provide new purely geometrical-topological proofs of some algebraic facts.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, May 23rd, 2025
h. 14:30 - Room "Roberta Dal Passo"

Santosha Kumar PATTANAYAK
(Indian Institute of Technology - Kanpur)

"Uniqueness of branching and unique factorization of tensor products of typical representations of Lie superalgebras"

Abstract: A theorem of Rajan says that a tensor product of irreducible, finite dimensional representations of a simple Lie algebra over a field of characteristic zero determines individual constituents uniquely. This is analogous to the uniqueness of prime factorization of natural numbers. We discuss a more general question of determining all the pairs (V₁ , V₂) consisting of two finite dimensional irreducible representations of a semisimple Lie algebra g such that Res(g₀)|V₁ ≅ Res(g₀)|V₂ , where g₀ is the fixed point subalgebra of g with respect to a finite order automorphism.
We will also discuss the above tensor product problem in the category of typical representations of basic classical Lie superalgebras.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, April 11th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Tommaso SCOGNAMIGLIO
(Universität Heidelberg)

"PGL(2)-character varieties and Langlands duality over finite fields"

Abstract: For a Riemann surface X and a complex reductive group G, G-character varieties are moduli spaces parametrizing G-local systems on X. When G=GL_n, the cohomology of these character varieties have been deeply studied and under the so-called genericity assumptions, their cohomology admits an almost full description, due to Hausel, Letellier, Rodriguez-Villegas, and Mellit. An interesting aspect is that the geometry of these varieties is related to the representation theory of the finite group GL_n(F_q). We expect in general that G-character varieties should be related to G(F_q)-representation theory, where G(F_q) is the Langlands dual.
In the beginning of the talk, I will recall the results concerning GL_n. Then, I will explain how to generalize some of these results when G=PGL₂ . In particular, we will see how to relate PGL₂-character varieties and the representation theory of SL₂(F_q).
This is joint work with Emmanuel Letellier.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, March 28th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Filippo AMBROSIO
(Friedrich-Schiller-Universität Jena)

"Étale geometry of Jordan classes closures"

Abstract: Let G be a connected reductive algebraic group over an algebraically closed field k. Lusztig (1984) partitioned G into subvarieties which play a fundamental role in the study of representation theory, the Jordan classes. An analogue partition of the Lie algebra Lie(G) into subvarieties, called decomposition classes, dates back to Borho-Kraft (1979). When k = C the study of geometric properties (e.g., smoothness) of a point g in the closure of a Jordan class J in G can be reduced to the study of the geometry of an element x in the closure of the union of finitely many decomposition classes in Lie( M), where M is a connected reductive subgroup of G depending on g.
The talk aims at introducing such objects and at generalizing this reduction procedure to the case char(k) > 0.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, March 14th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Lydia GÖSMANN
(Ruhr-Universität Bochum)

"Nakajima varieties of quivers"

Abstract: Moduli spaces of representations associated to quivers are algebraic varieties encoding the continuous parameters of linear algebraic classification problems. In recent years their topological and geometric properties have been explored to investigate wild quiver classification problems.
The goal of this talk is the construction of the Nakajima variety of a quiver as one of these moduli spaces. Starting with basic definitions from the representation theory of quivers, fundamental concepts like doubling and framing are introduced. Via geometric invariant theory Nakajima varieties can be defined. We will discuss their properties focusing on the example of the quiver consisting of one vertex and no arrows. Finally, further relations to recent research interests are explained.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, February 28th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Damien SIMON
(Université Paris-Saclay)

"Chiral differential operators on a reductive group and representation theory"

Abstract: Vertex algebras of chiral differential operators on a complex reductive group G are "Kac-Moody" versions of the usual algebra of differential operators on G. Their categories of modules are especially interesting because they are related to the theory of D-modules on the loop group of G. That allows one to reformulate some conjectures of the (quantum) geometric Langlands program in the language of vertex algebras. For instance, in view of the geometric Satake equivalence, one may expect the appearance of the category of representations of the Langlands dual group of G.
In this talk I will define this family of vertex algebras and we will see that they are classified by a certain parameter called level. Then, for generic levels, we will see that "to find" the Langlands dual group, it is necessary to perform a quantum Hamiltonian reduction. Finally I will build simple modules on the closely related equivariant W-algebra that match the combinatorics of the Langlands dual group.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, February 28th, 2025
h. 14:30 - Room "Roberta Dal Passo"
N.B.: joint session with the "Topology Seminar"

Andrea PIZZI
(Università di Roma "Tor Vergata")

"(Multi-)Simplicial methods for Configuration Space Recognition"

Abstract: The goal of this talk is to algebraically model the S_r-equivariant homotopy type of the configuration space of r labeled and distinct points in d-dimensional Euclidean space. We will present and compare two models: the Barratt-Eccles simplicial set and the multisimplicial set of 'surjections'. Moreover, we will introduce multisimplicial sets and discuss their connection to more well-known simplicial sets. Multisimplicial sets can model homotopy types using fewer cells, making them a highly useful tool. Following this, we will explore in detail how to recognize configuration spaces in the aforementioned models by playing with a graph poset. An explicit relationship between the models will also be presented.
This is joint work with Anibal M. Medina-Mardones and Paolo Salvatore.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, February 14th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Andrea GUIDOLIN
(University of Southampton)
N.B.: joint session with the "Topology Seminar"

"Algebraic Wasserstein distances and stable homological invariants of data"

Abstract: Persistent homology, a popular method in Topological Data Analysis, encodes geometric information of data into algebraic objects called persistence modules. Invoking a decomposition theorem, these algebraic objects are usually represented as multisets of points in the plane, called persistence diagrams, which can be fruitfully used in data analysis in combination with statistical or machine learning methods.
Wasserstein distances between persistence diagrams are a common way to compare the outputs of the persistent homology pipeline. In this talk, I will explain how a notion of p-norm for persistence modules leads to an algebraic version of Wasserstein distances which fit into a general framework for producing distances between persistence modules. I will then present stable invariants of persistence modules which depend on Wasserstein distances and can be computed efficiently. The use of these invariants in a supervised learning context will be illustrated with some examples.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, February 14th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Alessandro CAROTENUTO
(Università di Parma)

"Complex geometry of the full quantum flag manifold of quantum SU(3)"

Abstract: The noncommutative differential geometry of quantum flag manifolds has seen rapid growth in recent years, following the remarkable finding of a complex structure for flag manifolds of irreducible type by Heckenberger and Kolb. With a large part of the theory for the irreducible cases already figured out, it is now time to tackle the question of how to obtain the same structure for other types of flag manifolds. In this work in collaboration with R. Ó Buachalla and J. Razzaq, we give a complex structure for the full flag manifold of quantum SU(3), that includes the differential calculus discovered by Ó Buachalla and Somberg as its holomorphic sub-complex.
I shall review this construction that makes use of Lusztig quantum root vectors, while at the same time giving a general overview of the theory of noncommutative differential calculi for quantum homogeneous spaces.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, January 17th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Frédéric PATRAS
(Université Côte d'Azur)

"How to recognize free Lie algebras?"

Abstract: Structure properties of free Lie algebras are a fundamental tool in group theory and its many applications, however it is not always easy in practice to recognize that a Lie algebra is free. The talk will survey various results that allow to conclude to freeness, and various concrete examples.
Based on joint work with L. Foissy.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

rotating-knot

Friday, December 13th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Paolo ANTONINI
(Università del Salento)

"Optimal Transport between Algebraic Hypersurfaces"

Abstract: I will report on a recent work in collaboration with F. Cavalletti and A. Lerario, where we study complex projective hypersurfaces seen as probability measures on the projective space. Our guiding question is: “What is the best way to deform a complex projective hypersurface into another one?" Here the word best means from the point of view of measure theory and mass optimal transportation. In particular, we construct an embedding of the space of complex homogeneous polynomials into the probability measures on the projective space and study its intrinsic Wasserstein metric.The Kähler structure of the projective space plays a fundamental role and we combine different techniques from symplectic geometry to the Benamou-Brenier dynamical approach to optimal transportation to prove several interesting facts. Among them we show that the space of hypersurfaces with the Wasserstein metric is complete and geodesic: any two hypersurfaces (possibly singular) are always joined by a minimizing geodesic. Moreover outside the discriminant locus, the metric is induced by a Kähler structure of Weil-Petersson type. In the last part I will give an application to the condition number of polynomial equations solving.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, December 13th, 2024
h. 14:30 - Room "Roberta Dal Passo"
N.B.: joint session with the "Topology Seminar"

Maxime RAMZI
(Universität Münster)

"Induced character formulae and the Becker-Gottlieb transfer"

Abstract: The induced character formula in classical representation theory can be used, among other things, to describe the dimension of coinvariants of a representation in terms of its character. In this talk, I will explain how this formula is related to the multiplicativity of Euler characteristics in algebraic topology, and, in a more homotopy-coherent context, to the composability of so-called Becker-Gottlieb transfers, which are "wrong-way maps" in singular (co)homology; by describing a general formula to compute "dimensions of homotopy colimits". If time permits, I will discuss the most general case in which composability of Becker-Gottlieb transfers is now known. This is based on joint works with Carmeli-Cnossen-Yanovski, Klein-Malkiewich (and the last part with Volpe-Wolf).
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, November 29th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Özgür CEYHAN
(Université du Luxembourg)

"Tropical Neural Networks"

Abstract: The age of AI requires building capable and more efficient neural networks that are mainly achieved via:
(I) developing and manufacturing more capable hardware; (II) designing smaller and more robust versions of neural networks that realize the same tasks; (III) reducing the computational complexities of learning algorithms without changing the structures of neural networks or hardware.
The approach (I) is an industrial design and manufacturing challenge. The approach (II) is essentially the subject of network pruning. In this talk, we play on mathematicians' strengths and focus on a theoretical approach on (III) based on tropical arithmetics and geometry.
I will first describe the setup of machine learning in simple mathematical terms and briefly introduce tropical geometry. After verifying that tropicalization will not affect the classification capacity of deep neural networks, I will discuss a tropical reformulation of backpropagation via tropical linear algebra.
This talk assumes no preliminary knowledge of machine learning or tropical geometry: undergraduate-level math, and general curiosity will be sufficient for active participation.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, November 29th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Connor MALIN
(Max Planck Institute)

"A scanning map for the E_n operad"

Abstract: For a framed n-manifold M one can produce an explicit pairing between M and its one point compactification M⁺, taking values in Sⁿ, which on homology induces the Poincaré duality pairing. We show that this can be lifted to the level of operads to produce a stable equivalence between E_n , the little n-disks operad, and a shift of its Koszul dual. This gives a proof of the same celebrated result of Ching-Salvatore, but manages to avoid using technical results in geometry, homotopy theory, and even basic analysis that appear in their proof.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, November 15th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Alfonso TORTORELLA
(Università di Salerno)

"Deformations of Symplectic Foliations via Dirac Geometry and L_∞-Algebra"

Abstract: In this talk, based on joint work with Stephane Geudens and Marco Zambon, we develop the deformation theory of symplectic foliations, i.e. regular foliations equipped with a leafwise symplectic form. The main result is that each symplectic foliation is attached with a cubic L_∞-algebra controlling its deformation problem. Indeed, we establish a one-to-one correspondence between the small deformations of a given symplectic foliation and the Maurer-Cartan elements of the associated L_∞-algebra. Further, we prove that, under this one-to-one correspondence, the equivalence by isotopies of symplectic foliations agrees with the gauge equivalence of Maurer-Cartan elements. Finally, we show that the infinitesimal deformations of symplectic foliations can be obstructed.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, November 15th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Marco MORASCHINI
(Università di Bologna)

"An introduction to amenability in bounded cohomology"

Abstract: Bounded cohomology of groups is a variant of ordinary group cohomology introduced by Johnson in the 70s in the context of Banach algebras and then intensively studied by Gromov in his seminal paper "Volume and bounded cohomology" in relation to geometry and topology of manifolds. Since the 80s, bounded cohomology has then grown up as an independent and active research field. On the other hand, it is notoriously hard to compute bounded cohomology. For this reason it is natural to first investigate groups with trivial bounded cohomology groups. During this talk we survey recent advances around "acyclicity" in bounded cohomology and we will introduce a new algebraic criterion for the vanishing of bounded cohomology.
This is part of a joint work with Caterina Campagnolo, Francesco Fournier-Facio and Yash Lodha.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, October 25th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Anne MOREAU
(Université Paris-Saclay)

"On a series of simple affine VOAs arising from rank one 4D SCFTs"

Abstract: It is known by the works of Adamović and Perše that the affine simple vertex algebras associated with G₂ and B₃ at level -2 can be conformally embedded into L_-2(D₄).
In this talk, I will present a join work with Tomoyuki Arakawa, Xuanzhong Dai, Justine Fasquel, Bohan Li on the classification to the irreducible highest weight modules of these vertex algebras.
I will also describe their associated varieties: the associated variety of that corresponding to G₂ is the orbifold of the associated variety of that corresponding to D₄ by the symmetric group of degree 3 which is the Dynkin diagram automorphism group of D₄. This provides new interesting examples in the context of orbifold vertex algebras. These vertex algebra also appear as the vertex operator algebras corresponding to rank one Argyres-Douglas theories in four dimension with flavour symmetry G₂ and B₃.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, October 25th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Linda HOYER
(RWTH Aachen University)

"Orthogonal Determinants of Finite Groups"

Abstract: Let G be a finite group. It is not hard to see that for any representation ρ : G ⟶ GL(V) for V a real vector space, there exists a G-invariant bilinear form β on V, i.e., a non-degenerate bilinear form such that β(ρ(gv,ρ(g)w) = β(v,w) for all g ∈ G, v, w ∈ V. If ρ is "orthogonally stable" (so it is a sum of even-dimensional irreducible real representations) then the square class of the determinant of the Gram matrix for any basis (the "orthogonal determinant") does not depend on the choice of β, giving us interesting invariants of our group G. Richard Parker conjectured that these orthogonal determinants are always "odd", for any finite group. We will see that the conjecture holds for the symmetric groups, as well as the general linear groups GL(q) for q a power of an odd prime. In the discussion, important concepts like (standard) Young tableaux and Iwahori-Hecke algebras will come up. This talk has the additional purpose of giving a small introduction (with many examples) into the representation theory of finite groups. As such, no previous knowledge in that area will be assumed.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, October 11th, 2024
h. 14:30 - Room "Claudio D'Antoni"
N.B.: mind the change of room!

Thibault JUILLARD
(Université Paris-Saclay)

"Reduction by stages for affine W-algebras"

Abstract: Affine W-algebras form a family of vertex algebras indexed by the nilpotent orbits of a simple finite dimensional complex Lie algebra. Each of them is built as a noncommutative Hamiltonian reduction of the corresponding affine Kac-Moody algebra. In this talk, I will present a joint work with Naoki Genra about the problem of reduction by stages for these affine W-algebras: given a suitable pair of nilpotent orbits in the simple Lie algebra, it is possible to reconstruct one of the two affine W-algebras associated to these orbits as the Hamiltonian reduction of the other one. I will insist on how this problem relates to our previous work about reduction by stages between Slodowy slices, which are Poisson varieties associated with affine W-algebras. I will also mention some applications and motivations coming from Kraft-Procesi rule for nilpotent Slodowy slices, and isomorphisms between simple affine admissible W-algebras.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, September 27th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Gastón Andrés GARCÍA
(Universidad Nacional de La Plata / CONICET)

"Hopf algebras and finite simple groups of Lie type"

Abstract: Hopf algebras (and variations of them) are the algebraic counterpart of (strict, rigid) tensor categories. As such, they appear as symmetries of different categorial, geometrical and physical objects. In particular, several applications may be found in diverse areas of mathematics, physics and theoretical computer sciences.
Hopf algebras were already studied in the 60's and had a big impulse in the 80's after the work of Drinfeld on quantum groups. Despite more than 60 years of study, not much is known about them: general results are sparse and the classification is only known for (quite) small dimensions or for families with different properties.
This talk will be about a joint project with N. Andruskiewitsch and G. Carnovale in our attempt to determine finite-dimensional pointed Hopf algebras over finite-simple groups of Lie type. The main idea we exploit is the reduction of the problem to group-theoretical criteria to determine the finite-dimensionality of our objects, which boils down to the use of different properties of conjugacy classes, root systems and computational tools.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, September 27th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Giulio RICCI

"A brief introduction to Bruhat-Tits theory and its applications"

Abstract: We will review some theory of algebraic groups over Q_p and the construction of the Bruhat-Titts building for a split group G over Q_p . At the end, we will see some applications and we will mention some results about disconnected groups.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, September 13th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Sabino DI TRANI
("Sapienza" Università di Roma)

"Graph Cohomologies, Matroids and Colorings"

Abstract: A celebrated result in graph theory links the chromatic polynomial of a graph to the Tutte polynomial of the associated graphic matroid. In 2005, Helme-Guizon and Rong proved that the chromatic polynomial is categorified by a cohomological theory called chromatic cohomology.
In this talk, I will describe how to associate a matroid to a directed graph G, called the multipath matroid of G, which encodes relevant combinatorial information about edge orientation. We also show that a specialization of the Tutte polynomial of the multipath matroid of G provides the number of certain "good" digraph colorings.
Finally, analogously to the relationship between the chromatic polynomial and chromatic cohomology, I will show how the polynomial expressing the number of "good" digraph colorings is linked to multipath cohomology, introduced in a work with Caputi and Collari in 2021.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, September 13th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Gastón Andrés GARCÍA
(Universidad Nacional de La Plata / CONICET)

"On the representation theory of generalized small quantum groups"

Abstract: The small quantum groups u_q(g) are finite-dimensional quotients of quantum universal enveloping algebras U_q(g) at a root of unity q for g a semisimple complex Lie algebra. After the work of Lusztig, the representation theory of these quantum objects was intensively studied because of its relation with the representation theory of semisimple algebraic groups in positive characteristic. In this talk, I will present some results on the representation theory of what we call "generalized" small quantum groups. A particular feature of these objects is that the role of the corresponding Cartan subalgebra is played by a finite non-abelian group. Nevertheless, they still admit a triangular decomposition and share similar properties with the standard quantum groups, like the existence of weights (that are no longer one-dimensional) and Verma modules.
This talk is based on a joint work with Cristian Vay [Simple modules of small quantum groups at dihedral groups, Doc. Math. 29 (2024), 1-38].
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Monday, July 29th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Marianne DÉGLISE
(ENS Paris Saclay)
"Combinatorial study of coefficients of the decomposition theorem for universal linear degenerations"

Abstract: We study a geometric/combinatorial characterisation of supports for linear degenerations of flag varieties in an attempt to determine the coefficients of the decomposition theorem. We show that it behaves correctly when applying the operation suspension, and obtain positive results in the PBW-locus.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, July 5th, 2024 - ARTS Midsummer Day Edition
h. 16:00 - Room "Roberta Dal Passo"

Ping XU
(Pennsylvania State University)
"Derived differentiable manifolds"

Abstract: One of the main motivations behind derived differential geometry is to deal with singularities arising from zero loci or intersections of submanifolds. Both cases can be considered as fiber products of manifolds which may not be smooth in classical differential geometry. Thus, we need to extend the category of differentiable manifolds to a larger category in which one can talk about "homotopy fiber products". In this talk, we will discuss a solution to this problem in terms of dg-manifolds. The talk is mainly based on a joint work with Kai Behrend and Hsuan-Yi Liao.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, July 5th, 2024 - ARTS Midsummer Day Edition
h. 14:30 - Room "Roberta Dal Passo"

Mathieu STIÉNON
(Pennsylvania State University)
"Formal geometry of groupoids"

Abstract: I will give a brief survey of the formal geometry of groupoids.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, June 7th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Paolo ASCHIERI
(Università del Piemonte Orientale - Alessandria)
"Bundles on quantum projective varieties and their differential geometry"

Abstract: We study quantum principal bundles on projective varieties using a sheaf theoretic approach. Differential calculi are introduced in this context. The main class of examples is given by covariant calculi over quantum flag manifolds, which we provide via an explicit Ore extension construction. We next introduce principal covariant calculi by requiring a local compatibility of the calculi on the total sheaf, base sheaf and the structure Hopf algebra in terms of exact sequences. The examples of principal (covariant) calculi on the quantum principal bundles SL_q(2,C) and GL_q(2,C) over the projective space P¹(C) are presented.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, May 24th, 2024
h. 16:00 - Room "Claudio D'Antoni"
N.B.: mind the change of room!

Ulrich KRÄHMER
(UT Dresden)
"The ring of differential operators on a monomial curve is a Hopf algebroid"

Abstract: The ring of differential operators on a cuspidal curve whose coordinate ring is a numerical semigroup algebra is shown to be a cocommutative and cocomplete left Hopf algebroid. If the semigroup is symmetric so that the curve is Gorenstein, it is a full Hopf algebroid (admits an antipode). This is based on joint work with Myriam Mahaman.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, May 24th, 2024
h. 14:30 - Room "Claudio D'Antoni"
N.B.: (1) mind the change of room!
(2) joint session with the department "Topology Seminar"

Giovanni PAOLINI
(Università di Bologna)
"Dual Coxeter groups of rank three"

Abstract: In this presentation, I will discuss the combinatorics of the noncrossing partition posets associated with Coxeter groups of rank three. In particular, I will describe the techniques used to prove the lattice property and lexicographic shellability. These properties can then be used to solve several problems on the corresponding Artin groups, such as the K(π,1) conjecture, the word problem, the center problem, and the isomorphism between standard and dual Artin groups.
This is joint work with Emanuele Delucchi and Mario Salvetti.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, April 19th, 2024
h. 16:00 - Room "Roberta Dal Passo"
N.B.: joint session with the department "Topology Seminar"

Gabriele VEZZOSI
(Università di Firenze)
"Analogs of Beilinson-Drinfeld's Grassmannian on a surface"

Abstract: Beilinson-Drinfeld's Grassmannian on an algebraic curve is an important object in Representation Theory and in the Geometric Langlands Program. I will describe some analogs of this construction when the curve is replaced by a surface, together with related preliminary results.
This is partly a joint work with Benjamin Hennion (Orsay) and Valerio Melani (Florence), and partly a joint work in progress with Andrea Maffei (Pisa) and Valerio Melani (Florence).
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, May 10th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Stefano MARINI
(Università di Parma)
"On finitely Levi nondegenerate closed homogeneous CR manifolds"

Abstract: A complex flag manifold F = G/Q decomposes into finitely many real orbits under the action of a real form of G. Their embeddings into F define CR-manifold structures on them. We give a complete classification of all closed simple homogeneous CR-manifolds that have finitely nondegenerate Levi forms.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, April 19th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Elisabetta MASUT
(Università di Padova)
"Non-existence of integral Hopf orders for certain Hopf algebras"

Abstract: The study of the (non)-existence of integral Hopf orders was originally motivated by Kaplansky's sixth conjecture, which is a generalization of Frobenius theorem in the Hopf algebra setting. In fact, Larson proved that a Hopf algebra which admits an integral Hopf order satisfies the conjecture.
The aim of this talk is to give a partial answer to the following question: "Does a semisimple complex Hopf algebra admit an integral Hopf order?"
In particular, we will present several families of semisimple Hopf algebras which do not admit an integral Hopf order. These Hopf algebras will be constructed as Drinfeld twists of group algebras.
This talk is based on a joint work with Giovanna Carnovale and Juan Cuadra and on my Ph.D. thesis.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, April 19th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Martina COSTA CESARI
(Università di Bologna)
"Jordan classes and Lusztig strata in non-connected algebraic groups"

Abstract: Reductive non-connected groups appear frequently in the study of algebraic groups, for example as centralizers of semisimple elements in non-simply connected semisimple groups. Let G be a non-connected reductive algebraic group over an algebraically closed field of arbitrary characteristic and let D be a connected component of G. We consider the strata in D defined by G. Lusztig as fibers of a map E given in terms of truncated induction of Springer representation. By the definition of the map E, one can see that elements with the same unipotent part and the same centralizer of the semisimple part are in the same stratum. The connected component of the set collecting the elements with these properties are called Jordan classes. In his work, G. Lusztig suggests that the strata are locally closed: in my work I prove this assertion. To prove it, I show that a stratum is a union of the regular part of the closure of Jordan classes. From this result, one can also describe the irreducible components of a stratum in terms of regular closures of Jordan classes.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, April 12th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Grant BARKLEY
(University of Harvard)
"Hypercube decompositions and combinatorial invariance for elementary intervals"

Abstract: The combinatorial invariance conjecture asserts that the Kazhdan-Lusztig (KL) polynomial of an interval [u,v] in Bruhat order can be determined just from the knowledge of the poset isomorphism type of [u,v]. Recent work of Blundell, Buesing, Davies, Velicković, and Williamson posed a conjectural recurrence for KL polynomials depending only on the poset structure of [u,v]. Their formula uses a new combinatorial structure, called a hypercube decomposition, that can be found in any interval of the symmetric group. We give a new, simpler, formula based on hypercube decompositions and prove it holds for "elementary" intervals: an interval [u,v] is elementary if it is isomorphic as a poset to an interval with linearly independent bottom edges. As a result, we prove combinatorial invariance for Kazhdan-Lusztig R-polynomials of elementary intervals in the symmetric group, generalizing the previously known case of lower intervals.
This is a joint work with Christian Gaetz.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, April 12th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Willem DE GRAAF
(Università di Trento)
"Classifying orbits of complex and real Vinberg representations"

Abstract: Vinberg representations are representations of algebraic groups that arise from a cyclic grading of a semisimple Lie algebra. In the literature they are mainly known as theta-groups or Vinberg pairs. A distinguishing feature of these representations is that it is possible to classify the orbits of the algebraic group. We sketch how this can be done when the base field is the complex numbers. This mainly uses results of Vinberg of the 70's. Then we describe techniques for classifying the orbits when the base field is the real numbers. This talk is based on joint work with Mikhail Borovoi, Hong Van Le, Heiko Dietrich, Marcos Origlia, Alessio Marrani.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, March 15th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Christophe HOHLWEG
(Université du Quebec à Montréal)
"Shi arrangements in Coxeter groups"

Abstract: Given an arbitrary Coxeter system (W,S) and a nonnegative integer m, the m-Shi arrangement of (W,S) is a subarrangement of the Coxeter hyperplane arrangement of (W,S). The classical Shi arrangement (m=0) was introduced in the case of affine Weyl groups by Shi to study Kazhdan-Lusztig cells for W. As two key results, Shi showed that each region of the Shi arrangement contains exactly one element of minimal length in W and that the union of their inverses form a convex subset of the Coxeter complex. The set of m-low elements in W was introduced to study the word problem of the corresponding Artin-Tits (braid) group and they turn out to produce automata to study the combinatorics of reduced words in W.
In this talk, I will discuss how to Shi's results extend to any Coxeter system and show that the minimal elements in each Shi region are in fact the m-low elements. This talk is based on joint work with Matthew Dyer, Susanna Fishel and Alice Mark.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

ball-Moebius

Friday, March 15th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Anna MICHAEL
(Universität Magdeburg)
"Folded galleries - a museum tour through 192 years of math history"

Abstract: Folded galleries, as introduced by Peter Littelmann in the 1990s, are combinatorial objects related to certain (subsets of) elements of Coxeter groups. They have shown to have versatile applications in algebra and geometry, making them an object of interest for current research. In this talk we will retrace the roots of their invention 192 years back in history, contemplate colorful illustrations of examples, and discover open questions for future applications.
N.B.: (1) the talk will be colloquium-style and aimed at a wide audience: no prerequisite of deep algebraic nor group theoretic knowledge is required;
(2): this talk is part of the activity of the MIUR Excellence Department Project MatMod@TOV (CUP E83C23000330006).

Calabi-spin

Friday, March 1st, 2024
h. 16:00 - Room "Roberta Dal Passo"

Matteo MICHELI
("Sapienza" Università di Roma)
"Degenerations of the classical Grassmannians and their isotropic subvarieties"

Abstract: This talk is based on joint work in progress with E. Feigin, M. Lanini and A. Pütz.
We analyze a family of Quiver Grassmannians for the equioriented cycle, which are degenerations of the classical Grassmannians: for each one, we describe its irreducible components, find a cellular decomposition in terms of attracting sets, and give an overview of the underlying combinatorics. Then we introduce symplectic conditions and try to understand the associated subvarieties, which are degenerations of the classical isotropic Grassmannians.

ball-Moebius

Friday, March 1st, 2024
h. 14:30 - Room "Roberta Dal Passo"

Timm PEERENBOOM
(Ruhr-Universität - Bochum)
"CoHas of extended Dynkin quivers"

Abstract: In this talk I give a description of the semistable Cohomological Hall algebra (CoHa) for extended Dynkin quivers with central slope in terms of generators and relations.
This extends work of Franzen-Reineke who dealt with the case of the Kronecker quiver.

Calabi-spin

Friday, February 16th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Loic FOISSY
(LMPA-ULCO Calais)
"Cointeracting bialgebras and applications to graphs"

Abstract: Pairs of cointeracting bialgebras appear recently in the literature of combinatorial Hopf algebras, with examples based on formal series, on trees (Calaque, Ebrahimi-Fard, Manchon and Bruned, Hairer, Zambotti), graphs (Manchon), posets... These objects have one product (a way to combine two elements in a single one) and two coproducts (the first one reflecting a way to decompose a single element into two parts, maybe into several ways, the second one reflecting a way to contract parts of an element in order to obtain a new one). All these structures are related by convenient compatibilities.
We will give several results obtained on pairs of cointeracting bialgebras: actions on the group of characters, antipode, polynomial invariants... and we will give applications to a Hopf algebra of graphs, including the Fortuin and Kasteleyn's random cluster model, a variation of the Tutte polynomial.

ball-Moebius

Friday, February 16th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Rui XIONG
(University of Ottawa)
"Pieri rules over Grassmannians"

Abstract: The classical Pieri rule is a multiplication formula for Schubert class and Chern classes of the tautological bundle. Combinatorially, it is given by adding a chain of boxes on partitions. In this talk, we will discuss its generalization to equivariant Motivic Chern classes and its dual basis Segre motivic classes. Our formula is in terms of ribbon Schubert operators, which is roughly speaking adding ribbons on partitions. As an application, we have found a little surprising relation between motivic Chern classes and Segre motivic classes, extending the relation between ideal sheaves and structure sheaves over Grassmannian.

Calabi-spin

Friday, February 2nd, 2024
h. 16:00 - Room "Roberta Dal Passo"

Giovanni CERULLI IRELLI
("Sapienza" Università di Roma)
"Specialization map for quiver Grassmannians"

Abstract: We define a specialization map for quiver Grassmannians of Dynkin type and prove that it is surjective in type A. This generalizes a beautiful theorem of Lanini and Strickland concerning the cohomology of degenerate flag varieties.
This is a joint ongoing work with Francesco Esposito, Ghislain Fourier and Fang Xin.

ball-Moebius

Friday, February 2nd, 2024
h. 14:30 - Room "Roberta Dal Passo"

Roberto FRINGUELLI
("Sapienza" Università di Roma)
"Zastava Spaces via non-degenerate maps"

Abstract: Let k be a field, G be a reductive group over k, B be a Borel subgroup of G and C be a smooth curve over k. The B-orbit stratification of the flag variety G/B induces a natural stratify-cation on the moduli space of maps from C to G/B . The open stratum is strictly related to the so-called Zastava spaces.
In this talk, we give an overview of the main properties of these spaces. If time permits, we also present some consequences on the moduli space of G-bundles.

Calabi-spin

Friday, January 19th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Tommaso ROSSI
(Università di Roma "Tor Vergata")
"Homology operations for gravity algebras"

Abstract: In the early nineties Getzler discovered a nice algebraic structure on the equivariant homology of a topological conformal field theory. He called this algebraic structure a "gravity algebra" and he showed that it is governed by an operad which is closely related to the homology of M_0,n+1 , the moduli space of genus zero Riemann surfaces with n+1 marked points. A gravity algebra can be thought as a generalization of a (dg) Lie algebra, in the sense that other than the Lie bracket we also have higher arity operations which satisfies a "generalized Jacobi identity".
In this talk we will first give an introduction to gravity algebras, providing many interesting examples from both algebra (cyclic cohomology of a Frobenius algebra) and topology (S¹-equivariant homology of the free loop space on a manifold). Then I will briefly explain that any class in the S¹-equivariant homology of the (unordered) configuration spaces of points in the plane H_*^S¹(C_n(R);F_p) (with coefficients in a field F_p of p elements) gives rise to an homology operation for gravity algebras. After that we will see how to compute this equivariant homology and if time permits we will see some applications.

ball-Moebius

Friday, January 19th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Riccardo ARAGONA
(Università de L'Aquila)
"Normalizer chain, modular idealizer chain and partitions"

Abstract: In recent joint works with Civino, Gavioli and Scoppola, we studied the conjugacy classes of an elementary Abelian regular subgroup T of the symmetric group on 2ⁿ elements. In particular we computed, via GAP software package, a chain of normalizers in a Sylow 2-subgroup of this symmetric group defined iteratively, starting from T. We noticed that the logarithm of the indice of the (i-1)-th normalizer in the i-th normalizer of our chain is equal to the i-th partial sum of the sequence of the numbers of partitions of an integer in at least two distinct parts.
In this talk we present some techniques developed in order to prove this result, including the notion of a special family of elements of a Sylow 2-subgroup, called rigid commutators. Finally, some generalizations to Lie algebras are given, considering similar results for an idealizer chain.

rotating-knot

Friday, December 15th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Tiziano GAIBISSO
(Imperial College, London)
"Nakajima quiver varieties"

Abstract: Nakajima quiver varieties, originally defined in '94 by H. Nakajima, form an interesting class of algebraic varieties with many applications in algebraic geometry (e.g. resolution of singularities), representation theory (e.g. Kac-Moody algebras), and string theory (e.g. Coulomb and Higgs branches). In this talk, we will begin introducing the general setting of Hamiltonian reductions via GIT, highlighting how this technique produces Poisson quasi-projective varieties in a canonical way, and, in some cases, resolutions of symplectic singularities. We will then apply this theory to quiver representations, defining Nakajima quiver varieties and illustrating how the combinatorial nature of quivers is reflected in the geometry of these varieties.

ball-Moebius

Friday, December 15th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Chetan VUPPULURY
("Sapienza" Università di Roma)
"Higher projective representations and higher central extensions"

Abstract: Projective representations of a group G with assigned 2-cocycle α are equivalent to (certain) representations of the central extension of G associated with α. This classical result can be seen as a piece of 2-category theory fallen into the realm of 1-categories, and in this perspective it admits natural generalizations relevant to the context of anomalous topological or Euclidean QFTs. In particular, Stolz-Teichner's Clifford field theories naturally emerge as a particular example of this construction.

Calabi-spin

Friday, December 1st, 2023
h. 16:00 - Room "Roberta Dal Passo"

Sophie CHEMLA
(Université Sorbonne - Paris Cité)
"Duality properties for induced and
coinduced representations in positive characteristic"

Abstract: Let k be a field of positive characteristic p>2. We explain a duality property concerning the kernel of coinduced representations of Lie k-(super)algebras. This property was already proved by M. Duflo for Lie algebras in any characteristic under more restrictive finiteness conditions. It was then generalized to Lie superalgebras in characteristic 0 in previous works.
In characteristic 0, it is known that the induced representation can be realized as the local cohomology with coefficients in some coinduced representation. In positive characteristic, in the case of a restricted Lie algebra, we prove a similar result for the restricted induced representation.

ball-Moebius

Friday, December 1st, 2023
h. 14:30 - Room "Roberta Dal Passo"

Victoria SCHLEIS
(University of Tübingen)
"Tropical Quiver Grassmannians"

Abstract: Grassmannians and flag varieties are important moduli spaces in algebraic geometry. Quiver Grassmannians are generalizations of these spaces arise in representation theory as the moduli spaces of quiver subrepresentations. These represent arrangements of vector subspaces satisfying linear relations provided by a directed graph.
The methods of tropical geometry allow us to study these algebraic objects combinatorially and computationally. We introduce matroidal and tropical analoga of quivers and their Grassmannians obtained in joint work with Alessio Borzì and separate joint work in progress with Giulia Iezzi; and describe them as affine morphisms of valuated matroids and linear maps of tropical linear spaces.

Calabi-spin

Friday, November 17th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Andrea RIVEZZI
(Università di Milano "Bicocca")
"Universal quantizations and the Drinfeld-Yetter algebra"

Abstract: In a renowned series of papers, Etingof and Kazhdan proved that every Lie bialgebra can be quantized, answering positively a question posed by Drinfeld in 1992. The quantization is explicit and "universal", that is it is natural with respect to morphisms of Lie bialgebras. A cohomological construction of universal quantizations has been later obtained by Enriquez, relying on the coHochschild complex of a somewhat mysterious cosimplicial algebra. In this talk, I will review the realization of Enriquez' algebra in terms of "universal endomorphisms" of a Drinfeld-Yetter module over a Lie bialgebra, due to Appel and Toledano Laredo, and present a novel combinatorial description of its algebra structure. This is a joint work with A. Appel.

ball-Moebius

Friday, November 17th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Francesca PRATALI
(Université Sorbonne - Paris Nord)
"A tree-like approach to linear infinity operads"

Abstract: Arisen in Algebraic Topology to model the up-to-homotopy associative algebra structure of loop spaces, operads can be thought of as collections of *spaces* of n-ary operations together with composition laws between them. We talk about oo-operads when these operations can be composed only 'up-to-homotopy'. When the n-ary operations organise into actual topological spaces/simplicial sets, several equivalent models for the homotopy theory of oo-operads have been developed. Of our interest is Weiss and Moerdijk's approach, where a certain category of trees replaces the simplex category, and oo-categorical methods are generalized to the operadic context. However, while the theory is well developed in the topological case, very little is known for what it concerns oo-operads enriched in chain complexes ('linear'). In this talk, we explain how the tree-like approach can be applied to the linear case. We discuss the combinatorics of trees and a Segal-like condition which allows to define linear oo-operads as certain coalgebras over a comonad. Then, by considering a category of 'trees with partitions', we realize linear oo-operads as a full subcategory of a functor category.

Calabi-spin

Escher-birds

Colloquium di Dipartimento
Friday, November 3rd, 2023
h. 16:00 - Room "Roberta Dal Passo"
Victor REINER
(University of Minnesota)

"Combinatorics of configuration spaces - recent progress"

N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006
Abstract: The topology of the space of n distinct labeled points in Euclidean space has a long history. Its cohomology is fairly well understood, including as a representation of the symmetric group permuting the n labels. These representations also have mysterious connections with combinatorial notions like descents of permutations, and sometimes "hidden" actions of the symmetric group on n+1 points. We will discuss several results in recent years elucidating some of these connections, including work by and with Marcelo Aguiar, Ayah Almousa, Sarah Brauner, Nick Early, and Sheila Sundaram.

ball-Moebius

Friday, October 20th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Misha FEIGIN
(University of Glasgow)
"Quasi-invariants and free multi-arrangements"

Abstract: Quasi-invariants are special polynomials associated with a finite reflection group W and a multiplicity function. They appeared in 1990 in the study of Calogero-Moser integrable systems by Chalykh and Veselov, in which case they are the highest symbols of differential operators which form a large commutative ring. Similarly to all the polynomials, quasi-invariants form a free module over invariant polynomials of rank |W|, and they have other good properties. Quasi-invariants form representations of spherical Cherednik algebras as was established by Berest, Etingof and Ginzburg in 2003, which gives a way to establish the freeness property. I am going to explain a more recent application of quasi-invariants to the theory of free multi-arrangements of hyperplanes. In this case one is interested in the module of logarithmic vector fields which is known to be free over polynomials for some arrangements including Coxeter ones. Quasi-invariants can be used to construct elements of this module, and they also lead to new free multi-arrangements in the case of complex reflection groups.
The talk is based on a joint work with T. Abe, N. Enomoto and M. Yoshinaga.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project Mat-Mod@TOV (CUP E83C23000330006)

Calabi-spin

Friday, October 20th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Luca FRANCONE
(Université "Claude Bernard" Lyon 1)
"Minimal monomial lifting of cluster algebras and branching problems"

Abstract: We will talk about minimal monomial lifting of cluster algebras. That is sort of a homogenisation technique, whose goal is to identify a cluster algebra structure on some schemes "suitable for lifting", compatibly with a base cluster algebra structure on a given subscheme. We will see how to apply this technique to study some branching problems, in representation theory of complex reductive groups and, time permitting, we will discuss some possible development as the construction of polyhedral models for multiplicities.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project Mat-Mod@TOV (CUP E83C23000330006)

ball-Moebius

Friday, October 6th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Francesco D'ANDREA
(Università di Napoli "Federico II")
"Operator Algebras That One Can See"

Abstract: Many well-known examples of "noncommutative spaces", like Woronowicz' quantum SU(2) or Vaksman-Soibelman odd-dimensional quantum spheres, can be described by C*-algebras associated to directed graphs. More generally, many compact quantum groups and quantum homogeneous spaces, can be described by convolution C*-algebras of "nice" groupoids. C*-algebras associated to combinatorial data (graphs, diagrams, groupoids) allow efficient models to attack key open problems in noncommutative geometry.
The aim of this talk is to present some basic ideas of noncommutative geometry, using graph and groupoid C*-algebras as examples of "noncommutative spaces that one can see".

Calabi-spin

Friday, October 6th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Ryan AZIZ
(Université Libre de Bruxelles)
"Generalized Yetter-Drinfeld modules and the center construction of bimodule categories"

Abstract: A Yetter-Drinfeld module (=YD-module) over a bialgebra H is at the same time module and comodule over H satisfying a compatibility condition. It is well-known that the category of YD-modules (over a finite-dimensional Hopf algebra H) is equivalent to the center of the monoidal category of H-(co)modules as well as the category of modules over Drinfeld doubles of H. Canaepeel, Militaru, and Zhu introduced generalized YD-modules. More precisely, they consider two bialgebras H, K, together with a bicomodule algebra C and bimodule coalgebra over them. A generalized YD-module in their sense, is simultaneously an A-module and a C-comodule with a compatibility condition. Under a finiteness condition, they showed that these modules are exactly modules over a suitable constructed smash product build out of A and C. The aim of this talk is to show how the category of the generalized YD-modules can be obtained as a relative center of the category A-modules, viewed as a bimodule category over categories of H-modules and K-modules. Moreover, we also show how other variations of YD-modules, such as anti-YD-modules, arise as a particular case.
This talk is based on ongoing work with Joost Vercruysse.

ball-Moebius

Friday, June 9th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Alessandro ZAMPINI
(Università di Napoli "Federico II")
"Derivation based differential calculus for a class of noncommutative spaces"

Abstract: After a general introduction on differential calculi on noncommutative spaces, we equip a family of algebras whose noncommutativity is of Lie type with a derivation based differential calculus obtained, upon suitably using both inner and outer derivations, as a reduction of a redundant calculus over the Moyal four dimensional space.

Calabi-spin

Friday, June 9th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Rosanna LAKING
(Università di Verona)
"Cosilting complexes and asymptotic triangulations of the annulus"

Abstract: In 2014 Baur and Dupont introduced the notion of an asymptotic triangulation. This is a combinatorial object arising naturally from the combinatorics of cluster algebras of type Ã. They showed that the set of all asymptotic triangulations has an interesting combinatorial structure: it is a poset and the edges of the Hasse graph can be obtained by flipping the asymptotic arcs. In this talk I will explain how this set parametrises certain 2-term complexes in derived category of a finite-dimensional algebra called a cluster-tilted algebra of type Ã and that the flip operation corresponds to a mutation operation.
This is joint work with L. Angeleri Hügel, K. Baur and F. Sentieri.

ball-Moebius

Friday, May 26th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Gleb NENASHEV
(Brandeis University)
"Schubert Calculus and bosonic operators"

Abstract: In this talk I will present a new point of view on Schubert polynomials via bosonic operators. In particular, we extend the definition of bosonic operators from the case of Schur polynomials to Schubert polynomials. More precisely, we work with back-stable Schubert polynomials and our operators act on the left weak Bruhat order. Furthermore, these operators with an extra condition give sufficiently enough linear equations for the structure of the cohomology ring of flag varieties.

Calabi-spin

Friday, May 26th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Arun RAM
(University of Melbourne)
"Boson-Fermion correspondence for Macdonald polynomials"

Abstract: In its simplest form, this correspondence is the map from symmetric functions to skew-symmetric functions given by multiplication by the Weyl denominator (the Vandermonde determinant). A generalization produces the motivating shadow of "geometric Satake", a diagram which contains the Satake isomorphism, the center of the affine Hecke algebra and the Casselman-Shalika formula. In a miracle that I wish I understood better, the whole diagram generalizes to the case of Macdonald polynomials and sends the bosonic Macdonald polynomial to the fermionic Macdonald polynomial. Does this suggest an "elliptic version" of geometric Langlands?
This talk is based upon arXiv2212.03312, joint with Laura Colmenarejo.

ball-Moebius

Friday, May 12th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Emanuele LATINI
(Università di Bologna)
"Higher conformal Yang-Mills equation"

Abstract: On a pseudo Riemannian manifold consider a 2-form taking value in the adjoint representation of some (semisimple) Lie algebra. It is well known that the corresponding Yang-Mills functional is conformally invariant just in four dimensions. A natural question is whether there are natural replacements of the Yang-Mills functional that are conformally invariant.
In the first part of the talk we will describe the main tools needed to answer this question, namely conformal defining densities for conformally compact manifolds and the (adjoint) tractor bundle; then we will show how to set up and to formally solve the Yang-Mills boundary problem on conformally compact manifolds. In general, smooth solutions are obstructed by an invariant of boundary connections. Specializing to Poincaré-Einstein manifolds with even boundary dimension parity, this obstruction is a conformal invariant of boundary Yang-Mills connections. This yields conformally invariant, higher order generalizations of the Yang-Mills equations and their corresponding energy functionals.

Calabi-spin

Friday, May 12th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Thomas WEBER
(Università di Torino)
"Noncommutative differential geometry with Hopf algebra symmetry"

Abstract: While in classical differential geometry one is given a unique differential structure, the de Rham calculus, such a canonical choice does not exist in noncommutative geometry. Moreover, while the de Rham differential is equivariant with respect to a given Lie group action, a noncommutative calculus might not be compatible with a corresponding Hopf algebra symmetry.
We give a gentle introduction to noncommutative differential geometry, reviewing seminal work of Woronowicz (covariant calculi on Hopf algebras) and Hermisson (covariant calculi on quantum homogeneous spaces). The latter invokes the notion of faithful flatness and Takeuchi/Schneider equivalence. Afterwards we discuss an original construction of a canonical equivariant calculus for algebras in symmetric monoidal categories, with main examples including algebras with (co)triangular Hopf algebra symmetry, particularly Drinfel'd twisted (star product) algebras. The approach relies on and is essentially dual to the concept of 'braided derivations' and we show that the corresponding braided Gerstenhaber algebra of multi-vector fields combines with the noncommutative calculus, forming a braided Cartan calculus. If time permits we illustrate how to formulate Riemannian geometry in this framework, proving that for every equivariant braided metric there is a unique quantum Levi-Civita connection. The second half of the talk is based on the thesis of the speaker.

ball-Moebius

Friday, April 28th, 2023
h. 16:30 - Aula Magna "P. Gismondi"
“Roberto Petronzio Lecture 2023”

Giorgio PARISI
(Accademia dei Lincei & "Sapienza" Università di Roma)
"Multiple Equilibria"

Friday, April 28th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Ernesto SPINELLI
("Sapienza" Università di Roma)
"Hartley's Conjecture and development arising"

Abstract: In the 80s Bryan Hartley conjectured that if the unit group a torsion group algebra FG satisfies a group identity, then FG satisfies a polynomial identity. In this talk we aim to review the most relevant results that arose from its solution and to discuss some recent developments concerning group identities for the set of symmetric units of FG.

ball-Moebius

Friday, April 14th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Filippo VIVIANI
(Università di Roma "Tor Vergata")
"On the Picard group of the stack of G-bundles on families of curves"

Abstract: Given a family of smooth projective curves and an arbitrary connected linear algebraic group G, we investigate the Picard group of the stack of relative G-bundles on the family.
This is a joint work with Roberto Fringuelli.

Calabi-spin

Friday, April 14th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Ilaria DAMIANI
(Università di Roma "Tor Vergata")
"Quantum affine algebras: comparing two coproducts"

Abstract: The quantum affine algebras U_q are Hopf algebras with the coproduct Δ defined by Drinfeld and Jimbo; but they have also a "coproduct" Δ_v with values in a completion of U_q ⊗ U_q , introduced by Drinfeld for quantm affinizations. While the relation between Δ and the action of the braid group (and also of the weight lattice, which is a subgroup of the braid group) is complicated and involves the R-matrix, Δ_v is by construction equivariant with respect to the action of the weight lattice.
In this talk I will show that Δ_v can be obtained as "equivariant limit" of Δ .

ball-Moebius

Friday, March 31st, 2023
h. 16:00 - Room "Roberta Dal Passo"

Elena PASCUCCI
("Sapienza" Università di Roma)
"Fundamental Superalgebras in PI Theory"

Abstract: Fundamental superalgebras are special finite-dimensional superalgebras over an alge-braically closed field of characteristic zero defined in terms of certain multialternating graded polynomials. They play a key role in Kemer's Representability Theorem. In the present talk we provide new examples of fundamental superalgebras. Finally, if time allows, we shall give a characterization of fundamental superalgebras in terms of the representation theory of the hyperoctahedral group.
This is based on a joint work with Antonio Giambruno and Ernesto Spinelli.

Calabi-spin

Friday, March 31st, 2023
h. 14:30 - Room "Roberta Dal Passo"

Giulia IEZZI
(Università di Roma "Tor Vergata" & RWTH Aachen University)
"A realisation of some Schubert varieties as quiver Grassmannians"

Abstract: Quiver Grassmannians are projective varieties parametrising subrepresentations of quiver representations. Their geometry is an interesting object of study, due to the fact that many geometric properties can be studied via the representation theory of quivers. For instance, this method was used to study linear degenerations of flag varieties, obtaining characterizations of flatness, irreducibility and normality via rank tuples.
We give a construction for smooth quiver Grassmannians of a specific wild quiver, realising a class of Schubert varieties inside flag varieties. This allows for a definition of linear degenerations of Schubert varieties.

ball-Moebius

Friday, March 17th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Paolo SARACCO
(Université Libre de Bruxelles)
"Glimpses from truss theory"

Abstract: Trusses are like affine rings: as a ring is an abelian group with a compatible multiplica-tion, a truss is a torsor (over an abelian group) with a compatible multiplication. Introduced by Brzeziński in 2017 to unify the classical theory of rings with the modern theory of braces, trusses unexpectedly revealed the existence of a previously uncharted territory, whose exploration is not just leading to fascinating discoveries, but it is also shedding new light on groups and rings themselves. This talk would like to be a brief walk in the world of ternary operations to meet heaps, trusses, their modules and their novel heaps of modules.

Calabi-spin

Friday, March 17th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Antonio IOPPOLO
(Università de L'Aquila)
"A taste of polynomial identities"

Abstract: A polynomial identity of an algebra A is a polynomial (in non-commuting variables) vanishing under all evaluations in A. Algebras satisfying at least one of these non-trivial relations are called PI-algebras. The first goal of this talk is to show how it is possible to characterize PI-algebras by means of numerical invariants related to their identities. Along the way we will highlight the combinatorial and analytical aspects of the theory, its connection with invariant theory, representation theory and growth problems. In the last part, we will look at some of the latest developments in this area, when it comes to algebras with additional structure.

ball-Moebius

Friday, March 10th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Evgeny FEIGIN
(HSE [Mosca] & Weizmann Institute of Science [Israel])
"Cauchy identities and representation theory"

Abstract: The celebrated Cauchy identity rewrites a certain infinite product as a sum of products of Schur functions. The identity has a vast number of applications and interpretations; in particular, one can understand the infinite product as the character of polynomial functions on the space of square matrices and the products of Schur functions as characters of tensor products of irreducible gl(n) modules.
The classical Cauchy identity has (at least) three natural generalizations: a nonsymmetric version, a q-version and their mixture. The representation theory of the nonsymmetric Cauchy is governed by the Borel subalgebra, the q-Cauchy identity is controlled by the representations of the current algebras and the nonsymmetric q-Cauchy identity has to do with the modules over the Iwahori algebra. We will discuss all these identities and explain the relevant representation theory.
Based on joint works with Anton Khoroshkin, Ievgen Makedonskyi and Daniel Orr.

Calabi-spin

Friday, March 10th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Alexander PÜTZ
(Ruhr-Universität - Bochum)
"Desingularizations of Quiver Grassmannians
for the Equioriented Cycle Quiver"

Abstract: Quiver Grassmannians are projective varieties parametrizing subrepresentations of quiver representations. Originating in the geometric study of quiver representations and in cluster algebra theory, they have been applied extensively in recent years in a Lie-theoretic context, namely as a fruitful source for degenerations of (affine) flag varieties. This approach allows for an application of homological methods from the representation theory of quivers to the study of such degenerate structures. The resulting varieties being typically singular, a construction of natural desingularizations is very desirable.
We construct torus equivariant desingularizations of quiver Grassmannians for arbitrary nilpotent representations of an equioriented cycle quiver. This applies to the computation of their torus equivariant cohomology.

ball-Moebius

Friday, March 3rd, 2023
h. 14:30 - Room "Roberta Dal Passo"

Kirill ZAYNULLIN (University of Ottawa)
"Oriented cohomology of a linear algebraic group
vs. localization in 2-monoidal categories"

Abstract: The Chow ring CH(G) of a split semi-simple linear algebraic group G is one of the key geometric invariants in the theory of linear algebraic groups, torsors, motives of twisted flag varieties. Starting from pioneering works by Grothendieck and Borel, it has been studied for decades and computed for all simple groups (see e.g. Kac 1985, Duan 2015's). In the present talk we explain how to describe (and, hence, to compute) an oriented cohomology (Borel-Moore homology) functor A(G) using the localization techniques of Kostant-Kumar and the techniques of 2-monoidal categories: we show that the natural Hopf-algebra structure on A(G) can be lifted to a 'bi-Hopf' structure on the T-equivariant cohomology A_T(G/B) of the complete flag variety. More generally, we prove that the structure algebra of a Bruhat moment graph of a root system is a Hopf algebroid with respect to the right Hecke and left Brion-Knutson-Tymoczko actions. As an application, we obtain an effective combinatorial way to compute the coproduct on A(G).
This is a joint work with Martina Lanini and Rui Xiong.

Calabi-spin

Friday, February 10th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Mario MARIETTI (Università Politecnica delle Marche)
"Towards Combinatorial Invariance: Kahdan-Lusztig R-polynomials"

Abstract: Kazhdan-Lusztig (KL) polynomials play a central role in several areas of mathematics. In the 80's, Dyer and Lusztig, independently, formulated the Combinatorial Invariance Conjecture (CIC), which states that the KL polynomial associated with two elements u and v only depends on the poset of elements between u and v in Bruhat order. With the help of certain machine learning models, recently Blundell, Buesing, Davies, Velickovic, and Williamson discovered a formula for the KL polynomials of a Coxeter group W of type A, and stated a conjecture that implies the CIC for W (see [Towards combinatorial invariance for Kazhdan-Lusztig polynomials, Representation Theory (2022)] and [Advancing mathematics by guiding human intuition with AI, Nature 600 (2021)]. In this talk, I will present a formula and a conjecture about R-polynomials of W. The advantage in considering R-polynomials rather than KL polynomials is that the corresponding formula and conjecture are less intricate and have a dual counterpart. Our conjecture also implies the CIC.
This is based on joint work with F. Brenti.

ball-Moebius

Friday, February 10th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Maxim GUREVICH (Technion - Israel Institute of Technology)
"Positive decompositions for Kazhdan-Lusztig polynomials"

Abstract: A new algorithmic approach for computation of S_n Kazhdan-Lusztig polynomials, through their restriction to lower rank Bruhat intervals, was recently presented by Geordie Williamson and DeepMind collaborators.
In a joint work with Chuijia Wang we fit this hypercube decomposition into a general framework of a parabolic recursion for Weyl group Kazhdan-Lusztig polynomials. We also show how the positivity phenomena of Dyer-Lehrer and Grojnowski-Haiman come into play in such decompositions.
Staying in type A, I will explain how the new approach naturally manifests through the KLR categorification of (dual) PBW and canonical bases.

Calabi-spin

Friday, February 3rd, 2023
h. 16:00 - Room "Roberta Dal Passo"

Francesco ESPOSITO (Università di Padova)
"Cohomology of quiver Grassmannians and Motzkin combinatorics"

Abstract: Quiver Grassmannians are projective algebraic varieties generalizing ordinary Grass-mannians and flag varieties. The cohomology of quiver Grassmannians of particular type has appli-cations to the geometric interpretation of various algebraic objects such as quantized universal enveloping algebras and cluster algebras. The variation in the cohomology of families of quiver Grassmannians of equioriented type A has been studied by Lanini-Strickland and Fang-Reineke.
In this talk, I relate on joint work with Cerulli Irelli-Fang-Fourier and Cerulli Irelli-Marietti, in which we prove an upper semicontinuity statement for the cohomology of quiver Grassmannians of type A and we study the relation with Motzkin combinatorics found in work of Fang-Reineke.

ball-Moebius

Friday, February 3rd, 2023
h. 14:30 - Room "Roberta Dal Passo"

Lorenzo VECCHI (Università di Bologna)
"Categorical valuative invariants of matroids"

Abstract: Matroids are combinatorial objects that abstract the notion of linear independence and can be used to describe several structures such as, for example, vector spaces and graphs. Informa-tion on matroids can be encoded in several polynomial invariants, the most famous one being the characteristic polynomial; some of these polynomials can also be upgraded to graded vector spaces via abelian categorification or, when the matroid has a non-trivial group of symmetries, to graded virtual representations.
Moreover, to each matroid, one can associate a polytope that belongs to the more general class of generalized permutahedra; a matroid invariant is called valuative if it behaves well under subdivi-sions of matroid polytopes.
After introducing matroids and their invariants, the goal of the talk is to formulate the new notion of categorical valuativity and give some examples.
This is based on a joint ongoing project with Dane Miyata and Nicholas Proudfoot.

rotating-knot

Friday, November 25th, 2022
h. 16:00 - Room "Roberta Dal Passo"

Alessandro IRACI (Università di Pisa)
"Delta and Theta operators expansions"

Abstract: Delta and Theta operators are two families of operators on symmetric functions that show remarkable combinatorial properties. Delta operators generalise the famous nabla operator by Bergeron and Garsia, and have been used to state the Delta conjecture, an extension of the famous shuffle theorem proved by Carlsson and Mellit. Theta operators have been introduced in order to state a compositional version of the Delta conjecture, with the idea, later proved successful, that this would have led to a proof via the Carlsson-Mellit Dyck path algebra. We are going to give an explicit expansion of certain instances of Delta and Theta operators when t=1 in terms of what we call gamma Dyck paths, generalising several results including the Delta conjecture itself, using interesting combinatorial properties of the forgotten basis of the symmetric functions.

Calabi-spin

Friday, November 25th, 2022
h. 14:30 - Room "Roberta Dal Passo"

Giovanni GAIFFI (Università di Pisa)
"Combinatorial aspects of the cohomology of compactifications of toric arrangements"

Abstract: I will describe how to construct monomial bases for the integer cohomology rings of compact wonderful models of toric arrangements. In the description of the monomials various combinatorial objects come into play: building sets, nested sets, and the fan of a suitable toric variety. In particular, I will focus on the case of the toric arrangements associated with root systems of type A. Here the combinatorial description of these basis offers a geometrical point of view on the relation between some eulerian statistics on the symmetric group.
This is a joint work with Oscar Papini and Viola Siconolfi.

ball-Moebius

Friday, November 11th, 2022
h. 16:00 - Room "Roberta Dal Passo"

Sabino DI TRANI (Università di Trento)
"Smoothness Criteria for T-Fixed Points in Flat Linear Degenerations of the Flag Variety"

Abstract: Linear Degenerations of the Flag Variety arise as very natural generalizations of the Complete Flag Variety and their geometrical properties very often appear to be linked with interesting combinatorial patterns.
The talk will focus on a special class of linear degenerations, the Flat Degenerations, that have the remarkable property of being equidimensional algebraic varieties of the same dimension as the Complete Flag Variety. In some very recent works of M. Lanini and A. Pütz it is proved that Linear Degenerations of the Flag Variety can be endowed with a structure of GKM variety, under the action of a suitable algebraic torus T.
The aim of the talk is to show how GKM Theory can be applied in this setting to prove some new results about the smooth locus in Flat Degenerations, generalizing a smoothness criterion proved by G. Cerulli Irelli, E. Feigin and M. Reineke for Feigin Degeneration.
Finally, we provide a different combinatorial criterion, linking the smoothness property of a T-fixed point to the complete graph and to its orientations.

Calabi-spin

Friday, November 11th, 2022
h. 14:30 - Room "Roberta Dal Passo"

Azzurra CILIBERTI ("Sapienza" Università di Roma)
"Categorification of skew-symmetrizable cluster algebras through symmetric quivers"

Abstract: I will present my attempt to categorify cluster algebras of type B and C using the theory of symmetric quivers in the sense of Derksen and Weyman.

ball-Moebius

Friday, October 28th, 2022
h. 16:00 - Room "Roberta Dal Passo"

Salvatore STELLA (Università de L'Aquila)
"Dominance order and pointed bases for cluster algebras"

Abstract: Cluster algebras are a class of commutative rings endowed with a partial canonical basis whose elements are called cluster monomials. They are defined recursively through the combinatorial machinery of seeds and mutations. Cluster monomials have a particularly nice property: they are pointed, i.e. they can be written as the product of a Laurent monomial with a monic polynomial with respect to any seed.
One of the main problems in the theory has been to extend the set of cluster monomials to a full basis consisting only of pointed elements. This has been achieved in a variety of generalities using approaches deriving, for example, from representation of associative algebras, Teichmüller theory, and mirror symmetry. Recently Qin introduced a dominance order on the tropical points of the associated cluster variety and showed that this order can be used to parametrize all possible pointed bases.
In this talk we will explicitly describe the dominance order in rank two using a simple geometric construction. We will then connect it to certain representations of SL₃ . Time permitting we will conclude discussing how this construction generalizes to higher rank.

Calabi-spin

Friday, October 28th, 2022
h. 14:30 - Room "Roberta Dal Passo"

Carolina VALLEJO RODRIGUEZ (Università di Firenze)
"On character conductors"

Abstract: Given a character χ of a finite group G, there is a minimal positive integer f_χ such that all the values of χ belong to the f_χ-th cyclotomic field over the rationals. This number is often referred to as the conductor of χ. I will discuss some features of irreducible character conductors and their behavior with respect to factor groups.

ball-Moebius

Friday, October 14th, 2022
h. 16:00 - Room "Roberta Dal Passo"

Boris KRUGLIKOV (UiT / University of Tromsø)
"Symmetries of supergeometries
related to nonholonomic superdistributions"

Abstract: We extend the Tanaka theory to the context of supergeometry and obtain an upper bound on the supersymmetry dimension of geometric structures related to strongly regular bracket-generating distributions on supermanifolds and their structure reductions. Several examples will be demonstrated, including distributions with at most simple Lie superalgebras as maximum symmetry.
The talk is based on joint works with Andrea Santi, Dennis The and Andreu Llabres.

Calabi-spin

Friday, October 14th, 2022
h. 14:30 - Room "Roberta Dal Passo"

Guido PEZZINI ("Sapienza" Università di Roma)
"Moment polytopes of spherical varieties and
applications to multiplicity-free Hamiltonian manifolds"

Abstract: Spherical varieties are a generalization of toric, symmetric and flag varieties. They are also relevant in symplectic geometry, in particular in relation with multiplicity-free Hamiltonian manifolds. In this talk we will describe how the combinatorial structures arising in this theory can be used to characterize those multiplicity-free manifolds that admit a Kähler structure, in terms of their moment polytopes.
This is a joint work with Bart Van Steirteghem.

ball-Moebius

Friday, September 30th, 2022
h. 16:00 - Room "Roberta Dal Passo"

Apoorva KHARE (Indian Institute of Science)
"Higher-order theory for highest weight modules: positive weight-formulas,
resolutions and characters for higher order Verma modules"

Abstract: We introduce higher order Verma modules over a Kac-Moody algebra g (one may assume this to be sl_n throughout the talk, without sacrificing novelty). Using these, we present positive formulas - without cancellations - for the weights of arbitrary highest weight g-modules V. The key ingredient is that of "higher order holes" in the weights, which we introduce and explain.

Calabi-spin

Friday, September 30th, 2022
h. 14:30 - Room "Roberta Dal Passo"

Daniele VALERI ("Sapienza" Università di Roma)
"Integrable triples in simple Lie algebras"

Abstract: We define integrable triples in simple Lie algebras and classify them, up to equivalence. The classification is used to show that all (but few exceptions) classical affine W-algebras W(g,f ), where g is a simple Lie algebra and f a nilpotent element, admit an integrable hierarchy of bi-Hamiltonian PDEs. This integrable hierarchy generalizes the Drinfeld-Sokolov hierarchy which is obtained when f is the sum of negative simple root vectors.

ball-Moebius

Friday, May 27th, 2022
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Francesco BRENTI (Università di Roma "Tor Vergata")
"Graphs, stable permutations, and Cuntz algebra automorphisms"

Abstract: Stable permutations are a class of permutations that arises in the study of the automor-phism group of the Cuntz algebra. In this talk, after introducing the Cuntz algebra and surveying the main known results about stable permutations, I will present a characterization of stable permu-tations in terms of certain associated graphs. As a consequence of this characterization we prove a conjecture in [Advances in Math. 381 (2021) 107590], namely that almost all permutations are not stable, and we characterize explicitly stable 4 and 5-cycles.
This is a joint work with Roberto Conti and Gleb Nenashev.

Calabi-spin

Friday, May 20th, 2022
h. 16:00 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Iain GORDON (University of Edinburgh)
"Gaudin algebras, RSK and Calogero-Moser cells in type A"

Abstract: A few years ago, Bonnafé-Rouquier defined 'Calogero-Moser cells' through the representation theory of rational Cherednik algebras. These cells partition the elements of a complex reflection group G, but are currently difficult to calculate except in small rank examples. In the special case when G is a finite Coxeter group, the cells are conjectured to be the same as Kazhdan-Lusztig cells. In other words, conjecturally 'Calogero-Moser cells' generalise Kazhdan-Lusztig cell theory from Coxeter groups to complex reflection groups. I will discuss a confirmation of this conjecture for G being the symmetric group. The proof uses ideas from integrable systems (Gaudin algebras), algebraic geometry (moduli of points on genus zero curves), and combinatorics (crystals).
This is joint work with A.Brochier and N.White.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006

ball-Moebius

Friday, May 20th, 2022
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Fabio GAVARINI (Università di Roma "Tor Vergata")
"Multiparameter quantum groups: a unifying approach"

Abstract: The original quantum groups - in particular, quantized universal enveloping algebras, in short QUEA's - have been introduced as depending on just one "continuous" parameter. Later on, multiparameter quantum groups - in particular, multiparameter QUEA's - have been introduced in differente ways, with the new, "discrete" parameters either affecting the coalgebra structure or the algebra structure (while leaving the dual structure unchanged). Both cases can be realized as special type deformations - namely, either by twist, or by 2-cocycle deformation - of Drinfeld's celebrated QUEA U_h(g). In this talk I will introduce a new, far-reaching family of multiparameter QUEA's that encompasses and generalizes the previous ones, while also being stable with respect to both deformation by twists and deformations by cocycles.
Taking semiclassical limits, these new multiparameter QUEA's give rise to a new family of multiparameter Lie bialgebras, that in turn is stable under both by twist and deformations by 2-cocycles (in the Lie bialgebraic sense).
This is a joint work with Gastón Andrés García - cf. arXiv:2203.11023 (2022).

Calabi-spin

Friday, May 6th, 2022
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Peter FIEBIG (Friedrich-Alexander-Universität Erlangen-Nüürnberg)
"Tilting modules and torsion phenomena"

Abstract: Given a root system and a prime number p we introduce a category X of "graded spaces with Lefschetz operators" over a ring A. Then we show that under a base change morphism from A to a field K this category specialises to representations of the hyperalgebra of a reductive group, if K is a field of positive characteristic, and of a quantum group at p^l-th root of unity, if K is the p^l-th cyclotomic field. In this category we then study torsion phenomena (over the ring A) and construct for any highest weight a family of universal objects with certain torsion vanishing conditions. By varying these conditions, we can interpolate between the Weyl modules (maximal torsion) and the tilting objects (no torsion). This construction might shed some light on the character generations philosophy of Lusztig and Lusztig-Williamson.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006

ball-Moebius

Friday, April 22nd, 2022
h. 16:00 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Andrea APPEL (Università di Parma)
"Schur-Weyl duality for quantum affine symmetric pairs"

Abstract: In the work of Kang, Kashiwara, Kim, and Oh, the Schur-Weyl duality between quantum affine algebras and affine Hecke algebras is extended to certain Khovanov-Lauda-Rouquier (KLR) algebras, whose defining combinatorial datum is given by the poles of the normalised R-matrix on a set of representations.
In this talk, I will review their construction and introduce a "boundary" analogue, consisting of a Schur-Weyl duality between a quantum symmetric pair of affine type and a modified KLR algebra arising from a (framed) quiver with a contravariant involution. With respect to the Kang-Kashiwara-Kim-Oh construction, the extra combinatorial datum we take into account is given by the poles of the normalised K-matrix of the quantum symmetric pair.

Calabi-spin

Friday, April 22nd, 2022
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Lleonard RUBIO y DEGRASSI (Università di Verona)
"Maximal tori in HH¹ and the homotopy theory of bound quivers"

Abstract: Hochschild cohomology is a fascinating invariant of an associative algebra which possesses a rich structure. In particular, the first Hochschild cohomology group HH¹(A) of an algebra A is a Lie algebra, which is a derived invariant and, among selfinjective algebras, an invariant under stable equivalences of Morita type. This establishes a bridge between finite dimensional algebras and Lie algebras, however, aside from few exceptions, fine Lie theoretic properties of HH¹(A) are not often used.
In this talk, I will show some results in this direction. More precisely, I will explain how maximal tori of HH¹(A), together with fundamental groups associated with presentations of A, can be used to deduce information about the shape of the Gabriel quiver of A. In particular, I will show that every maximal torus in HH¹(A) arises as the dual of some fundamental group of A. By combining this, with known invariance results for Hochschild cohomology, I will deduce that (in rough terms) the largest rank of a fundamental group of A is a derived invariant quantity, and among self-injective algebras, an invariant under stable equivalences of Morita type. Time permitting, I will also provide various applications to semimonomial and simply connected algebras.
This is joint work with Benjamin Briggs.

ball-Moebius

Friday, April 8th, 2022
(( beware of the unusual time!!! ))
h. 16:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Paolo PAPI ("Sapienza" Università di Roma)
"Collapsing levels for affine W-algebras"

Abstract: I will discuss some projects in collaboration with D. Adamovic, V. Kac and P. Moseneder-Frajria regarding affine W-algebras. I will concentrate on the notion of collapsing level for not necessarily minimal W-algebras and I will illustrate some applications to the representation theory of affine algebras and, if time allows, to our conjectural classification of unitary representations for minimal W-algebras.

Calabi-spin

Friday, April 8th, 2022
(( beware of the unusual time!!! ))
h. 14:00 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Vincenzo MORINELLI (Università di Roma "Tor Vergata")
"About Lie theory in Algebraic Quantum Field Theory"

Abstract: The relation between the geometric and the algebraic structure in algebraic quantum field theory is an intriguing topic that has been studied through several mathematical areas (Operator algebras, Lie theory, Category theory...). A fundamental concept in Algebraic Quantum Field Theory (AQFT) is the relation between the localization property and the geometry of models. In the recent work with K.-H. Neeb (Univ. Erlangen-Nürnberg) we rephrased and generalized some aspects of this relation by using the language of Lie theory.
We will start the talk introducing fundamental algebraic features of AQFT, in particular the Haag-Kastler axioms and the one particle formalism, and the presenting algebraic construction of the free field due to R.Brunetti, D. Guido and R. Longo. We will explain how this picture can be generalized. Firstly, how to determine some fundamental localization region, called wedge regions, at the Lie theory level and how a general Lie group can support a generalized AQFT. Then we show a classification of the simple Lie algebras supporting abstract wedges in relation with some special wedge configurations. The construction is possible for a large family of Lie groups and provides several new models in a generalized framework. Such a description of AQFT model generalization does not need a supporting manifold even if it is a desirable object. Time permitting, we will comment on recent developments about symmetric manifolds such models.
Based on V. Morinelli and K.-H. Neeb, Covariant homogeneous nets of standard subspaces, Commun. in Math. Phys 386 (1), 305-358 (2021).

ball-Moebius

Friday, March 25th, 2022
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Andrea SANTI (UiT The Arctic University of Norway / Università di Roma "Tor Vergata")
"G(3) supergeometry and a supersymmetric extension of the Hilbert-Cartan equation"

Abstract: I will report on the realization of the simple Lie superalgebra G(3) as symmetry superalgebra of various geometric structures - most importantly super-versions of the Hilbert-Cartan equation and Cartan's involutive system that exhibit G(2) symmetry - and compute, via Spencer cohomology groups, the Tanaka-Weisfeiler prolongation of the negatively graded Lie superalgebras associated with two particular choices of parabolics. I will then discuss non-holonomic superdistributions with growth vector (2|4 , 1|2 , 2|0) obtained as super-deformations of rank 2 distributions in a 5-dimensional space, and show that the second Spencer cohomology group gives a binary quadric, thereby providing a "square-root" of Cartan's classical binary quartic invariant for (2,3,5)-distributions.
This is a joint work with B. Kruglikov and D. The.

Calabi-spin

Friday, March 11th, 2022
h. 16:00 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Rita FIORESI (Università di Bologna)
"Generalized Root Systems"

Abstract: In Lie theory we define root systems in several contexts: Lie algebras, superalgebras, affine algebras, etc. There is even more: Kostant defines a more general notion of root systems, by taking roots with respect to a generic toral subalgebra (i.e. not necessarily maximal). All these notions of root systems do not behave well with respect to quotients: the quotient (or projection) of a root systems is not in general a root system. We present here a more general notion of root system, inspired by Kostant, which accomodates all of the above examples and behaves well with respect to quotients and projections.
We give a classification theorem for rank 2 generalized root system: there are only 14 of them up to combinatorial equivalence, moreover they are all quotients of Lie algebra root systems. We also prove that root systems of contragredient Lie superalgebras are quotients of root systems of Lie algebras, up to combinatorial equivalence.
In the end, we relate our construction with the problem of determining the conjugacy class of two Levi subgroups in a Lie (super)algebra.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006

ball-Moebius

Friday, March 11th, 2022
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Andrea BIANCHI (University of Copenhagen)
"Symmetric groups, Hurwitz spaces and moduli spaces of surfaces"

Abstract: Let d≥2 , and consider the symmetric group S_d . For k≥0 , the classical Hurwitz space Hur_k(d) parametrises d-fold branched covers of the complex plane C with precisely k branching points. We introduce an amalgamation of all Hurwitz spaces, for varying k, into a single space Hur(S_d). The construction relies on the notion of "partially multiplicative quandle", an algebraic structure slightly weaker than the structure of group, and we will see how to consider S_d as a PMQ in a convenient way.
The main motivation to consider the amalgamated Hurwitz space Hur(S_d) is the following. For all g&geq>0 and n≥1 , let M_g,n denote the moduli space of Riemann surfaces of genus g with n ordered and parametrised boundary components. Our main result ensures that if d is large enough (with respect to g and n), then there exists a connected component of Hur(S_d) which is homotopy equivalent to M_g,n .
Moreover, the space Hur(S_d) carries a natural structure of topological monoid, and we will briefly sketch the computation of the stable, rational cohomology of its connected components. The result is very explicit in degrees up to roughly d. Letting d go to infinity, one can in particular recover the Mumford conjecture on the stable, rational cohomology of moduli spaces of Riemann surfaces, originally proved by Madsen and Weiss.

Calabi-spin

Friday, February 25th, 2022
h. 16:00 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Eugenio GIANNELLI (Università di Firenze)
"On Sylow Branching Coefficients"

Abstract: In this talk we will discuss the nature of the relationship between the representations of a finite group G and those of a Sylow subgroup P of G.
We will introduce Sylow Branching Coefficients (SBCs) and we will show how the study of these numbers led us to prove a conjecture proposed by Malle and Navarro in 2012. We will conclude by presenting new results on SBCs in the case where G is the symmetric group.
The talk is based on joint works with Law, Long, Navarro, Vallejo and Volpato.

ball-Moebius

Friday, February 25th, 2022
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Jacopo GANDINI (Università di Bologna)
"Fully commutative elements and spherical nilpotent orbits"

Abstract: Let g be a simple Lie algebra, with a fixed Borel subalgebra b = t+n, and let W be the associated Weyl group. The Steinberg map associates to any element of W a nilpotent orbit in g, which is defined by the corresponding set of inversions. Extending on previous work of Fan and Stembridge, in this talk I will compare two different notions of "smallness", one available in the Weyl group and the other one for nilpotent orbits.

Calabi-spin

Friday, February 11th, 2022
h. 16:00 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Corrado DE CONCINI
(Accademia Nazionale delle Scienze / "Sapienza" Università di Roma)
"Paving Springer fibers"

Abstract: In the paper [De Concini C., Lusztig G., Homology of the zero-set of a nilpotent vector field on a flag manifold, J. Amer. Math. Soc. 1 (1988), no. 1, 15-34] it was proven that the so called Springer fiber B_n for any nilpotent element n in a complex simple Lie algebra g has homological properties that suggest that B_n should have a paving by affine spaces.
This last statement was proved to hold in the case in which g is classical, but remained open for exceptional groups in types E₇ and E₈.
In a joint project with Maffei we are trying to fill the gap. At this point our efforts have been successful in type E₇ and "almost" in type E₈, where one is reduced to show it only in one case.
The goal of the talk is to survey the problem and give an idea on how to show our new results.

ball-Moebius

Friday, February 11th, 2022
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Domenico FIORENZA ("Sapienza" Università di Roma)
"Brackets and products from centres in extension categories"

Abstract: Building on Retakh's approach to Ext groups through categories of extensions, Schwede reobtained the well-known Gerstenhaber algebra structure on Ext groups over bimodules of associative algebras both from splicing extensions (leading to the cup product) and from a suitable loop in the categories of extensions (leading to the Lie bracket). We show how Schwede's construction admits a vast generalisation to general monoidal categories with coefficients of the Ext groups taken in (weak) left and right monoidal (or Drinfel'd) centres. In case of the category of left modules over bialgebroids and coefficients given by commuting pairs of braided (co)commutative (co)monoids in these categorical centres, we provide an explicit description of the algebraic structure obtained this way, and a complete proof that this leads to a Gerstenhaber algebra is then obtained from an operadic approach. This, in particular, considerably generalises the classical construction given by Gerstenhaber himself. Conjecturally, the algebraic structure we describe should produce a Gerstenhaber algebra for an arbitrary monoidal category enriched over abelian groups, but even the bilinearity of the cup product and of the Lie-type bracket defined by the abstract construction in terms of extension categories remain elusive in this general setting.
This is a joint work with Niels Kowalzig, cf. arXiv:2112.11552.

Calabi-spin

Friday, January 28th, 2022
h. 16:00 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

René SCHOOF (Università di Roma "Tor Vergata")
"Class numbers of cyclotomic fields"

Abstract: It is notoriously difficult to compute class numbers of cyclotomic fields.
In this expository lecture we describe an experimental approach to this problem.

ball-Moebius

Friday, January 28th, 2022
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Andrea FERRAGUTI (SNS Pisa)
"Abelian dynamical Galois groups"

Abstract: Dynamical Galois groups are invariants associated to dynamical systems generated by the iteration of a self-rational map of P¹. These are still very mysterious objects, and it is conjectured that abelian groups only appear in very special cases. We will show how the problem is deeply related to a dynamical property of these rational maps (namely that of being post-critically finite) and we will explain how to approach and prove certain non-trivial cases of the conjecture.
This is based on joint works with A. Ostafe, C. Pagano and U. Zannier.

Calabi-spin

Friday, January 14th, 2022
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Jens EBERHARDT (University of Bonn)
"Motivic Springer theory"

Abstract: Algebras and their representations can often be constructed geometrically in terms of convolution of cycles. For example, the Springer correspondence describes how irreducible repre-sentations of a Weyl group can be realised in terms of a convolution action on the vector spaces of irreducible components of Springer fibers. Similar situations yield the affine Hecke algebra, quiver Hecke algebra (KLR algebra), quiver Schur algebra or Soergel bimodules.
In this spirit, we show that these algebras and their representations can be realised in terms of certain equivariant motivic sheaves called Springer motives. On our way, we will discuss weight structures and their applications to motives as well as Koszul and Ringel duality.
This is joint work with Catharina Stroppel.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006

rotating-knot

Friday, December 10th, 2021
h. 16:00 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Riccardo BIAGIOLI (Università di Bologna)
"Temperley-Lieb algebra and fully commutative elements in affine type C"

Abstract: The Temperley-Lieb algebra is a well studied finite dimensional associative algebra: it can be realized as a diagram algebra and it has a basis indexed by the fully commutative elements in the Coxeter group of type A. A few years ago, Dana Ernst introduced an elegant generalization of such diagrammatic representation for the generalized Temperley-Lieb algebra of affine type C. The proof that such representation is faithful is quite involved and the same author wonders if an easier proof exists.
In this talk, we present a new combinatorial way to describe Ernst's algebra homomorphism, from which injectivity and subjectivity follow more easily. Our results are based on a classification of fully commutative elements of affine type C in terms of heaps of pieces, and on certain operations that we define on such heaps.
This talk is based on a joint work with Giuliana Fatabbi and Gabriele Calussi.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006

ball-Moebius

Friday, December 10th, 2021
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Margherita PAOLINI (Università de L'Aquila)
"Integral forms of affine Lie algebras"

Abstract: Let be g a semisimple finite dimensional Lie algebra and U(g) its universal enveloping algebra. The theory of highest weight representations of g may pass through the description of an integral form of U(g), namely a suitable Z-subalgebra of U(g) generated by the divided powers of the Chevalley generators; for this reason it has been studied by several authors (e.g., Chevalley and Cartier).
If ĝ is an affine Lie algebra, in order to extend this approach, the analogous Z-subalgebra has been studied by Garland (in the untwisted case) and by Mitzman and by Fisher-Vasta (in the twisted case). Anyhow, the case when ĝ is of type A_2n² still remains obscure. In order to study the representation theory of this algebra we try to find more manageable techniques that will help to get a deeper understanding.
The aim of this talk is to present the structure of these integral forms and some related results.

Calabi-spin

Friday, November 26th, 2021
h. 16:00 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Niels KOWALZIG (Università di Napoli "Federico II")
"Centres, traces, and cyclic cohomology"

Abstract: In this talk, we will discuss the biclosedness of the monoidal categories of modules and comodules over a (left or right) Hopf algebroid, along with the bimodule category centres of the respective opposite categories and a corresponding categorical equivalence to anti Yetter-Drinfel'd contramodules and anti Yetter-Drinfel'd modules, respectively. This is directly connected to the existence of a trace functor on the monoidal categories of modules and comodules in question, which in turn allows to recover (or define) cyclic operators enabling cyclic cohomology.

ball-Moebius

Friday, November 26th, 2021
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Marco TREVISIOL ("Sapienza" Università di Roma)
"Normality of closure of orthogonal nilpotent symmetric orbits"

Abstract: Kraft and Procesi showed that the Zariski closure of the conjugacy classes of type A are all normal and, in type B, C and D, they have described which ones are normal. In their work the Lie group acts on its Lie algebra by the adjoint action. In types B, C, D, a similar question can be asked for the action of the Lie group on the odd part of the general linear Lie algebra; that is the orthogonal group acting on the symmetric matrices and the symplectic group acting on the symmetric-symplectic matrices. Ohta showed that in the latter case every orbit has normal closures while this conclusion is not valid in the former case. In this talk I will present the main result of my Ph.D. thesis which gives a combinatorial description of the orbit whose closures are normal in the orthogonal case.

Calabi-spin

Friday, November 12th, 2021
h. 16:00 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Viola SICONOLFI (Università di Pisa)
"Zeta-functions for class two nilpotent groups"

Abstract: The notion of Zeta-function for groups was introduced in a seminal paper from Grunewald, Segal and Smith and proved to be a powerful tool to study the subgroup growth in some classes of groups.
In this seminar I will introduce this Zeta-function presenting some general properties for this object. I will then focus on some results obtained for class two nilpotent groups.
I will in particular describe some combinatorial tecniques used to tackle this problem, namely the study of series associated to polyhedral integer cones.
This is a joint work with Christopher Voll and Marlies Vantomme.

ball-Moebius

Friday, November 12th, 2021
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Paolo BRAVI ("Sapienza" Università di Roma)
"On the multiplication of spherical functions of reductive spherical pairs"

Abstract: Let G be a simple complex algebraic group and let K be a reductive subgroup of G such that the coordinate ring of G/K is a multiplicity free G-module. We consider the G-algebra structure of C[G/K], and study the decomposition into irreducible summands of the product of irreducible G-submodules in C[G/K]. We will present a conjectural decomposition rule for some special reductive pairs together with some partial results supporting the conjecture. We will explain how our conjecture would actually follow from an old conjecture of Stanley on the multiplication of Jack symmetric functions. We will also present a few new basic results related to Stanley's conjecture itself. The talk is based on a collaboration with Jacopo Gandini.

Calabi-spin

Friday, October 29th, 2021
h. 16:00 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Michele D'ADDERIO (Université Libre de Bruxelles)
"Partial and global representations of finite groups"

Abstract: The notions of partial actions and partial representations have been extensively studied in several algebraic contexts in the last 25 years. In this talk we introduce these concepts and give a short overview of the results known for finite groups.
We will briefly show how this theory extends naturally the classical global theory, in particular in the important case of the symmetric group.
This is joint work with William Hautekiet, Paolo Saracco and Joost Vercruysse.
N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006

ball-Moebius

Friday, October 29th, 2021
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Chris BOWMAN (University of York)
"Soergel diagrammatics in modular representation theory"

Abstract: We provide an elementary introduction to Elias-Williamson's Soergel diagrammatics and p-Kazhdan-Lusztig theory and discuss the applications in representation theory. In particular we will discuss the recent proof of (generalised versions of) Libedinsky-Patimo's conjecture, which states that certain simple characters of affine Hecke algebras are given in terms of p-Kazhdan-Lusztig polynomials and of Berkesch-Griffeth-Sam's conjecture which states that the unitary representations admit cohomological constructions via BGG resolutions.
This is joint work with Anton Cox, Amit Hazi, Emily Norton, and Jose Simental.

Calabi-spin

Friday, October 15th, 2021
h. 16:00 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Giovanni CERULLI IRELLI ("Sapienza" Università di Roma)
"On degeneration and extensions of symplectic and orthogonal quiver representations "

Abstract: Motivated by linear degenerations of flag varieties, and the study of 2-nilpotent B-orbits for classical groups, I will review the representation theory of symmetric quivers, initiated by Derksen and Weyman in 2002. I will then focus on the problem of describing the orbit closures in this context and how to relate it to the orbit closures for the underlying quivers. In collaboration with M. Boos we have recently given an answer to this problem for symmetric quivers of finite type. I believe that this result is a very special case of a much deeper and general result that I will mention in the form of conjectures and open problems. The talk is based on the preprint version of my paper with Boos available on the arXiv as 2106.08666.

ball-Moebius

Friday, October 15th, 2021
h. 14:30 - Room "Roberta Dal Passo"
click HERE to attend the talk in streaming

Lorenzo GUERRA (Scuola Normale Superiore - Pisa)
"Symmetric groups, tensor powers and extended powers of a topological space"

Abstract: The n-th cohomology of the symmetric group S_n on n objects with coefficients in the n-th tensor power of a vector space V on a field k, is endowed with an extremely rich algebraic structure. Indeed, their direct sum for all n ∈ N is an example of what goes under the name of "Hopf ring".
First I will recall and review the definition of Hopf ring, then I will explicitly describe the cohomology algebras above, and finally I will briefly discuss the link with extended powers and other topological spaces interesting for homotopy theorists.
The content of this talk stems from an ongoing collaboration with Paolo Salvatore and Dev Sinha.

Calabi-spin

Online Representation Theory Seminar

Friday, June 25th, 2021 - h. 15:00
click HERE to attend the talk in streaming

Oleksandr TSYMBALIUK (Purdue University - USA)
"Shifted Yangians and quantum affine algebras revisited"

Abstract: In the first part of the talk, I will recall some basic results about shifted Yangians (and their trigonometric versions-the shifted quantum affine algebras), which first appeared in the work of Brundan-Kleshchev relating type A Yangians and finite W-algebras and have become a subject of renewed interest over the last five years due to their close relation to quantized Coulomb branches introduced by Braverman-Finkelberg-Nakajima.
In the second part of the talk, I will try to convince that the case of antidominant shifts (opposite to what was originally studied in the work of Brundan-Kleshchev in type A and of Kamnitzer-Webster-Weekes-Yacobi in general type) is of particular importance as the corresponding algebras admit the RTT realization (at least in the classical types).
In particular, this provides a conceptual explanation of the coproduct homomorphisms, gives rise to the integral forms of shifted quantum affine algebras, and also yields a family of (conjecturally) integrable systems on the corresponding Coulomb branches. As another application, the GKLO-type homomorphisms used to define truncated version of the above algebras provide a wide class of rational/trigonometric Lax matrices in classical types.
This talk is based on the joint works with Michael Finkelberg as well as Rouven Frassek and Vasily Pestun.

ball-Moebius

Online Representation Theory Seminar

Friday, June 18th, 2021 - h. 15:00
click HERE to attend the talk in streaming

Lara BOSSINGER (UNAM Oaxaca - Mexico)
"Newton-Okounkov bodies for cluster varieties"

Abstract: Cluster varieties are schemes glued from algebraic tori. Just as tori themselves, they come in dual pairs and it is good to think of them as generalizing tori. Just as compactifications of tori give rise to interesting varieties, (partial) compactifications of cluster varieties include examples such as Grassmannians, partial flag varieties or configurations spaces. A few years ago Gross-Hacking-Keel-Kontsevich developed a mirror symmetry inspired program for cluster varieties. I will explain how their tools can be used to obtain valuations and Newton-Okounkov bodies for their (partial) compactifications. The rich structure of cluster varieties however can be exploited even further in this context which leads us to an intrinsic definition of a Newton-Okounkov body.
The theory of cluster varieties interacts beautifully with representation theory and algebraic groups. I will exhibit this connection by comparing GHKK's technology with known mirror symmetry constructions such as those by Givental, Baytev-Ciocan-Fontanini-Kim-van Straten, Rietsch and Marsh-Rietsch (joint work in progress with M. Cheung, T. Magee and A. Nájera Chávez).

Calabi-spin

Online Representation Theory Seminar

Friday, June 11th, 2021 - h. 15:00
click HERE to attend the talk in streaming

Lucien HENNECART (Université de Paris-Saclay - France)
"Perverse sheaves with nilpotent singular support for curves and quivers"

Abstract: Perverse sheaves on the representation stacks of quivers are fundamental in the categorification of quantum groups. I will explain how to prove that semisimple perverse sheaves with nilpotent singular support on the stack of representations of an affine quiver form Lusztig category and how to extend this question to quivers with loops. The analogous question for curves is to determine perverse sheaves on the stack of coherent sheaves whose singular support is a union of irreducible components of the global nilpotent cone. We solve this problem for elliptic curves, for which we also show that the characteristic cycle map induces a bijection between simple Eisenstein spherical perverse sheaves and irreducible components of the global nilpotent cone. This constitutes a step towards the understanding of the degree zero part of the cohomological Hall algebra of a curve.

ball-Moebius

Online Representation Theory Seminar

Friday, June 4th, 2021 - h. 15:00
click HERE to attend the talk in streaming

Abel LACABANNE (UC Louvain - Belgium)
"An asymptotic cellular category for G(e,e,n)"

Abstract: Given a Coxeter group W, one may consider its Hecke algebra, which is a deformation of the group algebra of W. Kazhdan and Lusztig have constructed the celebrated Kazhdan-Lusztig basis, which has many interesting properties. This basis can be used to construct a partition of W into Kazhdan-Lusztig cells, a partition of the irreducible complex representations of W into families and also a partition of the "unipotent characters" of W into families. There exist categorical counterparts of these objects, and the goal of this talk is to explain a tentative towards a partial generalization for the complex reflection group G(e,e,n).
First, I will describe the situation of a Coxeter group and then explain briefly what can be extended to (some) complex reflection groups. Finally, I will turn to an description of the asymptotic category, which is constructed from representations of quantum sln at a 2e-th root of unity, and try to justify the term "asymptotic cellular category".

Calabi-spin

Online Representation Theory Seminar

Friday, May 28th, 2021 - h. 15:00
Room "Roberta Dal Passo" (20 seats available)
click HERE to attend the talk in streaming

Alessio CIPRIANI (Università di Roma "Tor Vergata" - Italy)
"Perverse Sheaves, Finite Dimensional Algebras and Quivers"

Abstract: In this talk I will introduce the category of perverse sheaves on a topologically stratified space X and give some examples. Then, I will show that when X has finitely many strata, each with finite fundamental group, such category is equivalent to a category of modules over a finite dimensional algebra A. Finally, I will discuss some algebraic approaches one can use in order to describe the algebra A.
This talk is based on joint work with Jon Woolf.

ball-Moebius

Online Representation Theory Seminar

Friday, May 21st, 2021 - h. 15:00
click HERE to attend the talk in streaming

Sachin GAUTAM (Ohio State University - USA)
"R-matrices and Yangians"

Abstract: An R-matrix is a solution to the Yang-Baxter equation (YBE), a central object in Statistical Mechanics, discovered in 1970's. The R-matrix also features prominently in the theory of quantum groups formulated in the eighties. In recent years, many areas of mathematics and physics have found methods to construct R-matrices and solve the associated integrable system.
In this talk I will present one such method, which produces meromorphic solutions to (YBE) starting from the representation theory of a family of quantum groups called Yangians. Our techniques give (i) a constructive proof of the existence of the universal R-matrix of Yangians, which was obtained via cohomological methods by Drinfeld in 1983, and (ii) prove that Drinfeld's universal R-matrix is analytically well behaved.
This talk is based on joint works with Valerio Toledano Laredo and Curtis Wendlandt.

Calabi-spin

Online Representation Theory Seminar

Friday, May 7th, 2021 - h. 15:00
click HERE to attend the talk in streaming

Thorge JENSEN (free lance)
"Cellularity of the p-Kazhdan-Lusztig basis for symmetric groups"

Abstract: After recalling the most important results about Kazhdan-Lusztig cells for symmetric groups, I will introduce the p-Kazhdan-Lusztig basis and give a complete description of p-cells for symmetric groups. After that I will mention important consequences of the Perron-Frobenius theorem for p-cells which provide one of the last missing ingredients for the proof of the cellularity of the p-Kazhdan-Lusztig basis in finite type A.

ball-Moebius

Online Representation Theory Seminar

Friday, April 30th, 2021 - h. 15:00
click HERE to attend the talk in streaming

Filippo AMBROSIO (EPFL Lausanne - Switzerland)
"Birational sheets in linear algebraic groups"

Abstract: The sheets of a variety X under the action of an algebraic group G are the irreducible components of subsets of elements of X with equidimensional G-orbits. For G complex connected reductive, the sheets for the adjoint action of G on its Lie algebra g were studied by Borho and Kraft in 1979. In 2016, Losev introduced finitely many subvarieties of g consisting of equidimensional orbits, called birational sheets: their definition is less immediate than the one of a sheet, but they enjoy better geometric and representation-theoretic properties and are central in Losev's suggestion of an Orbit method for semisimple Lie algebras.
In the opening part of the seminar we give a brief overview of sheets and recall some basics about Lusztig-Spaltenstein induction of conjugacy classes in terms of the so-called Springer generalized map and analyse its interplay with birationality. This will give the instruments to introduce Losev's birational sheets in g.
The main part is aimed at investigating analogues of birational sheets of conjugacy classes in G. To conclude, assuming that the derived subgroup of G is simply connected, we illustrate the main features of these varieties, comparing them with the objects defined by Losev.
Part of the talk is based on joint works with G. Carnovale and F. Esposito, and M. Costantini.

Calabi-spin

Online Representation Theory Seminar

Friday, April 23rd, 2021 - h. 15:00
click HERE to attend the talk in streaming

Kenji IOHARA (Université Lyon 1 - France)
"Elliptic root systems of non-reduced type"

Abstract: After explaining some known basic facts about elliptic root systems (ERS) of reduced type, I will show the classification and automorphism groups of ERS of non-reduced type. Some future problems will be discussed.
These results are obtained in collaboration with A. Fialowski and Y. Saito.

ball-Moebius

Online Representation Theory Seminar

Friday, April 16th, 2021 - h. 15:00
click HERE to attend the talk in streaming

Anton MELLIT (Universität Wien - Austria)
"Old and new identities for the nabla operator and counting affine permutations"

Abstract: An amazing nabla operator discovered by Bergeron and Garsia is a cornerstone of the theory of Macdonald polynomials. Applying it to various symmetric functions produces interesting generating functions of Dyck paths and parking functions. These kind of results are sometimes known as "shuffle theorems". I will try to give an overview of these results and explain how working with affine permutations and certain generalized P-tableaux allows to view them from a uniform point of view. The "new" in the title refers to the formula conjectured by Loehr and Warrington giving an explicit expansion of nabla of a Schur function in terms of nested Dyck paths.
This is a joint work with Erik Carlsson.

Calabi-spin

Online Representation Theory Seminar

Friday, April 9th, 2021 - h. 15:00
click HERE to attend the talk in streaming

Giovanna CARNOVALE (Università di Padova - Italy)
"Approximations of a Nichols algebra from a geometric point of view"

Abstract: The talk is based on work in progress with Francesco Esposito and Lleonard Rubio y Degrassi.
Recently Kapranov and Schechtman have settled an equivalence between the category of graded connected co-connected bialgebras in a braided monoidal category W and the category of factorizable systems of perverse sheaves on all symmetric products Symⁿ(C) with values in W. The Nichols (shuffle) algebra associated with an object V corresponds to the system of intersection cohomology extensions of a precise local system on the open strata.
Motivated by the study of Fomin-Kirillov algebras and their relation with Nichols algebras, we describe the factorizable perverse sheaves counterpart of some algebraic constructions, including the n-th approximation of a graded bialgebra, and we translate into geometric statements when a Nichols algebra is quadratic.

ball-Moebius

Online Representation Theory Seminar

Friday, March 26th, 2021 - h. 15:00
click HERE to attend the talk in streaming

Valerio TOLEDANO LAREDO (NE University Boston - USA)
"Stokes phenomena, Poisson-Lie groups and quantum groups"

Abstract: Let G be a complex reductive group, G* its dual Poisson-Lie group, and g the Lie algebra of G. G-valued Stokes phenomena were exploited by P. Boalch to linearise the Poisson structure on G*. I will explain how U(g)-valued Stokes phenomena can be used to give a purely transcendental construction of the quantum group U_h(g), and show that the semiclassical limit of this construction recovers Boalch's. The latter result is joint work with Xiaomeng Xu.

Calabi-spin

Online Representation Theory Seminar

Friday, March 19th, 2021 - h. 15:00
click HERE to attend the talk in streaming

Daniele VALERI (University of Glasgow - United Kingdom)
"Lax type operator for W-algebras"

Abstract: In 1985 Zamolodchikov constructed a "non-linear" extension of the Virasoro algebra known as W₃ algebra. This is one of the first appearances of a rich class of algebraic structures, known as W-algebras, which are intimately related to physical theories with symmetries and revealed many applications in mathematics. In the talk I will review some basic facts about the general theory of W-algebras and provide a description using Lax operators. This approach shows the deep connection of the theory of W-algebras with Yangians and integrable Hamiltonian hierarchies of Lax type equations.
This is a joint work with A. De Sole and V. G. Kac.

ball-Moebius

Online Representation Theory Seminar

Friday, March 12th, 2021 - h. 15:00
click HERE to attend the talk in streaming

Stephen GRIFFETH (Universidad de Talca - Chile)
"Ideals of subspace arrangements and representation theory"

Abstract: I will discuss results linking the study of certain highly symmetric arrangements of linear subspaces in affine space to representation theory, describing how to obtain qualitative information (e.g., is the arrangement Cohen-Macaulay?) and quantitative information (e.g., what is the Hilbert series of the ideal of the arrangement?) using techniques from the representation theory of Cherednik algebras.

Calabi-spin

Online Representation Theory Seminar

Friday, March 5th, 2021 - h. 15:00
click HERE to attend the talk in streaming

Olivier SCHIFFMANN (CNRS - Université Paris Saclay - France)
"Cohomological Hall algebras associated to ADE surface singularities"

Abstract: To a (reasonable) CY category C of global dimension two one can attach an associative algebra - its cohomological Hall algebra (COHA) - which is an algebra structure on the Borel-Moore homology of the stack of objects in C. In examples related to quivers (i.e. when C is the category of representations of the preprojective algebra of a quiver Q) this yields (positive halves) of Kac-Moody Yangians. In ongoing joint work with Diaconescu, Sala and Vasserot, we consider the case of the category of coherent sheaves supported on the exceptional locus of a Kleinian surface singularity. This is related to the above quiver case by a '2d-COHA' version of Cramer's theorem relating the (usual) Hall algebras of two hereditary categories which are derived equivalent.

rotating-knot

Friday, June 19th, 2020 - h. 14:30
- in streaming -
due to restrictions because of Covid-19 contamination

Salvatore STELLA ("Sapienza" Università di Roma)
"Cluster algebras and generalized minors reloaded"

N.B.: the slides of the talk are available here;
also, the full videorecording is available here.

Abstract: In a work with D. Rupel and H. Williams we showed that, given an acyclic finite or affine cluster algebra, its cluster monomials can be understood as generalized minors of the associated Kac-Moody group. The proof hinges upon a double recursion made possible by a technical tool, the double cambrian fan.
In this talk I will explain how one can prove a slightly weaker, but at the same time much more general result in a cleaner way. This is a work in progress in collaboration also with A. Appel.

The slides of the talk are available here;
also, the full videorecording is available here.

ball-Moebius

Friday, June 12th, 2020 - h. 14:30
- in streaming -
due to restrictions because of Covid-19 contamination

Sabino DI TRANI (Università di Firenze)
"The Reeder's Conjecture for Classical Lie Algebras"

N.B.: the slides of the talk are available here.

Abstract: A well known result of the first half of XX century asserts that the cohomology of a compact connected Lie group G is isomorphic as a graded vector space to the ring of G-invariants of the exterior algebra of g = Lie(G) . Finding Betti numbers of G then corresponds to identifying copies of the trivial representation in Λg. Reeder in '95 reduces this computation to the problem of finding copies of the trivial representation of the Weyl group of G in a suitable bi-graded algebra. As a generalization of this result, he conjectured that it is possible to compute the graded multiplicites in Λg of a special class of representations reducing to a similar "Weyl group representation"-problem. In the talk I will give a proof of the Reeder's Conjecture for the C_n case and present some new progress for type D.

The slides of the talk are available here.

Calabi-spin

Friday, June 5th, 2020 - h. 14:30
- in streaming -
due to restrictions because of Covid-19 contamination

Pawel DLOTKO (Swansea University)
"TDA for medical data analysis, how can we help in the current pandemic?"

N.B.: the slides of the talk are available here.

Abstract: Topological data analysis is a source of stable and explainable methods to analyze data. Those features are of the key importance in medical applications. In this talk I will review concepts of conventional and ball mapper. I will highlight how those tools have been used to analyze medical data, starting from work performed in Ayasdi, ending up in my work related to the current pandemic. I will finish by describing my work with Oxford Covid19 database (OxCDB). N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006

The slides of the talk are available here.

ball-Moebius

Friday, May 29th, 2020 - h. 14:30
- in streaming -
due to restrictions because of Covid-19 contamination

Mattia COLOMA (Università di Roma "Tor Vergata")
"The Hirzebruch-Riemann-Roch theorem in the fancy language of Spectra"

N.B.: the slides of the talk are available here.

Abstract: The category of spectra indubitably is the best of possible worlds for cohomology theories. For instance in spectra one can start with a few basic morphisms, be confident that every natural diagram built from them will commute, and end up with a proof of the Hirzebruch-Riemann-Roch theorem. As in every good story we'll have a deus ex machina: Atiyah's identification of the Spanier-Whitehead dual of a manifold with the Thom spectrum of minus its tangent bundle. I will try to gently introduce all of this tools assuming basic notions of topology, geometry and algebra.
Based on a joint work with Domenico Fiorenza and Eugenio Landi.

The slides of the talk are available here.

Calabi-spin

Friday, May 22nd, 2020 - h. 14:30
- in streaming -
due to restrictions because of Covid-19 contamination

Jorge VITÓRIA (Università di Cagliari)
"Quantity vs. size in representation theory"

N.B.: the slides of the talk are available here.

Abstract: Indecomposable modules over a finite-dimensional algebra R are largely thought of as the building blocks of the module category of R. A famous theorem of Auslander, Fuller-Reiten and Ringel-Tachikawa, states that a finite-dimensional algebra admits only finitely many indecomposable modules up to isomorphism if and only if every indecomposable module is finite-dimensional. This establishes a correlation between quantity (of indecomposable finite-dimensional modules) and size (of indecomposable modules).
In this talk, we will take a macroscopic view of the module category and look at certain subcategories of modules rather than individual modules. Our focus will be on torsion pairs, which are orthogonal decompositions of the module category. We will show that a finite-dimensional algebra admits only finitely many torsion classes if and only if every torsion class is generated by a finite-dimensional module. Time permitting, I will also make a few comments on how this relation between quantity and size transfers to the derived category of a finite-dimensional algebra. This talk is based on joint works with Lidia Angeleri Hügel, Frederik Marks and David Pauksztello.

The slides of the talk are available here.

ball-Moebius

Friday, May 15th, 2020 - h. 14:30
- in streaming -
due to restrictions because of Covid-19 contamination

Francesco SALA (Università di Pisa)
"Continuum Kac-Moody algebras (and their quantizations)"

N.B.: the slides of the talk are available here.

Abstract: In the present talk, I will define a family of infinite-dimensional Lie algebras associated with a "continuum" analog of Kac-Moody algebras. They depend on a "continuum" version of the notion of the quiver. These Lie algebras have some peculiar properties: for example, they do not have simple roots and in the description of them in terms of generators and relations, only quadratic (!) Serre type relations appear. If time permits, I will discuss their quantizations, called "continuum quantum groups".
This is based on joint works with Andrea Appel and Olivier Schiffmann.

The slides of the talk are available here.

Calabi-spin

Friday, May 8th, 2020 - h. 14:30
- in streaming -
due to restrictions because of Covid-19 contamination

Andrea APPEL (Università di Parma)
"Parabolic K-matrices for quantum groups"

N.B.: the slides of the talk are available here.

Abstract: Braided module categories provide a conceptual framework for the reflection equation, mimicking the relation between the Yang-Baxter equation and braided categories. Indeed, while the latter describes braids on a plane (type A), the former can be thought of in terms of braids on a cylinder (type B). In the theory of quantum groups, natural examples of braided module categories arise from quantum symmetric pairs (coideal subalgebras quantizing certain fixed point Lie subalgebra), where the action of type B braid groups is given in terms of a so-called universal K-matrix, constructed in finite-type by Balagovic-Kolb.
In this talk, I will describe the construction of a family of "parabolic" K-matrices for quantum Kac-Moody algebras, which is indexed by Dynkin subdiagrams of finite-type and includes Balagovic-Kolb K-matrix as a special case. If time permits, I will explain how this construction could lead to a meromorphic K-matrix for quantum loop algebras. This is based on joint works with D. Jordan and B. Vlaar.

The slides of the talk are available here.

ball-Moebius

Friday, April 24th, 2020 - h. 14:30
- in streaming -
due to mandatory restrictions because of Covid-19 contamination

Fabio GAVARINI (Università di Roma "Tor Vergata")
"Real forms of complex Lie superalgebras and supergroups"

N.B.: the slides of the talk are available here.

Abstract: A real form of a complex Lie algebra is the subset of fixed points of some "real structure", that is an antilinear involution; a similar description applies for real forms of complex (Lie or algebraic) groups. For complex Lie superalgebras, the notion of "real structure" extends in two different variants, called standard (a straightforward generalization) and graded (somewhat more sophisticated): the notion of "real form", however, stands problematic in the graded case.
I will present the functorial version of "real structure" (standard or graded), and show that the notion of "real form" then properly extends, in both cases; along the same lines, I will introduce real structures and real forms for complex supergroups. Then, basing on a suitable notion of "Hermitian form" on complex superspaces, I will introduce unitary Lie superalgebras and supergroups (again standard or graded); any Lie superalgebra which embeds into a unitary one will then be called "super-compact" - and similary for supergroups. Finally, I will give nice existence/uniqueness results of super-compact real forms for complex Lie superalgebras which are simple of basic (or "contragredient") type, and similarly for their associated connected simply-connected supergroups.
This is based on a joint work with Rita Fioresi, cf. arXiv:2003.10535 [math.RA] (2020).

The slides of the talk are available here.

Calabi-spin

Friday, April 17th, 2020 - h. 14:30
- in streaming -
due to mandatory restrictions because of Covid-19 contamination

Leonardo PATIMO (Albert Ludwigs Universität Freiburg)
"The quest for bases of the intersection cohomology of Schubert varieties"

N.B.: the slide of the talk are available here.

Abstract: The Schubert basis is a distinguished basis of the cohomology of a Schubert variety and it is a precious tool to study the ring structure of the cohomology.
When working with the intersection cohomology, we do not have a Schubert basis in general, and in fact understanding the intersection cohomology of a Schubert variety can be much more difficult. However, if one can understand well the related Kazhdan-Lusztig polynomials, one may often exploit their combinatorics and produce new bases in intersection cohomology which extend the original Schubert basis.
In this talk I would like to talk about two (if time permits!) different cases where this is possible. The first one are Schubert varieties in the Grassmannian. Here we obtain bases by "lifting" the combinatorics of Dyck partitions. The second case, joint with Nicolás Libedinsky, is the affine Weyl group Ã₂ . Here we realize our basis by defining a set of indecomposable light leaves.

The slides of the talk are available here.

ball-Moebius

Friday, April 3rd, 2020 - h. 14:30
- in streaming -
due to mandatory restrictions because of Covid-19 contamination

Martina LANINI (Università di Roma "Tor Vergata")
"Singularities of Schubert varieties within a right cell"

N.B.: the notes of the talk are available here.

Abstract: We describe an algorithm which takes as input any pair of permutations and gives as output two permutations lying in the same Kazhdan-Lusztig right cell. There is an isomorphism between the Richardson varieties corresponding to the two pairs of permutations which preserves the singularity type. This fact has applications in the study of W-graphs for symmetric groups, as well as in finding examples of reducible associated varieties of sl_n-highest weight modules, and comparing various bases of irreducible representations of the symmetric group or its Hecke algebra.
This is joint work with Peter McNamara.

The notes of the talk are available here.

Calabi-spin

Friday, March 27th, 2020 - h. 14:30
- in streaming -
due to mandatory restrictions because of Covid-19 contamination

Nicola CICCOLI (Università di Perugia)
"Orbit method via groupoid quantization"

N.B.: the slides of the talk are available here.

Abstract: The orbit method, in its most general form, can be seen as a general correspondence between symplectic leaves of a Poisson manifold and unitary irreducible representations of its quan-tization algebra. Properties of such correspondence should not depend on the choice of a specific quantization procedure. In this talk we will show how the so-called groupoid quantization allows to understand the correspondence for a wide family of quantum groups and their homogeneous spaces.

The slides of the talk are available here.

ball-Moebius

Friday, March 20th, 2020 - h. 15:00
(( beware of the unusual time!!! ))
- in streaming -
due to mandatory restrictions because of Covid-19 contamination

Sebastiano CARPI (Università di Roma "Tor Vergata")
"Weak quasi-Hopf algebras, vertex operator algebras and conformal nets"

N.B.: the slides of the talk are available here.

Abstract: Weak quasi-Hopf algebras give a generalization of Drinfeld's quasi-Hopf algebras. They were introduced by Mack and Schomerus in the early nineties in order to describe quantum symmetries of certain conformal field theories. Every fusion category is tensor equivalent to the representation category of a weak quasi-Hopf algebra. In particular the representation categories arising from rational conformal field theories such as the representation categories of strongly rational vertex operator algebras or of completely rational conformal nets can be described and studied by means of weak quasi-Hopf algebras.
In this talk I will discuss some aspects of the theory of weak quasi-Hopf algebras in connection with vertex operator algebras and conformal nets and explain some applications.
Based on a joint work in preparation with S. Ciamprone and C. Pinzari.

The slides of the talk are available here.

Calabi-spin

Friday, March 13th, 2020 - h. 14:30
(( beware of the unusual time!!! ))
- in streaming -
due to mandatory restrictions because of Covid-19 contamination

Mario MARIETTI (Università Politecnica delle Marche - Ancona)
"Weak generalized lifting property, Bruhat intervals and Coxeter matroids"

N.B.: the slides of the talk are available here.

Abstract: A natural generalization of the concept of a matroid is the concept of a Coxeter matroid, which was introduced by I. Gelfand and V. Serganova in 1987. In this talk, we will present a result stating that the Bruhat intervals of any arbitrary finite Coxeter group are Coxeter matroids. The main tool for proving this result is a new property, the weak generalized lifting property, which holds for all (finite and infinite) Coxeter groups and may have interest in its own right.
This is based on joint work with F. Caselli and M. D'Adderio.

The slides of the talk are available here.

ball-Moebius

Friday, February 28th, 2020
h. 14:00 - Room "Claudio D'Antoni"
Marco D'ANNA (Università di Catania)
"Almost Gorenstein rings and further generalizations: the 1-dimensional case"

Abstract: I will present the class of almost Gorenstein rings, with focus onto 1-dimensional (commutative, unital) local rings. This class of rings, introduced (by Barucci and Froberg) for algebroid curves, and recently extended (by Goto and others) to more general 1-dimensional rings, and late on in dimension greater than 1, has been intensively studied in the last years. In the 1-dimensional case definitions of other classes of rings have later been suggested that generalize Gorenstein rings and almost Gorenstein rings from different point of view: in particular, I will discuss one of them, which is motivated by the relations between the properties of the ring (R,m) and those of the R-algebra m:m in Q(R).
This is (partially) a joint work with Francesco Strazzanti.

Calabi-spin

Friday, February 21th, 2020
h. 14:00 - Room "Claudio D'Antoni"
Alessandro D'ANDREA ("Sapienza" Università di Roma)
"Irreducible representations of primitive Lie pseudoalgebras of type H"

Abstract: Lie pseudoalgebras are a "multivariable" generalization of Lie algebras. Their classification follows Cartan's 1909 classification of simple infinite dimensional linearly compact Lie algebras, in terms of four infinite families, called of type W, S, K and H. Finite irreducible representations in type W, S and K have already been classified by exploiting a common strategy, which, however, remarkably fails, in multiple points, in case H. In this talk I will explain all of the above issues and how to obtain a classification in type H as well.
This is a joint work with B. Bakalov and V. G. Kac.

ball-Moebius

Friday, February 14th, 2020
h. 14:00 - Room 1200
(( beware of the change of room!!! ))
Paolo PAPI ("Sapienza" Università di Roma)
"Yangians vs. minimal W-algebras: a surprising coincidence"

Abstract: I will discuss a proof of the fact that the singularities of the R-matrix R(k) of the minimal quantization of the adjoint representation of the Yangian associated with a Lie algebra g are opposite to the roots of the monic polynomial that expresses the OPE of conformal fields with conformal weight 3/2 in the affine W-algebra of level k associated with g. I will then explain some interesting consequences.
This is a joint work with V. G. Kac and P. Moseneder-Frajria.

Calabi-spin

Friday, February 7th, 2020
h. 14:00 - Room "Claudio D'Antoni"
Riccardo ARAGONA (Università de L'Aquila)
"Group theoretical approach for symmetric encryption"

Abstract: In 1949 Shannon gave the first abstract definition of cipher as a set of transformations on a message space. In 1975, Coppersmith and Grossman studied the group generated by a set of bijective transformations defining a cipher and the link of some properties of this group with the security of the corresponding cipher. From this work a new research sector in algebraic cryptography arises, that of the study of the properties of the group generated by the encryption functions of a cipher that can reveal weaknesses in the cipher itself.
In the first part of the talk, after presenting the algebraic background describing the structure of block ciphers, we will explain the link between the study of permutation groups and the study of the security of symmetric cryptosystems. In the second part of the talk we will present some new results that characterize the properties of the components of a block cipher which imply that the group generated by its encryption functions has those properties that make it resistant against known algebraic attacks.

ball-Moebius

Friday, January 24th, 2020
h. 14:00 - Room "Claudio D'Antoni"
Carlo Maria SCOPPOLA (Università de L'Aquila)
"Classifying p-groups?"

Abstract: During the last 100 years several ideas were suggested to address the classification problem of finite p-groups. In this talk I will remind some of them (Hall's isoclinism, the coclass theory) and I will talk about some recent progress. Then I will define the p-groups of Frobenius type, and I will remind some recent results in this setting too.

Calabi-spin

Friday, January 17th, 2020
h. 14:30 - Room "Claudio D'Antoni"
Kenji IOHARA (Université de Lyon 1)
"On elliptic root systems"

Abstract: Elliptic root systems are introduced in 1985 by K. Saito having simply elliptic singularities in mind. In this talk, the state of art around elliptic root systems will be explained.

ball-Moebius

Friday, January 10th, 2020
h. 15:45 - Room "Claudio D'Antoni"
Peter McNAMARA (University of Melbourne)
"Geometric Extension Algebras"

Abstract: A number of algebras that we study in Lie theory have geometric interpretations, appearing as a convolution algebra in Borel-Moore homology or equivalently as the Ext-algebra of a pushforward sheaf. We will discuss how information on the representation theoretic side (like being quasihereditary) is related to information on the geometric side (like odd cohomology vanishing). The primary application is to KLR and related algebras.

Calabi-spin

Friday, January 10th, 2020
h. 14:30 - Room "Claudio D'Antoni"
Markus REINEKE (University of Bochum)
"Cohomological Hall algebras of quivers"

Abstract: Cohomological Hall algebras form a class of graded algebras which are defined by a convolution operation on representation spaces of quivers. In the talk, we will motivate their definition, construct them, and review basic properties and known structural results. Then we turn to the special case of the Kronecker quiver and derive a description by generators and relations of the corresponding cohomological Hall algebra, which is related to Yangians. N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006

rotating-knot

Friday, December 13th, 2019
h. 14:30 - Room "Claudio D'Antoni"
Alexander PÜTZ (University of Rome "Tor Vergata")
"Linear degenerations of affine Grassmannians and moment graphs"

Abstract: Every projective variety is a quiver Grassmannian. Hence, we can use representation theory of quivers to study the geometry of certain projective varieties. In this talk we apply it to study the affine Grassmannian. Namely we identify certain finite approximations of it with quiver Grassmannians for the loop quiver. In this way we can study the geometry of the affine Grass-mannian via the limit of the approximations. We also examine how the geometry changes with linear degenerations and find out that it behaves very different from the non-affine setting. There is an action of a one-dimensional torus on the affine Grassmannian and its linear degenerations which induces a cellular decomposition. Based on the combinatorics of this decomposition we can compute Euler characteristics, Poincaré polynomials and cohomology. In the non-degenerate setting, we rediscover some results obtained with the combinatorics of the affine Weyl group.

ball-Moebius

Friday, December 6th, 2019
h. 14:30 - Room "Claudio D'Antoni"
Niels KOWALZIG (University of Napoli "Federico II")
"Cyclic Gerstenhaber-Schack cohomology"

Abstract: In this talk, we answer a long-standins question by explaining how the diagonal complex computing the Gerstenhaber-Schack cohomology of a bialgebra (that is, the cohomology theory governing bialgebra deformations) can be given the structure of an operad with multiplication if the bialgebra is a (not necessarily finite dimensional) Hopf algebra with invertible antipode; if the antipode is involutive, the operad is even cyclic. Therefore, the Gerstenhaber-Schack cohomology of any such Hopf algebra carries a Gerstenhaber, resp. Batalin-Vilkovisky, algebra structure; in particular, one obtains a cup product and a cyclic boundary B that generate the Gerstenhaber bracket, and that allows to define cyclic Gerstenhaber-Schack cohomology. In case the Hopf algebra in question is finite dimensional, the Gerstenhaber bracket turns out to be zero in cohomology and hence the interesting structure is not given by this e2-algebra structure, which is expressed in terms of the cup product and B.

Calabi-spin

Friday, November 29th, 2019
h. 14:30 - Room "Claudio D'Antoni"
Sean GRIFFIN (University of Washington)
"Ordered set partitions, Tanisaki ideals, and rank varieties"

Abstract: We introduce a family of ideals I_n,λ in Q[x₁,...,x_n] for λ a partition of k≤n. This family contains both the Tanisaki ideals and the ideals I_n,k of Haglund-Rhoades-Shimozono as special cases. We study the corresponding quotient rings R_n,λ as symmetric group modules. We give a monomial basis for R_n,λ in terms of (n,λ)-staircases, unifying the monomial bases studied by Garsia-Procesi and Haglund-Rhoades-Shimozono. Furthermore, we realize the S_n-module structure of R_n,λ in terms of an action on (n,λ)-ordered set partitions. We then prove that the graded Frobenius characteristic of R_n,λ has a positive expansion in terms of dual Hall-Littlewood functions. Finally, we use results of Weyman to connect the quotient rings R_n,λ to Eisenbud-Saltman rank varieties. This allows us to generalize results of De Concini-Procesi and Tanisaki on "nilpotent" diagonal matrices.

ball-Moebius

Friday, November 22nd, 2019
h. 14:30 - Room "Claudio D'Antoni"
Ivan PENKOV (Jacobs University, Bremen)
"Some older and some recent results on the ind-varieties G/P for the ind-groups G = GL(∞) , O(∞) , Sp(∞)"

Abstract: About 15 years ago, Dimitrov and I worked out the flag realizations of the homogeneous ind-varieties GL(∞)/P for arbitrary splitting parabolic ind-subgroups P. An essential difference from the finite-dimensional case is that we have to work with generalized flags, not with usual flags. Generalized flags are chains of subspaces which have more interesting linear orders. In the first part of the talk, I will recall our results with Dimitrov. In the second part, I will explain(without proof) two recent results. The first one (joint with A. Tikhomirov) is a purely algebraic-geometric construction of the ind-varieties of generalized flags. The second one (joint with L.Fresse) answers the following question: on which multiple ind-varieties of generalized flags, i.e. direct products of ind-varieties of generalized flags, does GL(∞) act with finitely many orbits? N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006

Calabi-spin

rotating-knot

Workshop
"ARTS in Rome"

A Representation Theory Summit in Rome
Friday-Saturday, November 15-16th, 2019
via Lucullo 11, Roma - ITALY

WARNING: due to space limitations in the conference venue, only registered participants will be allowed to attend the meeting.

Speakers & Talks:

Peter FIEBIG (Erlangen) - Friday 15th, h. 15:00-16:00
"Lefschetz operators and tilting modules"

Carolina VALLEJO (Madrid) - Friday 15th, h. 16:30-17:30
"Galois action on the principal block"

David HERNANDEZ (Paris 7) - Friday 15th, h. 17:45-18:45
"Grothendieck ring isomorphims, cluster algebras and Kazhdan-Lusztig polynomials"

Gunter MALLE (Kaiserslautern) - Saturday 16th, h. 9:00-10:00
"Blocks and Lusztig induction"

Olivier DUDAS (Paris 7) - Saturday 16th, h. 10:15-11:15
"Modular reduction of unipotent characters"

Maria CHLOUVERAKI (Versailles) - Saturday 16th, h. 11:45-12:45
"Are complex reflection groups real?"

N.B.: this workshop is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006

ball-Moebius

Friday, November 8th, 2019
h. 14:30 - Room "Claudio D'Antoni"
Simon RICHE (Université Clermont Auvergne)
"Central sheaves on affine flag varieties"

Abstract: Gaitsgory's construction of "central sheaves" on the affine flag variety of a reductive algebraic group is a fundamental tool in the Geometric Langlands Program, with important applications to the geometry of Shimura varieties. In this talk I will review this construction, and explain how it generalizes to arbitrary rings of coefficients. I will also explain its main application in Geometric Representation Theory, namely an equivalence of categories due to Arkhipov-Bezrukavnikov relating constructible sheaves on the affine flag variety to equivariant coherent sheaves on the Springer resolution of the Langlands dual group, together with an extension to modular coefficients. This will be based on joint works in progress with Achar and with Bezrukavnikov-Rider.

Calabi-spin

N.B.: questo seminario si svolge in "Sapienza" Università di Roma
dipartimento di Matematica "Guido Castelnuovo"
Thursday, October 24th, 2019
h. 15:30 - Room B
Kyokazu NAGATOMO (Osaka University)
"Vertex operator algebras whose dimensions of weight one spaces are 8 and 16"

Abstract: We classify (strongly regular) vertex operator algebras (VOAs) V of CFT type, whose spaces generated by characters are equal to spaces solutions of monic modular linear differential equations of third order, which have two parameters; expected central charge c and conformal weight. Among VOAs with these properties, we focus on one, such that the weight one space V₁ is 8 or 16-dimensional, respectively, since VOAs which have a non-trivial automorphism with a fixed point have such dimensions. The typical examples of such VOAs are lattice VOAs associated with the √2E₈ lattice for c = 8 and the Barnes-Walls lattice (denoted by Λ₁₆) for c = 16, respectively. This is because central charges and dimV₁ of lattice VOAs are equal to ranks of the corresponding lattices. Of course, we could study VOAs with central charge 8 and 16, which is certainly more natural. However, it is would be (was) not easy to characterize such VOAs since there exist solutions that are independent of one of two parameters. If the character of V is free of a parameter, we cannot determine expected central charge. We may consider the characters of V-modules, in fact we did try to figure out c = 8 and 16 cases. But it was not very successful since first coefficients of V-modules are not always 1 (in our method this property is crucial). First we have shown that VOAs whose space of characters in our interest is equal to one of the √2E₈ lattice VOA for dimV₁ = 8 and one of the Barnes-Walls lattice VOA V_Λ₁₆ for dimV₁ = 18, respectively. Moreover, we showed that V is isomorphic to V_√2E₈ for c = 8 under a mild condition. We expect the same as for c = 16.

ball-Moebius

Friday, October 18th, 2019
h. 14:00 - Room "Claudio D'Antoni"
(( beware of the change of time!!! ))
Yusra NAQVI (University of Sidney)
"A gallery model for affine flag varieties"

Abstract: Positively folded galleries in Coxeter complexes play a role in many areas of maths, such as in the study of affine Hecke algebras, Macdonald polynomials, MV-polytopes, and affine Deligne-Lusztig varieties. In this talk, we will define positively folded galleries, and then look at a new recursive description of the set of end alcoves of folded galleries which are positive with respect to alcove-induced orientations. This further allows us to find the images of retractions from points at infinity, giving us a combinatorial description of certain double coset intersections in the affine flag variety.
This talk is based on joint work with Elizabeth Milićević, Petra Schwer and Anne Thomas. N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006

Calabi-spin

Friday, October 4th, 2019
h. 14:30 - Room "Claudio D'Antoni"
Gianluca MARZO (Università degli Studi di Roma "Tor Vergata")
"Functorial resolution of singularities"

Abstract: Resolution of singularities, already in the category of complex analytic spaces, cannot be achieved in a way that is both étale local and independent of the resolution process itself while blowing up in smooth centres. However, we will explain how this can be achieved in the 2-category of Deligne-Mumford champ following a new method based on smoothed weighted blow up in regular centre. We construct an invariant, inv, of regular m-dimensional characteristic zero local rings and their ideals, that makes the resolution process functorial and more widely applicable, rather than just complex spaces. The goal of the talk is to relate the construction of the invariant with a functorial definition of the Newton polyhedron of an ideal, and show how we get a very easy and fully functorial resolution of complex singularities.
This is based on joint work with M. McQuillan.

ball-Moebius

Thursday, June 20th, 2019
h. 14:30 - Room "Roberta Dal Passo"
Kirill ZAYNULLIN (University of Ottawa)
"Hyperplane sections of Grassmannians and the equivariant cohomology"

Abstract: We study a family of hyperplane sections of Grassmannians from the point of view of the GKM-theory. Starting from the Schubert divisor which corresponds to the most singular section and has a natural torus action we provide a uniform description of the equivariant cohomology of the whole family of sections including the smooth one.
This is a joint work in progress with Martina Lanini.

Calabi-spin

Monday, June 10th, 2019
h. 14:30 - Room "Roberta Dal Passo"
Magdalena BOOS (Ruhr-Universität, Bochum)
"Towards degenerations for algebras with self-dualities"

Abstract: A parabolic subgroup P of a classical Lie group G acts on the nilpotent coneN of nilpotent complex matrices in Lie(G) via conjugation. If N is restricted to the subvariety of 2-nilpotent matrices, then the number of orbits is finite and we can describe a parametrization of the orbits by using a translation to the symmetric representation theory of a finite-dimensional algebra with self-duality. Our main goal is the description of the orbit closures and we discuss first results. These results are obtained via degenerations of symmmetric representations of a certain algebra with self-duality, and we show which of them hold in general.
This is based on work in progress with G. Cerulli Irelli and F. Esposito.

ball-Moebius

Monday, June 3rd, 2019
h. 12:00 - Room "Roberta Dal Passo"
Rita FIORESI (Università di Bologna)
(( beware of the change of time!!! ))
"Quantum principal bundles over projective bases"

Abstract: In non commutative geometry, a quantum principal bundle over an affine base is recovered through a deformation of the algebra of its global sections: the property of being a principal bundle is encoded by the notion of Hopf Galois extension, while the local triviality is expressed by the cleft property. The quantum algebra of the base space is realized as suitable coinvariants inside the global sections of the quantum principal bundle.
We want to examine the case of a projective base X in the special case X=G/P , where G is a complex semisimple group and P a parabolic subgroup. We will substitute the coordinate ring of X with the homogeneous coordinate ring of X with respect to a projective embedding, corresponding to a line bundle L obtained via a character of P. The quantization of the line bundle will come through the notion of quantum section and the quantizations of the base (a quantum flag) will be obtained as semi-coinvariants. The quantization of G will then be interpreted as the quantum principal bundle on the quantum base space X, obtained via a quantum section.
(joint work with P. Aschieri and E. Latini)

Calabi-spin

Monday, May 27th, 2019
h. 14:30 - Room "Roberta Dal Passo"
Margherita PAOLINI
"The integral form of the universal enveloping algebra of twisted affine sl₃"

Abstract: In the representation theory of a semisimple Lie algebra L, the subring of the universal enveloping algebra U(L) generated by suitable divided powers arises naturally, thus leading to construct an integral form of U(L). Kostant and Cartier indipendently defined this form and explicitly constructed integral bases when L is finite. Their construction has later been generalized to the untwisted affine case by Garland.
An analogous work by Fisher-Vasta extends the construction of the integral form of U(L) to the affine twisted Kac-Moody algebra of rank 1 (type A²₂). These works are based on complicated commutation formulas, whose regularity remains hidden; moreover, in the twisted case there are some problems both with the statement and the proof.
The aim of this talk is to give a correct description of the integral form of the enveloping algebra of type A²₂ , providing explicit and compact commutation relations, so to reach a deeper comprehension and drastic simplification of the problem. This is achieved by means of a careful use of the generating series of families of elements and of the properties of the ring of symmetric functions.

ball-Moebius

Tuesday, May 7th, 2019
(( beware of the change of day and time!!! ))
h. 15:15 - Room "Roberta Dal Passo"
Gastón Andrés GARCÍA (Universidad Nacional de La Plata)
"Pointed Hopf algebras, quantum groups and the lifting method"

N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006
Abstract: This talk will focus on the classification of pointed Hopf algebras and the description of a general method (called "lifting method") that was the key stone to solve the problem of classifying finite-dimensional pointed Hopf algebras over Abelian groups. It turned out that Hopf algebras of this type are all isomorphic to variations of (Borel parts of) small quantum groups. The implementation of this method is based on the notions of Nichols algebras, braided spaces and PBW-deformations, and led to the development of generalized root systems and Weyl groupoids.
Time permitting, I will present a generalized lifting method that allows to construct new examples of non-pointed Hopf algebras related to quotients of quantized coordinate algebras over simple algebraic groups.

Calabi-spin

Tuesday, May 7th, 2019
(( beware of the change of day and time!!! ))
h. 14:00 - Room "Roberta Dal Passo"
Gastón Andrés GARCÍA (Universidad Nacional de La Plata)
"Classifying finite-dimensional Hopf algebras"

N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006
Abstract: In this talk I will introduce the problem of classifying (finite-dimensional) Hopf algebras over an algebraically closed field of characteristic zero. This is a difficult question, since the theory of Hopf algebras includes as first examples group algebras and universal enveloping algebras of Lie algebras. Up to know, there are very few general results, hence all efforts are focused on solving the classification problem for certain families of Hopf algebras: e.g., the semisimple ones, the pointed ones, or those of small dimension.
The main obstruction lies in the lack of enough examples. Although the appearance of Quantum Groups gave a strong impulse to the theory, drawing the attention of mathematicians from different areas (representation theory, mathematical physics, QCFT, etc.), the problem is far from being solved.
In this talk I will present some structural results, different techniques for classifying particular families of Hopf algebras, and then describe the current situation of the classification problem for small dimension.

ball-Moebius

Monday, April 29th, 2019
h. 14:30 - Room "Roberta Dal Passo"
Carmelo Antonio FINOCCHIARO (Università di Catania)
"Spectral spaces of rings and modules and applications"

Abstract: Let K be a field and D be a subring of K. The space Zar(K/D) of all the valuation domains of K containing D as a subring can be endowed with the topology, called the Zariski topology, generated by the sets of the type Zar(K/D[x]) , for every x in K. In [C. A. Finocchiaro, M. Fontana, K. A. Loper, "The constructible topology on spaces of valuation domains", Trans. Amer. Math. Soc. 365, n. 12 (2013), 6199-6216] it was proved that Zar(K/D) is a spectral space and a ring whose prime spectrum is homeomorphic to Zar(K/D) was explicitly provided. In this talk we will introduce a spectral extension of Zar(K/D) , that is, the space of all D-submodules of K. More generally, given any ring A, a Zariski-like spectral topology can be given to the space S_A(M) of A-submodules of an A-module M. Some application to flat modules (see [C. A. Finocchiaro, D. Spirito, "Topology, intersection of modules and flat modules", Proc. Amer. Math. Soc. 144 (2016), no. 10, 4125-4133]) will be presented.

Calabi-spin

Monday, April 15th, 2019
h. 14:30 - Room "Roberta Dal Passo"
Andrea MAFFEI (Università di Pisa)
"Equazioni differenziali con singolarità di tipo Oper"

Abstract: Lo spazio degli Oper regolari è una famiglia di equazioni differenziali lineari in una variabile complessa t, dipendenti da un parametro x e con una singolarità in t = x , associati ad un gruppo semisemplice G. Nel caso di G = SL(2) nel seminario verranno introdotte e studiate delle famiglie di equazioni differenziali dipendenti da due parametri x, y con singolarità in t = x e t = y e che per x diverso da y sono oper regolari vicino a x e vicino a y . Verrà determinato il tipo di equazioni che si ottiene per x = y .
I risultati descritti sono parte di un progetto di ricerca in collaborazione con Giorgia Fortuna.

ball-Moebius

Monday, April 8th, 2019
h. 14:30 - Room "Roberta Dal Passo"
Martina LANINI (Università di Roma "Tor Vergata")
"Combinatorial Fock space and representations of quantum groups at roots of unity"

Abstract: The classical Fock space arises in the context of mathematical physics, where one would like to describe the behaviour of certain configurations with an unknown number of identical, non-interacting particles. By work of Leclerc and Thibon, it(s q-analogue) has a realisation in terms of the affine Hecke algebra of type A and it controls the representation theory of the corresponding quantum group at a root of unity. In joint work with Arun Ram and Paul Sobaje, we produce a generalisation of the q-Fock space to all Lie types. This gadget can also be realised in terms of affine Hecke algebra and captures decomposition numbers for quantum groups at roots of unity.

Calabi-spin

Monday, April 1st, 2019
h. 14:30 - Room "Roberta Dal Passo"
Dmitriy RUMYNIN (University of Warwick)
"Kac-Moody Groups: representations, localisation, duality"

N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006
Abstract: We will look at representation theory of a complete Kac-Moody group G over a finite field. G is a locally compact totally disconnected group, similar, yet slightly different to the group of points of a reductive group scheme over a local field. After defining the group we will prove that the category of smooth representations has finite homological dimension. At the end we discuss localisation and homological duality for this category.

ball-Moebius

Monday, March 25th, 2019
h. 14:30 - Room "Roberta Dal Passo"
Claudio PROCESI ("Sapienza" Università di Roma - Accademia dei Lincei)
"Perpetuants: a lost treasure"

Abstract: Perpetuant is one of the several concepts invented (in 1882) by J. J. Sylvester in his investigations of covariants for binary forms. It appears in one of the first issues of the American Journal of Mathematics which he had founded a few years before. It is a name which will hardly appear in a mathematical paper of the last 70 years, due to the complex history of invariant theory which was at some time declared dead only to resurrect several decades later. I learned of this word from Gian-Carlo Rota who pronounced it with an enigmatic smile.
In this talk I want to explain the concept, a Theorem of Stroh, and some new explicit description.

Calabi-spin

Escher-birds

Colloquium di Dipartimento
Monday, March 11th, 2019
h. 14:30 - Room "Roberta Dal Passo"
Wolfgang SOERGEL
(Freiburg University)

"Kazhdan-Lusztig Theory"

N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006
Abstract: The study of continous actions of groups like GL(n;R) and GL(n;C) on Banach spaces leads to interesting algebraic questions.
This field has seen great progress recently, and I want to talk on it.

ball-Moebius

Monday, March 4th, 2019
h. 14:30 - Room "Roberta Dal Passo"
Viola SICONOLFI (Università di Roma "Tor Vergata")
"Wonderful models for generalized Dowling lattices"

Abstract: Given a subspace arrangement, De Concini and Procesi in the '90s described the construction of a variety associated to it, namely its wonderful model. An important feature of these model is that some of its geometric aspects are linked to some combinatorical properties of the subspace arrangement, in particular the description of its boundary and its Betti numbers.
During the talk I will consider the subspace arrangement associated to a generalized Dowling lattice, a combinatorial object introduced by Hanlon.
The aim is to study the wonderful model associated to it and to give a description of its boundary. To deal with this I will use a bijection between the set of boundary components of the wonderful model and a family of graphs. This is a joint work with Giovanni Gaiffi.

Calabi-spin

Monday, February 25th, 2019
h. 14:30 - Room "Claudio D'Antoni"
Ghislain FOURIER (RWTH Aachen)
"Recent developments on degenerations of flag and Schubert varieties"

N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006
Abstract: I'll recall flag varieties and Schubert varieties, and building on that PBW and linear degenerations. This first part is meant to be an introduction for Master and Phd-students.
I'll proceed with recent results on PBW degenerations of Schubert varieties, explaining triangular and rectangular Weyl group elements. The talk will end with several open questions, discussing the current limit of generalizations.

ball-Moebius

Tuesday, February 19th, 2019
h. 14:30 - Room "Claudio D'Antoni"
Benoit FRESSE (Université de Lille)
"Kontsevich' graph complexes, operadic mapping spaces, and the Grothendieck-Teichmüller group"

N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006
Abstract: I will report on a joint work with Victor Turchin and Thomas Willwacher about the applications of graph complexes to the study of mapping spaces associated to E_n-operads.
The class of E_n-operads consists of objects that are homotopy equivalent to a reference model, the operad of little n-disks, which was introduced by Boardman-Vogt in topology. I will briefly review the definition of these objects.
The main goal of my talk is to explain that the rational homotopy of mapping spaces of E_n-operads has a combinatorial description in terms of the homology of Kontsevich' graph complexes. This approach can also be used for the study of homotopy automorphism spaces associated to En-operads. In the case n=2 , one can identify the result of this computation with the pro-unipotent Grothedieck-Teichmüller group.
The proof of these statements relies on results on the rational homotopy of E_n-operads which I will also briefly explain in my talk.

Calabi-spin

Monday, February 4th, 2019
h. 14:30 - Room "Roberta Dal Passo"
Paolo SENTINELLI (Universidad de Chile - Santiago)
"The Jones-Wenzl idempotent of a generalized Temperley-Lieb algebra"

Abstract: Given a finite dimensional generalized Temperley-Lieb algebra , defined as a quotient of the Hecke algebra of a finite Coxeter group, we will define its Jones-Wenzl idempotente, in analogy with the classical case (type A).
In type B, these algebras have recently prove useful for the construction of knot invariants on the solid torus. On the other hand, in type A, the Jones-Wenzl idempotent appear in the construction of coloured Jones polynomials; thus one can believe that similar constructions can be found in type B or in other typer (where it makes sense to do some knot theory).
Taking into account a basis (the one indexed by the so-called fully commutative elements) which is seldom used in this context, we will show a way to obtain recursive formulas for the Jones-Wenzl idempotent, that are new even in the classical case, from which we shall deduce explicitly some coefficients of its expansion with respect to the above mentioned basis (namely, those related with the maxima of the minuscule quotients). These coefficients seem to be important in positive characteristic.

ball-Moebius

Monday, January 28th, 2019
h. 14:30 - Room "Roberta Dal Passo"
Paolo LIPPARINI (Università di Roma "Tor Vergata")
"Introduction to universal algebra"

Abstract: I will present a brief overview of some classical results in universal algebra, trying to stress the links with the study of algebra in a more "classical" sense, or the lack of such links.
Time permitting, I will consider some more recent developments, such as commutator theory and Hobby and McKenzie's classification of finite algebraic structures.

Calabi-spin

Monday, January 21st, 2019
h. 16:00 - Room "Claudio D'Antoni"
Sam GUNNINGHAM (University of Edinburgh)
"Quantum character theory"

Abstract: I will give an overview of various approaches to studying character varieties (moduli spaces of local systems on manifolds) using tools of geometric representation theory and topological field theory. For example, in my work with Ben-Zvi and Nadler we show that the homology groups of character varieties (which are the subject of fascinating conjectures of Hausel and Rodriguez Villegas) are extracted from a certain topological field theory associated to the monoidal category of Harish-Chandra bimodules. A closely related topological field theory constructed by Ben-Zvi, Brochier, and Jordan defines canonical quantization of character varieties associated to the quantum group; in my ongoing work with David Jordan we are investigating how this theory computes invariants of knots and skeins in 3-manifolds via q-analogues of character sheaves and Harish-Chandra bimodules.
I will not assume any priori familiarity with any of these concepts.

ball-Moebius

Monday, January 14th, 2019
h. 16:00 - Room "Claudio D'Antoni"
Corrado DE CONCINI ("Sapienza" - Università di Roma)
"Projective Wonderful Models for Toric Arrangements and their Cohomology"

Abstract: (joint work with Giovanni Gaiffi) I plan to sketch an algorithmic procedure which allows to build projective wonderful models for the complement of a toric arrangement in a n-dimensional algebraic torus T in analogy with the case of subspaces in a linear or projective space. The main step of the construction is a combinatorial algorithm that produces a projective toric variety in which the closure of each layer of the arrangement is smooth.
The explicit procedure of our construction allows us to describe the integer cohomology rings of such models by generators and relations.

rotating-knot

Monday, December 10th, 2018
h. 14:30 - Room "Roberta Dal Passo"
Gwyn BELLAMY (Glasgow University)
"Resolutions of symplectic quotient singularities"

N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006
Abstract: In this talk I will explain how one can explicitly construct all crepant resolutions of the symplectic quotient singularities associated to wreath product groups. The resolutions are all given by Nakajima quiver varieties. In order to prove that all resolutions are obtained this way, one needs to describe what happens to the geometry as one crosses the walls inside the GIT parameter space for these quiver varieties. This is based on joint work with Alistair Craw.

Calabi-spin

Monday, December 3rd, 2018
h. 14:30 - Room "Roberta Dal Passo"
Spela SPENKO (Vrije Universiteit Brussel)
"Comparing commutative and noncommutative resolutions of singularities"

Abstract: Quotient singularities for reductive groups admit the canonical Kirwan (partial) resolution of singularities, and often also a noncommutative resolution. We will motivate the occurrence of noncommutative resolutions and compare them to their commutative counterparts (via derived categories in terms of the Bondal-Orlov conjecture). This is a joint work with Michel Van den Bergh.

ball-Moebius

Workshop
"Representation theory in Rome
and Beyond"

Eli & Vel

Friday, November 16th, 2018
h. 14:00 - Room "Roberta Dal Passo"

N.B.: this workshop is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006

h. 14:15 - Welcome speech
by Giulia Maria PIACENTINI CATTANEO
(Università di Roma "Tor Vergata")

h. 14:30 - Michel BRION (Université de Grenoble)
"Automorphisms of almost homogeneous varieties"

Abstract: The automorphism group of a projective variety X is known to be a "locally algebraic group", extension of a discrete group (the group of components) by a connected algebraic group. But the group of components of Aut(X) is quite mysterious; in particular, it is not necessarily finitely generated.
In this talk, we will discuss the structure of Aut(X) when X has an action of an algebraic group with an open dense orbit. In particular, we will see that the group of components is arithmetic (and hence finitely generated) under this assumption.

h. 15:45 - Michèle VERGNE (Inst. Math. Jussieu / Académie de Sciences - Paris)
"Quiver Grassmannians, Q-intersection and Horn conditions"

Abstract:
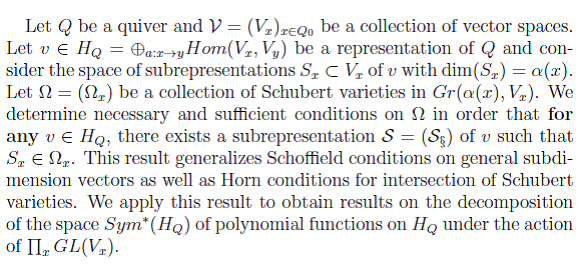
Vergne abstract

h. 16:45 - Coffee Break
**☆ ☆ ☆ Greeting Toasts for Elisabetta and Velleda ☆ ☆ ☆**

h. 17:15 - Peter LITTELMANN (Cologne University)
"Standard Monomial Theory via Newton-Okounkov Theory"

Abstract: Sequences of Schubert varieties, contained in each other and successively of codimension one, naturally lead to valuations on the field of rational functions of the flag variety. By taking the minimum over all these valuations, one gets a quasi valuation which leads to a flat semi-toric degeneration of the flag variety. This semitoric degeneration is strongly related to the Standard Monomial Theory on flag varieties as originally initiated by Seshadri, Lakshmibai and Musili. This is work in progress jointly with Rocco Chirivi and Xin Fang.

Calabi-spin

Monday, November 5th, 2018
h. 16:00 - Room "Roberta Dal Passo"
Niels KOWALZIG (Università di Napoli "Federico II")
"Higher brackets on cyclic and negative cyclic (co)homology"

Abstract: In this talk, we will embed the string topology bracket developed by Chas-Sullivan and Menichi on negative cyclic cohomology groups as well as the dual bracket found by de Thanhoffer de Voelcsey-Van den Bergh on negative cyclic homology groups into the global picture of a noncommutative differential (or Cartan) calculus up to homotopy on the (co)cyclic bicomplex in general, in case a certain Poincaré duality is given. For negative cyclic cohomology, this in particular leads to a Batalin-Vilkovisky algebra structure on the underlying Hochschild cohomology. In the special case in which this BV bracket vanishes, one obtains an e_3-algebra structure on Hochschild cohomology. The results are given in the general and unifying setting of (opposite) cyclic modules over (cyclic) operads.
All this is joint work with D. Fiorenza.

ball-Moebius

Monday, November 5th, 2018
h. 14:30 - Room TBA
Paolo BRAVI ("Sapienza" Università di Roma)
"Spherical functions and orthogonal polynomials"

Abstract: I will explain how some questions we asked a few years ago on the multiplication of spherical functions on symmetric spaces are related to the so-called linearization problem for a certain kind of orthogonal polynomials, namely Jacobi polynomials. I will state some conjectures in the particular case of Jack polynomials.

Calabi-spin

Monday, October 22nd, 2018
h. 16:00 - Room "Roberta Dal Passo"
Layla SORKATTI (Al-Neelain University, Khartoum)
"Symplectic alternating algebras"

N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006
Abstract: We first give some general overview of symplectic alternating algebras and then focus in particular on the structure and classification of nilpotent symplectic alternating algebras.

ball-Moebius

Monday, September 24th, 2018
h. 14:30 - Room "Claudio D'Antoni"
Pramod N. ACHAR (Louisiana State University)
"The Humphreys conjecture on support varieties of tilting modules"

N.B.: this talk is part of the activity of the MIUR Excellence Department Project CUP E83C18000100006
Abstract: Let G be a reductive algebraic group over a field of positive characteristic. This talk is about geometric invariants of representations of G. Given a finite-dimensional G-representation V, classical results of Andersen-Jantzen and Friedlander-Parshall make it possible to associate to V a certain subset of the nilpotent elements in the Lie algebra of G, called the "support variety of V". About 20 years ago, Humphreys proposed a conjectural description of the support variety for an important class of modules called tilting modules. I will discuss recent progress on this conjecture. This is joint work with William Hardesty and Simon Riche.

Calabi-spin

Friday, June 8th, 2018
h. 17:00 - Room "Claudio D'Antoni"
Jacinta TORRES (KIT - Karlsruher Institut für Technologie)
"Kostant Convexity and the Affine Grassmannian"

Abstract: We present some ideas and results towards a building-theoretical affine Grassmannian. One of our aims is to substitute many proofs carried out using relations in the Kac-Moody group using certain retractions. This is joint work in progress with Petra Schwer.

ball-Moebius

Friday, June 8th, 2018
h. 15:30 - Room "Claudio D'Antoni"
Gabriele GULLÀ (Università di Roma "Tor Vergata")
"Logical methods across mathematics: three examples in algebra"

Abstract: In this seminar I will talk about three well known examples of algebraic problems which have been engaged with logical tools (in particular set theoretic tools).
The first one, due to Patrick Dehornoy, is about the use of very high Large Cardinals Axioms to solve problems linked to Laver Tables, which are objects closely related to Braids theory.
The second one is about the study of relations among Forcing Axioms (which are extensions of Baire Category Theorem) and Operator Algebras, in particular C^*-algebra problems. This field of research has particularly grown thanks to Ilijas Farah and Nick Weaver.
The last one concerns the proof (by Saharon Shelah, 1974) of the independence of Whitehead Problem (a group theory problem from the '50s) from ZFC (the usual Zermelo-Fraenkel set theory with the Axiom of Choice). In this example in particular the set theoretic ideas which are useful are the Continuum Hypothesis (which can be considered as a cardinal assumption), Martin's Axiom (a specific Forcing Axiom) and the Axiom of Constructibility which is, in a certain way, an anti-Large Cardinal axiom.

Calabi-spin

Friday, May 25th, 2018
h. 16:30 - Room "Roberta Dal Passo"
Giovanni CERULLI IRELLI ("Sapienza" Università di Roma)
"Cellular decomposition of quiver Grassmannians"

Abstract: I will report on a joint project with F. Esposito, H. Franzen and M. Reineke - cf. arXiv:1804.07736. Quiver Grassmannians are projective varieties parametrizing subrepresentations of quiver representations of a fixed dimension vector. The geometry of such projective varieties can be studied via the representation theory of quivers (or of finite dimensional algebras). Quiver Grassmannians appeared in the theory of cluster algebras. As a consequence of the positivity conjecture of Fomin and Zelevinsky, the Euler characteristic of quiver Grassmannians associated with rigid quiver representations must be positive; this fact was proved by Nakajima.
We explore the geometry of quiver Grassmannians associated with rigid quiver representations: we show that they have property (S) meaning that: (1) there is no odd cohomology, (2) the cycle map is an isomorphism, (3) the Chow ring admits explicit generators defined over any field. As a consequence, we deduce that they have polynomial point count. If we restrict to quivers which are of finite or affine type (i.e. orientation of simply-laced extended Dynkin diagrams) we can prove much more: in this case, every quiver Grassmannian associated with an indecomposable representa-tion (not necessarily rigid) admits a cellular decomposition.

ball-Moebius

Friday, May 25th, 2018
h. 15:00 - Room "Roberta Dal Passo"
Ernesto SPINELLI ("Sapienza" Università di Roma)
"Codimension growth and minimal varieties"

Abstract: In characteristic zero an effective way of measuring the polynomial identities satisfied by an algebra is provided by the sequence of its codimensions introduced by Regev. In this talk we review some features of the codimension growth of PI algebras, including the deep contribution of Giambruno and Zaicev on the existence of the PI-exponent, and discuss some recent developments in the framework of group graded algebras. In particular, a characterisation of minimal supervarieties of fixed superexponent will be given. The last result is part of a joint work with O. M. Di Vincenzo and V. da Silva.

Calabi-spin

N.B.: questo seminario si svolge in "Sapienza" Università di Roma
dipartimento di Matematica "Guido Castelnuovo"
Friday, May 4th, 2018
h. 16:30 - Room "Mauro Picone"
Claudia PINZARI ("Sapienza" Università di Roma)
"Weak quasi-Hopf algebras, tensor categories and conformal field theory"

Abstract: In the early nineties, Mack and Schomerus introduced the notion of weak quasi-Hopf algebra as an extension of that of quasi-Hopf algebra of Drinfeld to the case where the coproduct is not unital. The class allows an analogue of twist deformations. We shall discuss various aspects of the theory, including the introduction of a weak analogue of the notion of Hopf algebras in a cohomological interpretation, or the study of C^*-structures with unitary ribbon structure. The special subclass includes the quantum groupoids recently associated to the modular categories of type A.
We use the general theory to construct new structures of tensor C^*-categories on tensor categories from known C^*-structures. Applications include the construction of tensor C^*-categories for the affine vertex operator algebras and builds on Wenzl-Xu and Kazhdan-Lusztig-Finkelberg theory.
The talk is based on an ongoing joint work with Sebastiano Carpi and Sergio Ciamprone.

ball-Moebius

N.B.: questo seminario si svolge in "Sapienza" Università di Roma
dipartimento di Matematica "Guido Castelnuovo"
Friday, May 4th, 2018
h. 15:00 - Room "Mauro Picone"
Claudia MALVENUTO ("Sapienza" Università di Roma)
"Partitions and pictures of posets and finite topologies: a Hopf algebraic viewpoint"

Abstract: In the seminal work of 1972, Richard Stanley gave a generalization to posets of semistandard tableaux associated to diagrams of partitions, and to its generating functions, going from Schur functions and symmetric functions to the world of quasi-symmetric functions. Since then, many different and fundamental Hopf algebra structures were studied in connection to it. Furthermore, the theory of pictures between partition diagrams is known to encode much of the combinatorics of symmetric group representations and related topics: it captures for example the Littlewood-Richardson formula, as already shown by Zelevinsky. In 2011, this framework was extended to double posets (pairs of orders coexisting on a given finite structure).
Recently we worked out a similar approach for finite preorders, which in turn are equivalent to finite topologies, and developed it from the point of view of combinatorial Hopf algebras, leading to new advances in the field.
This is a joint work with Loic Foissy and Frèdèric Patras.

Calabi-spin

Friday, April 13th, 2018
h. 16:00 - Room "Claudio D'Antoni"
René SCHOOF (Università di Roma "Tor Vergata")
"Il teorema di Lagrange per schemi in gruppi piatti e finiti"

Abstract: Il teorema di Lagrange dice che in un gruppo di cardinalità n la potenza n-esima di ogni elemento è uguale all’elemento neutro. Una congettura classica afferma che un risultato simile vale per schemi in gruppi piatti e finiti. Spiegherò la dimostrazione di un caso speciale della congettura.

ball-Moebius

Friday, April 13th, 2018
h. 14:30 - Room "Claudio D'Antoni"
Velleda BALDONI (Università di Roma "Tor Vergata")
"Multiplicities & Kronecker coefficients"

Abstract: Multiplicities of representations appear naturally in different contexts and as such their description could use different languages. The computation of Kronecker coefficients is in particular a very interesting problem which has many applications.
I will describe an approach based on methods from symplectic geometry and residue calculus (joint work with M. Vergne and M. Walter). I will state the general formula for computing Kronecker coefficients and then give many examples computed using an algorithm that implements the formula.
The algorithm does not only compute individual Kronecker coefficients, but also symbolic formulas that are valid on an entire polyhedral chamber. As a byproduct, it is possible to compute several Hilbert series.

Calabi-spin

Friday, March 16th, 2018
h. 15:30 - Room "Claudio D'Antoni"
Kirill ZAYNULLIN (University of Ottawa)
"Equivariant motives and Sheaves on moment graphs"

Abstract: Goresky, Kottwitz and MacPherson showed that the equivariant cohomology of varieties equipped with an action of a torus T can be described using the so called moment graph, hence, translating computations in equivariant cohomology into a combinatorial problem. Braden and MacPherson proved that the information contained in this moment graph is sufficient to compute the equivariant intersection cohomology of the variety. In order to do this, they introduced the notion of a sheaf on moment graph whose space of sections (stalks) describes the (local) intersection cohomology. These results motivated a series of paper by Fiebig, where he developed and axiomatized sheaves of moment graphs theory and exploited Braden-MacPherson's construction to attack representation theoretical problems.
In the talk we explain how to extend this theory of sheaves on moment graphs to an arbitrary algebraic oriented equivariant cohomology h in the sense of Levine-Morel (e.g. to K-theory or algebraic cobordism). Moreover, we show that in the case of a total flag variety X the space of global sections of the respective h-sheaf also describes an endomorphism ring of the equivariant h-motive of X.
This is a very recent joint work with Rostislav Devyatov and Martina Lanini.

ball-Moebius

Friday, March 16th, 2018
h. 14:00 - Room "Claudio D'Antoni"
Kirill ZAYNULLIN (University of Ottawa)
"Equivariant oriented cohomology and generalized Schubert calculus"

Abstract: This lecture can be viewed as an introduction to algebraic oriented cohomology theories (cohomology/Chow groups, K-theory, (local) elliptic cohomology, algebraic cobordism, etc.) and their (mostly T-)equivariant analogues. Our basic motivating example is the algebraic cobordism Ω which was constructed by Levine-Morel around 05's.
This theory serves as an algebraic analogue of the usual complex cobordism from algebraic topology of 60's (similarly, the Chow group serves as an algebraic version of the usual singular cohomology). We explain a general procedure which allows to compute such theories for generalized flag varieties G/P.
The talk is based on my joint results with Calmès, Petrov, Zhong and others.

Calabi-spin

Friday, February 23rd, 2018
h. 15:30 - Room "Claudio D'Antoni"
Laura GEATTI (Università di Roma "Tor Vergata")
"The adapted hyper-Kähler structure on the tangent bundle of a Hermitian symmetric space II"

Abstract: The cotangent bundle of a compact Hermitian symmetric space X = G/K (a tubular neighbourhood of the zero section, in the non-compact case) carries a unique G-invariant hyper-Kähler structure compatible with the Kähler structure of X and the canonical complex symplectic form of T^*X .
The tangent bundle TX, which is isomorphic to T^*X, carries a canonical complex structure J, the so called "adapted complex structure", and admits a unique G-invariant hyper-Kähler structure compatible with the Kähler structure of X and the adapted complex structure J. The two hyper-Kähler structures are related by a G-equivariant fiber preserving diffeomorphism of TX, as already noticed by Dancer and Szöke.
The fact that the domain of existence of J in TX is biholomorphic to a G-invariant domain in the complex homogeneous space G_C/K_C allows us to use Lie theoretical tools and moment map techniques to explicitly compute the various quantities of the "adapted hyper-Kähler structure".
This is part of a joint project with Andrea Iannuzzi, and this talk concludes his presentation of February 9.

ball-Moebius

Friday, February 23rd, 2018
h. 14:00 - Room "Claudio D'Antoni"
Domenico FIORENZA ("Sapienza" Università di Roma)
"T-duality in rational homothopy theory"

Abstract: Sullivan models from rational homotopy theory can be used to describe a duality in string theory. Namely, what in string theory is known as topological T-duality between K⁰-cocycles in type IIA string theory and K¹-cocycles in type IIB string theory, or as Hori's formula, can be recognized as a Fourier-Mukai transform between twisted cohomologies when looked through the lenses of rational homotopy theory. This is an example of topological T-duality in rational homotopy theory, which can be completely formulated in terms of morphisms of L-infinity algebras. Based on joint work with Hisham Sati and Urs Schreiber (arXiv:1712.00758).

Calabi-spin

N.B.: questo seminario si svolge in "Sapienza" Università di Roma - dipartimento di Scienze di Base e Applicate per l'Ingegneria (SBAI)
Friday, February 9th, 2018
h. 15:30 - Room 1B1 (SBAI)
Andrea IANNUZZI (Università di Roma "Tor Vergata")
"Adapted hyper-Kaehler structures I: previous examples and the adapted setting"

Abstract: In this first talk, we wish to recall basic facts and classical constructions of invariant hyper-Kaehler structures on the cotangent bundle T^*X of a Hermitian symmetric space X=G/K due to Eguchi-Hanson, Calabi, Biquard, Gauduchon, Feix, Kaledin.
On (a tubolar neighborhood of) the tangent bundle TX ~ T^*X one also has the adapted complex structure J which makes it biholomorphic to the (crown domain in the) complex homogeneous space G_C/K_C. By letting J replace the role of the holomorphic symplectic form on T^*X, one obtains the unique adapted hyper-Kaehler structure associated to G/K . In this context, the interplay of complex geometry and the Lie group structure of G_C leads to an explicit realization of all the terms of such a structure.
Time permitting, we compare the adapted context with the previous constructions. This is part of a joint project with Laura Geatti. More details on the adapted realization in the second talk.

ball-Moebius

N.B.: questo seminario si svolge in "Sapienza" Università di Roma - dipartimento di Scienze di Base e Applicate per l'Ingegneria (SBAI)
Friday, February 9th, 2018
h. 14:00 - Room 1B1 (SBAI)
Roberto CONTI ("Sapienza" Università di Roma)
"Le algebre di Cuntz e i loro automorfismi"

Abstract: Le C^*-algebre (non unitali) rappresentano spazi topologici (localmente) compatti possibilmente noncommutativi e i loro automorfismi giocano quindi lo stesso ruolo degli omeomorfismi in ambito classico. Esistono molte costruzioni di C^*-algebre con proprietà specifiche, che possano risultare utili nei diversi contesti. Negli anni settanta, Doplicher e Roberts utilizzarono sistematicamente multipletti di n isometrie con somma dei proiettori finali uguali all'identità per implementare morfismi localizzati in teoria algebrica dei campi. Le C^*-algebre O_n generate da tali famiglie di n isometrie sono oggi note come algebre di Cuntz e sono uno degli oggetti più studiati nell'ambito delle algebre di operatori. Nel seminario introdurremo tali C^*-algebre e, dopo averne ricordato alcune proprietà e applicazioni, ci soffermeremo su alcune recenti tematiche che emergono nello studio dei loro gruppi di automorfismi.

Calabi-spin

Friday, January 12th, 2018
h. 15:30 - Room "Claudio D'Antoni"
Ilaria DAMIANI (Università di Roma "Tor Vergata")
"On the Drinfeld coproduct"

Abstract: In an unpublished note Drinfeld defined a new ``coproduct'' on the affine quantum algebras U_q (different from the Drinfeld-Jimbo one), which actually takes values in a completion of the tensor product of U_q x U_q . In the simply laced case it is not too hard to prove that the relations defining U_q are preserved by the Drinfeld coproduct (which is then a well defined algebra homomorphism); but in the other cases the expression of the Drinfeld coproduct applied to the Serre relations is very complicated, and till now the direct attempts to prove that it is zero failed. In this talk a different strategy is presented: the bracket by the Drinfeld generators is deformed so to get a locally nilpotent derivation D on a suitable algebra V; the study of the exponential of D, which is an algebra automorphism of D, provides a proof that the Drinfeld coproduct is well defined.
This construction works for both the affine quantum algebras and the toroidal quantum algebras.

ball-Moebius

Friday, January 12th, 2018
h. 14:00 - Room "Claudio D'Antoni"
Alberto DE SOLE ("Sapienza" Università di Roma)
"W-algebre: dall'identità di Capelli generalizzata alle gerarchie KdV generalizzate"

Abstract: Descriverò la costruzione di un operatore di tipo Lax L(z) associato sia alle W-algebre W(g,f) quantistiche finite, sia a quelle classiche affini. Per g = gl_N , tale operatore si costruisce, nel caso quantistico finito, tramite una formula che generalizza l'identità di Capelli. Nel caso classico affine, invece, l'operatore di Lax L(z) può essere utilizzato per costruire una gerarchia integrabile di equazioni alle derivate parziali che generalizza la gerarchia KdV.
I risultati presentati sono frutto di un lavoro in collaborazione con V. Kac e D. Valeri.

rotating-knot

Friday, December 1st, 2017
h. 15:30 - Room "Roberta Dal Passo"
Fabio GAVARINI (Università di Roma "Tor Vergata")
"Supergroups vs. super Harish-Chandra pairs: a new equivalence"

Abstract: In the setup of supergeometry, "symmetries" are encoded as supergroups (algebraic or Lie ones), whose infinitesimal counterpart is given by Lie superalgebras. Moreover, every supergroup also bears a "classical (=non-super) content", in the form of a maximal classical subgroup. Thus every supergroup has an associated pair given by its tangent Lie superalgebra and its maximal classical subgroup - what is called a "super Harish-Chandra pair" (or "sHCp" in short): overall, this yields a functor F from supergroups to sHCp's.
It is known that the functor F is an equivalence of categories: indeed, this was showed by providing an explicit quasi-inverse functor, say G, to F. Koszul first devised G for the real Lie case, then later on several other authors extended his recipe to more general cases.
In this talk I shall present a new functorial method to associate a Lie supergroup with a given sHCp: this gives a functor K from sHCp's to supergroups which happens to be a quasi-inverse to F, that is intrinsically different from G.
In spite of different technicalities, the spine of the method for constructing the functor K is the same regardless of the kind of supergeometry (i.e., algebraic, real differential or complex analytic one) we are dealing with, so I shall treat all cases at once.

Calabi-spin

Friday, December 1st, 2017
h. 14:00 - Room "Roberta Dal Passo"
Alessandro D'ANDREA ("Sapienza" Università di Roma)
"Dynamical systems on graphs and Hecke-Kiselman monoids"

Abstract: A Coxeter monoid is generated by idempotents satisfying the usual braid relations found in the presentation of Coxeter groups. Kiselman's semigroups are certain monoids, originally introduced in the context of convexity theory. Hecke-Kiselman monoids provide a generalization of both concepts. I will first address the finiteness problem for Hecke-Kiselman monoids, and then give a combinatorial description of Kiselman's semigroups - and possibly some of its quotients - by considering all possible evolutions of some special dynamical systems on a graph, called "update systems".

ball-Moebius

Friday, November 17th, 2017
h. 14:30 - Room 1201 "Roberta Dal Passo"
Michael EHRIG (University of Sidney)
"Functoriality of link homologies and higher representation theory"

Abstract: In this talk, we will discuss the notion of functoriality of link homologies defined by Khovanov and Khovanov-Rozansky. These link homologies are categorifications of the link invariants defined by Reshetikhin-Turaev in case of the special linear group. We will discuss why functoriality is an important notion and how to show it. The latter will include the equivariant geometry of Grassmannians and partial flag varieties as well as higher representation theory.

Calabi-spin

Tuesday, September 26th, 2017
h. 15:00 - Room "Claudio D'Antoni"
Lara BOSSINGER (University of Cologne)
"Toric degenerations of Grassmannians: birational sequences and the tropical variety"

Abstract: As toric varieties are well understood due to their rich combinatorial structure, a toric degeneration allows to deduce properties of the original variety. For Grassmannians, such degenerations can be obtained from birational sequences and the tropical Grassmannian.
The first were recently introduced by Fang, Fourier, and Littelmann. They originate from the representation theory of Lie algebras and algebraic groups. In our case, we use a sequence of positive roots for the Lie algebra sl_n to define a valuation on the homogeneous coordinate ring of the Grassmannian. Nice properties of this valuation allow us to define a filtration whose associated graded algebra (if finitely generated) is the homogeneous coordinate ring of the toric variety.
The second was defined by Speyer and Sturmfels and is an example of a tropical variety: a discrete object (a fan) associated to the original variety that shares some of its properties and in nice cases, as the one of Grassmannians, provides toric degenerations. In this talk, I will briefly explain the two approaches and establish a connection between them.

ball-Moebius

Monday, June 12th, 2017
h. 16:30 - Room "Roberta Dal Passo"
Paolo PAPI ("Sapienza" Università di Roma)
"Conformal embeddings"

Abstract: Introdurrò la nozione di conformal embedding partendo dalla definizione originaria in termini di algebre affini per poi discutere la nozione a livello di algebre di vertice. Illustrerò la classificazione dei conformal embeddings massimali associati a algebre di vertice affini ottenuta in collaborazione con Adamovic, Kac, Moseneder, Perse.

Calabi-spin

Monday, June 12th, 2017
h. 15:00 - Room "Roberta Dal Passo"
Francesco BRENTI (Università di Roma "Tor Vergata")
"Punti fissi e salite adiacenti in gruppi classici complessi di riflessioni"

Abstract: In [Adv. Applied. Math., 2014] Diaconis, Evans e Graham dimostrano che il numero di permutazioni di S_n che hanno salite adiacenti in un certo insieme I è uguale al numero di permutazioni che hanno punti fissi minori di n in I. In questo seminario investigherò quanto questo risultato può essere raffinato ed esteso ai gruppi classici complessi di riflessioni. Questo lavoro è in collaborazione con M. Marietti.

ball-Moebius

N.B.: questo seminario si svolge in "Sapienza" Università di Roma - dipartimento di Matematica "Guido Castelnuovo"
Monday, May 29th, 2017
h. 16:30 - Room "Mauro Picone"
Luca GIORGETTI (Università di Roma "Tor Vergata")
"Modular Tensor Categories in Conformal Field Theory and their classification problem"

Abstract: MTCs are finite braided tensor categories where the braiding is non-degenerate, i.e., maximally non-symmetric. They owe their name to the well-known fact that this non-degeneracy is actually equivalent to the appearance of representations of the modular group PSL(2,Z) by means of certain canonically defined matrices S, T (also called modular data). I will explain their relevance in physics (more precisely, in models of chiral CFT) and comment on a trace formula for self-braidings by means of modular data, which can be used in the classification problem of MTCs.
References: http://web.math.ucsb.edu/~zhenghwa/data/course/cbms.pdf , https://arxiv.org/pdf/1201.6593.pdf , https://arxiv.org/abs/1305.2229 , http://arxiv.org/abs/1606.04378v1

Calabi-spin

N.B.: questo seminario si svolge in "Sapienza" Università di Roma - dipartimento di Matematica "Guido Castelnuovo"
Monday, May 29th, 2017
h. 15:00 - Room "Mauro Picone"
Mario MARIETTI (Università Politecnica delle Marche)
"The Combinatorial Invariance Conjecture for parabolic Kazhdan-Lusztig polynomials"

Abstract: The Dyer-Lusztig Combinatorial Invariance Conjecture states that a Kazhdan-Lusztig polynomial is determined by the underlying poset structure. We discuss the problem of combinatorial invariance in the parabolic setting.

ball-Moebius

Wednesday, May 17th, 2017
h. 13:00 - Room "Claudio D'Antoni"
Simona SETTEPANELLA (Hokkaido University)
"Intersection lattice of Discriminantal arrangement and hypersurfaces in Grassmannian"

Abstract: In 1989 Manin and Schechtman considered a family of arrangements of hyperplanes generalizing classical braid arrangements which they called the Discriminantal arrangements. Such an arrangement consists of parallel translates of collection of n hyperplanes in general position in C^k which fail to form a generic arrangement in C^k. In 1994 Falk showed that the combinatorial type of Discriminantal arrangement depends on the collection of n hyperplanes in general position in C^k.
In 1997 Bayer and Brandt divided generic arrangements in C^k in "very generic" and "non very generic" depending of the intersection lattice of associated Discriminatal arrangement. In 1999 Athanasiadis provided a full description of intersection lattice for Discriminantal arrangement in the very generic case. More recently, in 2016, Libgober and Settepanella gave a description of rank 2 intersection lattice of Discriminantal arrangement in non very generic case, providing a sufficient geometric condition for a generic arrangement in C^k to be non very generic. In this talk we will recall their result and we will show that non very generic arrangements in C³ satisfying their condition correspond to points in a degree 2 hypersurface in the complex Grassmannian Gr(3,n). This is a joint work with S. Sawada and S. Yamagata.

Calabi-spin

N.B.: questo seminario si svolge in "Sapienza" Università di Roma - dipartimento di Matematica "Guido Castelnuovo"
Monday, May 15th, 2017
h. 16:30 - Room "Claudio D'Antoni"
Giovanni CERULLI IRELLI ("Sapienza" Università di Roma)
"The cluster multiplication formula"

Abstract: In 2005, Caldero and Chapoton introduced a formula that provide an intrinsic description of the cluster variables of cluster algebras associated with Dynkin quivers. The key to prove the formula was a cluster multiplication formula. This formula was extended to acyclic quivers by Caldero and Keller in 2006 and further generalized by Hubery and independently by Fan Xu in 2010. In a more general context the multiplication formula was proved to hold by Yann Palu in his PhD thesis in 2009. In this talk I will revise the history of this formula and provide a new proof which seems much more simple than the previous ones. This is part of a joint project with F. Esposito, H. Franzen and M. Reineke.
Seguendo un lungo programma di classificazione iniziato da Luna nel 2001, le varietà sferiche omogenee sono state classificate combinatoricamente mediante notevoli oggetti combinatorici che possono essere pensati come generalizzazioni di sistemi di radici, tuttavia continua a sapersi abbastanza poco sui relativi insiemi delle B-orbite. Oltre al naturale ordinamento, l'insieme delle B-orbite di una varietà sferica presenta una ricca struttura combinatorica: un'azione del gruppo di Weyl su di esso è stata definita da Knop. Dopo aver discusso i problemi generali, introdurrò alcune famiglie di esempi dove la classificazione delle B-orbite può essere presentata in modo puramente combinatorico, mediante opportuni sistemi di radici che si comportano molto bene relativamente all'azione del gruppo di Weyl.

ball-Moebius

N.B.: questo seminario si svolge in "Sapienza" Università di Roma - dipartimento di Matematica "Guido Castelnuovo"
Monday, May 15th, 2017
h. 15:00 - Room "Claudio D'Antoni"
Guido PEZZINI ("Sapienza" Università di Roma)
"Varietà simmetriche per gruppi di Kac-Moody"

Abstract: Le varietà simmetriche sono varietà di notevole importanza nella teoria dei gruppi algebrici, hanno legami ed applicazioni fondamentali in vari campi quali geometria Riemanniana, analisi armonica, e teoria delle rappresentazioni. Nel seminario ricorderemo alcune proprietà di queste varietà, nell'ottica della teoria delle varietà sferiche. Inoltre discuteremo di una possibile generalizzazione infinito-dimensionale, per gruppi di Kac-Moody. Il seminario si basa su una collaborazione con Bart Van Steirteghem.

Calabi-spin

Friday, May 5th, 2017
h. 16:00 - Room "Claudio D'Antoni"
Jacopo GANDINI (Scuola Normale Superiore - Pisa)
"Sull'insieme delle orbite di un gruppo di Borel in una varietà sferica"

Abstract: Sia G/H uno spazio omogeneo per un gruppo algebrico riduttivo G. Tale G/H è detto varietà sferica (omogenea) se si decompone in un numero finito di orbite sotto l'azione di un sottogruppo di Borel B di G. L'esempio più semplice di questa situazione è quello della varietà delle bandiere G/B , il cui insieme delle B-orbite è in corrispondenza biunivoca con il gruppo di Weyl di G. Un'altro caso particolare di questa situazione che è stato molto studiato è quello delle varietà simmetriche (vale a dire quando H è l'insieme dei punti fissi di un'involuzione di G), nel qual caso Richardson e Springer hanno sviluppato un notevole apparato combinatorico per lo studio dell'insieme delle B-orbite e del relativo ordinamento dato dall'inclusione delle chiusure.
Seguendo un lungo programma di classificazione iniziato da Luna nel 2001, le varietà sferiche omogenee sono state classificate combinatoricamente mediante notevoli oggetti combinatorici che possono essere pensati come generalizzazioni di sistemi di radici, tuttavia continua a sapersi abbastanza poco sui relativi insiemi delle B-orbite. Oltre al naturale ordinamento, l'insieme delle B-orbite di una varietà sferica presenta una ricca struttura combinatorica: un'azione del gruppo di Weyl su di esso è stata definita da Knop. Dopo aver discusso i problemi generali, introdurrò alcune famiglie di esempi dove la classificazione delle B-orbite può essere presentata in modo puramente combinatorico, mediante opportuni sistemi di radici che si comportano molto bene relativamente all'azione del gruppo di Weyl.

ball-Moebius

Friday, May 5th, 2017
h. 14:30 - Room "Claudio D'Antoni"
Paolo SALVATORE (Università di Roma "Tor Vergata")
"Formalità in algebra, topologia e geometria"

Abstract: Una struttura algebrica differenziale (A,d) si dice formale se è equivalente nella categoria derivata alla sua coomologia (H(A),0). Questo ha senso per esempio per algebre associative, commutative, operads. Farò una panoramica di risultati di (non)-formalità, e loro significato, per strutture provenienti dalla topologia e dalla geometria, dal teorema di Deligne-Griffiths-Morgan-Sullivan, al lavoro di Kontsevich e altri sugli operad dei piccoli dischi, fino ai risultati recenti di Horel e Fresse-Turchin-Willwacher.

Calabi-spin

Friday, April 21st, 2017
h. 16:30 - Room "Claudio D'Antoni"
Niels KOWALZIG ("Sapienza" Università di Roma)
"Higher Structures and Cyclic Operads"

Abstract: We will discuss the notion of noncommutative differential calculus introduced by Nest, Tamarkin, and Tsygan, which in particular asks for a very general algebraic notion of Lie derivative and cap product, along with a cyclic differential in the sense of Connes and the structure of a Gerstenhaber algebra. We then dedicate our attention to so-called opposite modules over operads with multiplication (in k-mod) and the ingredients required to obtain on them the structure of a cyclic module and how the underlying simplicial homology gives rise to a Batalin-Vilkovisky module over the cohomology of the operad. Examples include the well-known calculi from differential geometry and in algebra on Hochschild cohomology groups. An interesting application consists in considering a cyclic operad as an opposite module over itself by which one obtains a homotopy formula for the bracket of a Batalin-Vilkovisky algebra, which allows to re-prove Menichi's result on Batalin-Vilkovisky algebra structures on the simplicial cohomology of a cyclic operad with multiplication.

ball-Moebius

Friday, April 21st, 2017
h. 15:00 - Room "Claudio D'Antoni"
Yoh TANIMOTO (Università di Roma "Tor Vergata")
"Rappresentazioni ad energia positiva dei loop group e net conformi"

Abstract: Un loop group è il gruppo delle mappe dal cerchio S¹ ad un gruppo (di Lie, compatto) con l'operazione punto per punto. Su esso agisce il cerchio in un modo naturale e si può considerare una classe delle rappresentazioni proiettive dove l'azione del cerchio ha uno spettro positivo, che si comportano una maniera analoga a quella del gruppo compatto. Tra tali rappresentazioni ce ne sono alcune il cui vettore ad energia minima è unico, il vuoto. Ad una rappresentazione col vuoto si può associare un net di algebre di von Neumann, un oggetto assiomatico per la teoria quantistica dei campi, e si possono considerare le loro rappresentazioni. Si dimostra che c'è una corrispondenza uno a uno tra le rappresentazioni ad energia positiva (ad un livello fisso) e le rappresentazioni del net. Spiego alcune nozioni che si possono definire o calcolare in una parte ma sono difficili nell'altra, per esempio dimensioni, fusioni e rappresentazioni indotte.

Calabi-spin

Tuesday, April 11th, 2017
h. 14:30 - Room "Claudio D'Antoni"
Salvatore STELLA (Sapienza - Università di Roma)
"Gruppi di Kac-Moody, minori generalizzati e rappresentazioni di quiver"

Abstract: La teoria delle rappresentazioni dei gruppi di Kac-Moody e quella dei quiver aciclici presentano entrambe, nel caso generale, una struttura tripartita. Le rappresentazioni di un gruppo di Kac-Moody G sono divise naturalmente in tre classi (peso più alto, peso più basso e livello zero) a seconda di come il centro di G agisce. Le rappresentazioni indecomponibili di un quiver Q sono preproiettive, postiniettive o regolari a seconda di dove sono collocate nel quiver di Auslander-Reiten associato a Q.
In questo seminario illustreremo un modo per collegare queste due tripartizioni. Identificando l'anello delle funzioni regolari su un'opportuna cella doppia di Bruhat di G con un'algebra cluster mostreremo che le variabili cluster che vengono da Q-moduli preproiettivi (rispettivamente postiniettivi o regolari) possono essere interpretate come minori generalizzati associati a rappresentazioni di peso più alto (rispettivamente peso più basso o livello zero) di G.
Non assumeremo nessuna conoscenza delle algebre cluster e solo minime nozioni di teoria delle rappresentazioni.

ball-Moebius

Monday, April 10th, 2017
h. 14:00 - Room "Claudio D'Antoni"
Andrea APPEL (University of South California - Los Angeles)
"Monodromia della connessione di Casimir e categorie di Coxeter"

Abstract: Una categoria di Coxeter (o di quasi-Coxeter) è una categoria tensoriale intrecciata sui cui oggetti è data un'azione naturale di un gruppo di trecce generalizzato B_W. L'assiomatica corrispondente è molto simile a quella delle categorie tensoriali intrecciate con analoghi vincoli di associatività e commutatività atti però a descrivere una proprietà di coerenza di una famiglia di funtori di restrizione. Mostreremo quindi come costruire due esempi di tale struttura sulla categoria O (integrabile) di un'algebra di Kac-Moody simmetrizzabile g: la prima è indotta dal corrispondente gruppo quantico U_h(g); la seconda è costruita a partire dalla monodromia delle corrispondenti connessioni KZ e Casimir di g. Grazie a un teorema di rigidità - dimostrato nell'ambito di categorie PROP - tale struttura sulla categoria O risulta unica (a meno di equivalenza). Da ciò segue in particolare che la monodromia della connessione di Casimir è descritta dagli operatori del gruppo di Weyl quantico di U_h(g).
Questo lavoro è in collaborazione con Valerio Toledano Laredo.

rotating-knot

Escher-birds

Colloquium di Dipartimento
Wednesday, November 11th, 2016
h. 15:00 - Room "Roberta Dal Passo"
Valerio TOLEDANO LAREDO
(Northeastern University - Boston)

Escher-birds

"Differential equations and quantum groups"

Abstract: Quantum groups were introduced in the mid-eighties by Drinfeld and Jimbo as the algebraic backbone of the quantum Inverse Scattering Method of Statistical Mechanics. They were soon found to have a host of other applications: to low-dimension topology, representation theory, and algebraic geometry to name a few. This talk will concentrate on one aspect of quantum groups, namely their uncanny ability to describe the monodromy of integrable systems of partial differential equations attached to semisimple Lie algebras. This phenomenon was originally discovered by Drinfeld and Kohno in the early 90s in connection with the Knizhnik-Zamolodchikov equations of Conformal Theory. More recently, I proved that quantum groups also describe the monodromy of the so-called Casimir equations of a semisimple Lie algebra and, in recent joint work with Andrea Appel (USC), that this continues to hold for any symmetrisable Kac-Moody algebra.

rotating-knot

N.B.: questo seminario si svolge in "Sapienza" Università di Roma - dipartimento di Matematica "Guido Castelnuovo"
Tuesday, June 24th, 2015
h. 15:00 - Aula di Consiglio
Fabio GAVARINI (Università di Roma "Tor Vergata")
"Affine supergroups and super Harish-Chandra pairs"

Abstract: Together with any supergroup, one can naturally associate the pair made of its classical (i.e. non super) underlying group and its tangent Lie superalgebra, two objects which obey some obvious mutual compatibility constraints; any similar pair is called "super Harish-Chandra pair" (=sHCp). This construction leading from supergroups to sHCp's is functorial, and actually an equivalence, as an explicit quasi-inverse functor is known.
In this talk I present a new, totally different recipe for such a quasi-inverse: indeed, it extends to a much larger setup, with a more geometrical method. I shall mainly adopt the point of view of algebraic (super)geometry, but the bunch of ideas and results we shall be dealing with actually applies to the real differential, the real analytic and the complex analytic case as well.
Reference: F. Gavarini, "Global splittings and super Harish-Chandra pairs for affine supergroups", Transactions of the American Mathematical Society (to appear), 56 pages – see http://arxiv.org/abs/1308.0462

rotating-knot

Thursday, June 19th, 2014
h. 11:00 - Room "Roberta Dal Passo"
Jerzy WEYMAN (University of Connecticut)
"Local Cohomology supported in determinantal varieties - II"

Abstract: This is a mini-course on local cohomology supported in determinantal varieties. In this second lecture, two recent papers by C. Raicu and E. Witt will be discussed.

Calabi-spin

Wednesday, June 18th, 2014
h. 11:00 - Room "Roberta Dal Passo"
Nicola SAMBIN (University of Connecticut)
"Semi-invariants for tame algebras"

Abstract: This is the discussion of the speaker's Ph. D. Thesis.

ball-Moebius

Monday, June 16th, 2014
h. 11:00 - Room "Roberta Dal Passo"
Jerzy WEYMAN (University of Connecticut)
"Local Cohomology supported in determinantal varieties - I"

Abstract: This is a mini-course on local cohomology supported in determinantal varieties. In this first lecture the basic notions will be introduced.

rotating-knot

Thursday, September 26th, 2013
h. 13:00 - Room "Roberta Dal Passo"
Stephen DONKIN (York)
"Resolutions of Quantised Weyl Modules"

Abstract: This is joint work with Ana Paula Santana and Ivan Yudin (Coimbra). In a recent paper Santana and Yudin proved a conjecture of Boltje and Hartmann on the exactness of a certain complexes of modules for the symmetric groups. This was done by first producing a resolution of Weyl modules of general linear groups and then applying the Schur functor. Still more recently analogous complexes of modules for the Hecke algebra of type A appeared in a paper by Boltje and Maisch. We adopt the same approach as in the classical case to show that these too are exact. In order to do this we develop some homological algebra of quantum general linear groups.

Calabi-spin

Monday, September 23rd, 2013
h. 15:00 - Room "Claudio D'Antoni"
Marc ROSSO (Paris 7)
"Around multiparametric quantum groups"

Abstract: Besides the familiar quantized enveloping algebras (depending on a parameter q), differents kinds of multiparametric versions were constructed by several authors, from various motivations: via twisting of quasi-triangular Hopf algebras, via Hopf 2-cocycles deformations, from the point of view quantized shuffle algebras on a braided vector space of diagonal type. They also have some interesting relations with multiparametric commutation relations, non commutative symmetric functions, etc.
This talk will present an overview of the subject.

ball-Moebius

Thursday, September 19th, 2013
h. 11:00 - Room "Roberta Dal Passo"
Stephen DONKIN (York)
"On the calculation of the cohomology of line bundles on flag varieties in characteristic p - IV"

Abstract: Let G be a reductive algebraic group (for example a special linear group) and let B be a Borel subgroup (i.e., a maximal closed connected solvable subgroup). The representation theory of G is closely related to the algebraic geometry of the quotient space G/B. In characteristic 0 this connection is succinctly summarized by the Borel-Bott-Weil Theorem. This shows in particular that the cohomology of a line bundle is non-zero in at most one degree and its character is either a Weyl character or 0. In characteristic p the connection between the representation theory and geometry still exists and has been extremely useful (in work by Haboush, Andersen, Jantzen and others). Nevertheless there is no known analogue of the Borel-Bott-Weil Theorem. Earlier work (e.g. that of Andersen and Humphreys) has focused on the module structure of the cohomology spaces. However, concentrating only on the character one can get complete information in some low rank cases using infinitesimal methods. So far the cases worked out completely are G = SL(2) (classical), G = SL(3) (Donkin), and G = Sp(4) in characteristic 2 (Donkin and Geranios). We describe an approach to this problem using infinitesimal methods and how they may be used to give recursive formulas for the characters of the cohomology of line bundles in favorable circumstances.

Calabi-spin

Tuesday, September 17th, 2013
h. 11:00 - Room "Roberta Dal Passo"
Stephen DONKIN (York)
"On the calculation of the cohomology of line bundles on flag varieties in characteristic p - III"

Thursday, February 28th, 2013
h. 15:00 - Room "Francesco De Blasi"
Gastón Andrés GARCÍA
(FaMAF-CIEM / Universidad Nacional de Córdoba)
"Quantum groups and Hopf algebras"

Abstract: We will introduce the notion of quantum group and we will show its relation with the classification problem of finite-dimensional Hopf algebras over an algebraically closed field of characteristic zero.
Quantum groups, introduced in 1986 by Drinfeld, form a certain class of Hopf algebras. They can be presented as deformations in one or more parameters of associative algebras related to semisimple (reductive) linear algebraic groups or semisimple (reductive) Lie algebras.
One of the main open problems in the theory of Hopf algebras is the classification of finite-dimensional Hopf algebras over an algebraically closed field of characteristic zero. The first obstruction in solving the classification is the lack of enough examples. Hence, it is necessary to find new families of Hopf algebras. From the beginning, this role was played by the quantum groups. They consists of a large family with different structural properties and were used with profit to solve the classification problem for fixed dimensions.
After introducing quantum groups and Hopf algebras, we will give some basic examples and we will study properties that characterize the known quantum groups. Finally, we will show how they get into the scene of the classification problem.

Calabi-spin

Thursday, February 14th, 2013
h. 15:00 - Room "Claudio D'Antoni"
Ulrich KRÄHMER (Glasgow)
"On the Dolbeault-Dirac operator of a quantised Hermitian symmetric space"

Abstract: In this joint work with Matthew Tucker-Simmons (Berkeley) the cobar-complex of the quantised compact Hermitian symmetric spaces is identified with the Koszul complexes of the quantised symmetric algebras of Berenstein and Zwicknagl. This leads for example to an explicit construction of the relevant quantised Clifford algebras.
The talk will be fairly self-contained and begin with three micro courses covering the necessary classical background (one on Dirac operators, one on symmetric spaces, one on Koszul algebras), and then I’ll explain how noncommutative geometry and quantum group theory lead to the problems that we are dealing with in this project.

ball-Moebius

Tuesday, January 29th, 2013
h. 15:30 - Room "Claudio D'Antoni"
Laiachi EL KAOUTIT (Granada - Spagna)
"The universal Picard-Vessiot ring, differential operators,
jet spaces and completed Hopf algebroids"

Abstract: In this talk we will see how the affine scheme Spec(V) represented by the "universal Picard-Vessiot ring" V of the affine line A¹_C over the complex numbers admits the structure of an affine algebraic groupoid, or, equivalently, that V is a commutative Hopf algebroid over the coordinate ring C[x]. Each affine algebraic Galois group attached to a matrix linear differential equation can then be identified with a closed subset of some affine algebraic groupoid containing an image of Spec(V). Moreover, the category of all differential modules is recognized as the Cauchy category of (right) comodules over V.
This will be a specialization of a general result which we will show and that deals with a certain duality between commutative Hopf algebroids and cocommuative (right) Hopf algebroids where source and target are equal. As we will see, in the case of the affine line this duality is strongly related to the well-known duality between differential operators and jet spaces. The general case, where one considers the jet bundle of a given Lie algebroid, is also analysed and where the notion of completed commutative Hopf algebroids makes its appearance.
(part of the results were obtained in collaboration with José Gómez-Torrecillas)

rotating-knot

Thursday, June 14th, 2012
h. 15:00 - Room "Roberta Dal Passo"
Niels KOWALZIG (INdAM-Tor Vergata)
"Twisted Cyclic (Co)Homology of Hopf Algebroids"

Abstract: The notions of Hopf algebroids and their cyclic (co)homology, Hopf-cyclic (co)homology, incorporate concepts of generalised symmetries in noncommutative geometry (i.e., the noncommutative analogue of groupoids and Lie algebroids) and their associated (co)homologies. We discuss the cyclic cohomology for left Hopf algebroids (also known as "×A-Hopf algebras") with coefficients in a right module-left comodule which is not necessarily stable anti Yetter-Drinfel'd, and we explain how this fits into the monoidal category of (Hopf algebroid) modules showing that it descends in a canonical way from the cyclic cohomology of corings. A generalization of cyclic duality that makes sense for arbitrary paracyclic objects yields a dual homology theory. We then give a few new examples of (left) Hopf algebroids such as universal enveloping algebras of Lie-Rinehart algebras (Lie algebroids), jet spaces, and convolution algebras over étale groupoids. By computing their respective cyclic theory, we establish Hopf-cyclic (co)homology as a noncommutative extension of both Lie algebroid (co)homology and groupoid homology. In particular, both Hochschild and Poisson (co)homology, crucial ingredients in, for example, deformation quantisation, are covered by this theory.

Calabi-spin

Wednesday, June 13th, 2012
h. 14:00 - Room "Roberta Dal Passo"
Cristina DI TRAPANO (Roma "Tor Vergata")
"Semi-invariants of Artin algebras"

Abstract: Let K be an algebraically closed field with characteristic 0 and let (Q,R) be a bound quiver without oriented cycles of finite type. Then we shall show that the ring of semi-invariants on a faithful irreducible component of the variety of all representations of (Q,R) of a given dimension vector is a semigroup ring. We shall describe a complete set of generators and relations of such ring. Moreover, we shall see an application to the class of tilted algebras of type A_n.

ball-Moebius

Tuesday, April 24th, 2012
h. 11:45-14:00 - Room "Roberta Dal Passo"
Stephen DONKIN (York)
"Representations of the hyperalgebra of a semisimple group - III"

Abstract: The point is to develop the representation theory of the hyperalgebra of a semisimple group (in characteristic p). To get the hyperalgebra you take the enveloping algebra of a complex Lie algebra, take the Kostant Z-form and tensor with a field of characteristic p. The point is that this is a very nice algebra (in particular a Hopf algebra) and one can develop its representation theory "from scratch". At some point one proves that the category of finite dimensional modules is the same as the category of modules for the algebraic group with the same root system. So this gives a way in to the representation theory of algebraic groups that does not require any algebraic geometry.
(N.B.: this is the third and last part of a three-sessions seminar)

Calabi-spin

Thursday, April 19th, 2012
h. 14:00-17:30 - Room "Roberta Dal Passo"
Stephen DONKIN (York)
"Representations of the hyperalgebra of a semisimple group - II"

Abstract: The point is to develop the representation theory of the hyperalgebra of a semisimple group (in characteristic p). To get the hyperalgebra you take the enveloping algebra of a complex Lie algebra, take the Kostant Z-form and tensor with a field of characteristic p. The point is that this is a very nice algebra (in particular a Hopf algebra) and one can develop its representation theory "from scratch". At some point one proves that the category of finite dimensional modules is the same as the category of modules for the algebraic group with the same root system. So this gives a way in to the representation theory of algebraic groups that does not require any algebraic geometry.
(N.B.: this is the second part of a three-sessions seminar)

ball-Moebius

Tuesday, April 17th, 2012
h. 11:45-14:00 - Room "Roberta Dal Passo"
Stephen DONKIN (York)
"Representations of the hyperalgebra of a semisimple group - I"

rotating-knot

Escher-birds

Colloquium di Dipartimento
Monday, January 17th, 2011
h. 15:00 - Room 2001
Earl J. TAFT (Rutgers)

"Polynomially recursive sequences and combinatorial identities"

Abstract: A polynomially recursive sequence satisfies a recursive relation with variable coefficients. The set of these sequences has the structure of a topological bialgebra. If such a sequence is of a combinatorial nature, a formula for its coproduct can be (upon appropriate evaluation) be interpreted as a combinatorial identity. Here we give a coproduct formula for each sequence of the form "binomial-coefficient-times-factorial", and its interpretation as a combinatorial identity. We also obtain a q-version of this coproduct formula and combinatorial identity

rotating-knot

Thursday, June 10th, 2010
h. 11:00 - Room 1103
Stephanie CUPIT-FOUTOU (Colonia)
"A geometrical realization of wonderful varieties - II"

Abstract: After introducing wonderful varieties and their combinatorial invariants, I will explain Luna's conjecture about the classification of these varieties. On the other hand, I shall recall definition and main results on invariant Hilbert schemes due to Alexeev and Brion. Then I will explain how I can answer positively Luna's conjecture by means of invariant Hilbert schemes.
(N.B.: this the second and last part of a two-sessions talk)

Calabi-spin

Wednesday, June 9th, 2010
h. 14:00 - Room "Roberta Dal Passo"
Stephanie CUPIT-FOUTOU (Colonia)
"A geometrical realization of wonderful varieties - I"

Escher-birds

Colloquium di Dipartimento
Tuesday, June 8th, 2010
h. 14:00 - Room "Roberta Dal Passo"
Enrico BOMBIERI (IAS, Princeton)

"Zeta and L-functions yesterday and tomorrow"

Abstract: This lecture is a panorama of zeta and L-functions. The Riemann, Dirichlet, Dedekind zeta functions, and Hecke L-functions are introduced. This is followed by the second way of introducing L-functions, via Galois extensions and Artin L-functions. This is followed by the third way of defining L-functions, via automorphic functions. The interrelation of these three classes leads to the modern theory with basic conjectures and the Langlands program. The geometric point of view is also given, with zeta functions of varieties over finite fields, with an outline of Weil's proof of the Riemann hypothesis for curves over a finite field. The lecture concludes with the modern conjectures and developments and about the analytic theory of zeta and L-functions and open problems.

Calabi-spin

Monday, May 24th, 2010
h. 17:30 - Istituto Nazionale di Alta Matematica
Workshop "Incontro Nazionale di Algebra Moderna"
Velleda BALDONI (Roma "Tor Vergata")
"Funzioni di partizione e politopi: esempi ed applicazioni"

Monday, May 3rd, 2010
h. 14:00 - Room "Roberta Dal Passo"
David BUCHSBAUM (Brandeis)
"Some open problems in representation theory and commutative algebra from the homological point of view"

Wednesday, March 17th, 2010
h. 15:00 - Room "Roberta Dal Passo"
Petter BRANDEN (KTH Stoccolma)
"Multivariate stable polynomials and the monotone column permanent conjecture"

Abstract: Joint with Borcea we have recently developed a theory around multivariate stable polynomials, i.e. polynomials that are nonvanishing whenever all variables are in the open upper half-plane. The monotone column permanent conjecture (MCPC) asserts that certain univariate polynomials arising from permanents have only real zeros. We will prove a vast generalization of MCPC using the theory of stable polynomials.
This is joint work with Haglund, Visontai and Wagner.

rotating-knot

Wednesday, September 23rd, 2009
h. 10:30 (2 ore) - Room 1101
Rita FIORESI (Bologna)
"Supergeometria - 4"

Abstract: La supergeometria si è rivelata uno strumento fondamentale in fisica per lo studio e la classificazione delle particelle elementari. Dopo i lavori fondazionali degli anni '70 e '80 del secolo scorso, di Berezin, Kostant e Manin, più recentemente altri matematici, fra i quali Bernstein e Deligne, si sono dedicati ad una trattazione più moderna di questa teoria utilizzando tecniche di geometria algebrica, prima fra tutte il funtore dei punti. Queste lezioni sono tratte dal libro "Mathematical Foundations of Supersymmetry", attualmente in corso di pubblicazione; che contiene una bibliografia esaustiva (per una versione ridotta e preliminare del libro, si veda il sito xxx.lanl.gov).
Lezione 4: Introdurremo la nozione di superschema e di funtore dei punti, con una particolare attenzione per i supergruppi algebrici affini e le loro superalgebre di Lie.
N.B: per il loro carattere introduttivo, queste lezioni si indirizzano anche a studenti di laurea specialistica.

ball-Moebius

Tuesday, September 22nd, 2009
h. 15:00 (2 ore) - Room 1101
Rita FIORESI (Bologna)
"Supergeometria - 3"

Abstract: La supergeometria si è rivelata uno strumento fondamentale in fisica per lo studio e la classificazione delle particelle elementari. Dopo i lavori fondazionali degli anni '70 e '80 del secolo scorso, di Berezin, Kostant e Manin, più recentemente altri matematici, fra i quali Bernstein e Deligne, si sono dedicati ad una trattazione più moderna di questa teoria utilizzando tecniche di geometria algebrica, prima fra tutte il funtore dei punti. Queste lezioni sono tratte dal libro "Mathematical Foundations of Supersymmetry", attualmente in corso di pubblicazione; che contiene una bibliografia esaustiva (per una versione ridotta e preliminare del libro, si veda il sito xxx.lanl.gov).
Lezione 3: Faremo una panoramica sui superspazi ed il loro funtore dei punti descrivendo numerosi esempi delle due tipologie più importanti di superspazio: le supervarietà differenziabili e i superschemi.
N.B: per il loro carattere introduttivo, queste lezioni si indirizzano anche a studenti di laurea specialistica.

Calabi-spin

Thursday, September 17th, 2009
Università di Roma "La Sapienza" - dipartimento di Matematica
h. 10:30 (2 ore) - Aula di Consiglio
Rita FIORESI (Bologna)
"Supergeometria - 2"

Abstract: La supergeometria si è rivelata uno strumento fondamentale in fisica per lo studio e la classificazione delle particelle elementari. Dopo i lavori fondazionali degli anni '70 e '80 del secolo scorso, di Berezin, Kostant e Manin, più recentemente altri matematici, fra i quali Bernstein e Deligne, si sono dedicati ad una trattazione più moderna di questa teoria utilizzando tecniche di geometria algebrica, prima fra tutte il funtore dei punti. Queste lezioni sono tratte dal libro "Mathematical Foundations of Supersymmetry", attualmente in corso di pubblicazione; che contiene una bibliografia esaustiva (per una versione ridotta e preliminare del libro, si veda il sito xxx.lanl.gov).
Lezione 2: Ripasseremo i concetti classici di fascio e spazio anellato, i rudimenti della teoria degli schemi e del funtore dei punti classico (N.B.: questa lezione non e' necessaria a chi già conosce e usa questi strumenti).
N.B: per il loro carattere introduttivo, queste lezioni si indirizzano anche a studenti di laurea specialistica.

ball-Moebius

Wednesday, September 16th, 2009
Università di Roma "La Sapienza" - dipartimento di Matematica
h. 15:00 (2 ore) - Aula di Consiglio
Rita FIORESI (Bologna)
"Supergeometria - 1"

Abstract: La supergeometria si è rivelata uno strumento fondamentale in fisica per lo studio e la classificazione delle particelle elementari. Dopo i lavori fondazionali degli anni '70 e '80 del secolo scorso, di Berezin, Kostant e Manin, più recentemente altri matematici, fra i quali Bernstein e Deligne, si sono dedicati ad una trattazione più moderna di questa teoria utilizzando tecniche di geometria algebrica, prima fra tutte il funtore dei punti. Queste lezioni sono tratte dal libro "Mathematical Foundations of Supersymmetry", attualmente in corso di pubblicazione; che contiene una bibliografia esaustiva (per una versione ridotta e preliminare del libro, si veda il sito xxx.lanl.gov).
Lezione 1: Studieremo l'algebra lineare nel contesto della supergeometria introducendo i concetti fondamentali di super spazio vettoriale, superalgebra, supergruppo lineare e superalgebra di Lie.
N.B: per il loro carattere introduttivo, queste lezioni si indirizzano anche a studenti di laurea specialistica.

rotating-knot

Monday, December 1st, 2008
h. 14:00 - Room "Roberta Dal Passo"
Alexander POSTNIKOV (M.I.T. - Boston)
"Totally positive Grassmannians, matroids and polytopes"

Abstract: We will discuss combinatorics coming from geometry of the totally positive Grassmannian. This object has an interesting structure of CW-complex. Its cells are the positive parts of Gelfand-Serganova matroid strata. They generalize type A double Bruhat cells of Fomin-Zelevinsky. This work is closely related to Fomin-Zelevinsky's cluster algebras. We will also discuss some recent joint results with Thomas Lam on extension of total positivity to the affine Grassmannian. Moment polytopes for positive points on the affine Grassmannian form an interesting class of convex polytopes, which we call polypositroids. These polytopes have remarkable combinatorial properties.

Calabi-spin

Monday, November 10th, 2008
h. 14:30 - Room "Roberta Dal Passo"
David BUCHSBAUM (Brandeis University)
"Il flusso dell'omologia attraverso l'algebra: un panorama selettivo"

Monday, June 16th, 2008
h. 14:50 - Room "Roberta Dal Passo"
Giovanni CERULLI IRELLI (Padova)
"Canonical bases and quiver Grassmannians in some cluster algebras of affine type"

Abstract: Cluster algebras were introduced by S.Fomin and A.Zelevinsky in 2001 with the aim of studying total positivity and canonical basis in semisimple algebraic groups. Here we present one of the first steps in the direction of finding canonical bases of such algebraic structure. We study a particular class of cluster algebras and we find their "canonical basis". We give an interpretation of such elements in terms of quiver Grassmannians. Such an interpretation is inspired by the works of P.Caldero and B.Keller. All the necessary definitions will be given. In particular no previous knowledge about cluster algebras is needed.

Calabi-spin

Monday, June 16th, 2008
h. 14:00 - Room "Roberta Dal Passo"
Riccardo ARAGONA (Tor Vergata)
"Semi-invariants of symmetric quivers"

Abstract: We shall describe the space of orthogonal and symplectic representations of a symmetric quiver, i.e. a quiver for which there exists an involution on the set of arrows and on the set of vertices. In particular we shall define an action of a product of classical groups on this space and, using definition of special types of semi-invariants for such action (Schofield), we shall display a set of generators of the ring of semi-invariants for symmetric quivers of finite type. Time permitting, we shall hint at the case of symmetric quivers of tame type.

ball-Moebius

Thursday, May 15th, 2008
h. 14:30 - Room 1101
Irasema SARMIENTO
"Hopf Algebras and transition polynomials"

Abstract: Transition polynomials of 4-regular graphs were defined by Jaeger. Many polynomials with a wide range of applications in mathematics and physics are transition polynomials. Such is the case for Penrose, Martin and Kauuman bracket polynomials. The Tutte polynomial of a plane graph is also a transition polynomial (in the case x=y). Transition polynomials for all Eulerian graphs were defined by the author and J.A. Ellis - Monaghan. We see generalized transition polynomials as homomorphisms of Hopf Algebras.

Calabi-spin

Escher-birds

Colloquium di Dipartimento
Tuesday, May 13th, 2008
h. 14:30 - Room "Roberta Dal Passo"
Christian KASSEL (Strasbourg)

"Quantum groups and knot theory"

Abstract: This is a general survey where I discuss the spectacular connection between quantum groups and topology. If time permits I'll present the way how from the general formalism behind this connection one can have the absolute Galois group of the rationals (a very typical object of number theory and still quite mysterious), which acts on the Vassiliev invariants of knots. Vassiliev invariants form a big class of invariants that includes all the new invariants that can be constructed from quantum groups, such as the famous Jones polynomial of knots.

ball-Moebius

Escher-birds

Colloquium di Dipartimento
Wednesday, April 23rd, 2008
h. 14:00 - Room "Roberta Dal Passo"
Jerzy WEYMAN (Northeastern University, Boston)

"Quivers, Clusters, Pictures"

Abstract: In this talk I will describe certain combinatorial structures of generalized associahedra appearing in several apparently unrelated constructions. The combinatorics of general decompositions of quiver representations turns out to be closely related to the theory of cluster algebras of Fomin-Zelevinsky and to the Igusa-Orr theory of pictures designed to describe the homology of nilpotent groups of upper-triangular matrices.
Departing from the definition of quiver representations and Gabriel, theorem I will define the generalized associahedra and sketch how they appear in the other areas mentioned above.

Calabi-spin

Friday, January 25th, 2008
h. 14:00 - Room "Roberta Dal Passo" (1201)
Antonin GUILLOUX (Università di Roma "Tor Vergata", LIEGRITS - ENS Lyon)
"Representations of linear groups: a dynamical point of view - 3"

Abstract: It is possible to study the theory of unitary representations of linear groups - for example SL(2,R) - from a dynamical point of view. And stating some theorems with the language of dynamical systems yields a good understanding of certain groups actions, namely actions of lattices in linear groups - for example the subgroup of matrices with integral entries SL(2,Z).
Here we will present a simple case, due to Margulis. We will introduce the hyperbolic plane and geometry together with the action of the groups SL(2,R) and SL(2,Z). Then we will state some results about unitary representations of SL(2,R) and see their dynamical interpretations to eventually count the cardinality of certain subsets of the hyperbolic plane.
This third (and last) lecture will be devoted to equirepartition and counting. We will see how the tools developed during the second lecture apply to the problem stated during the first.

ball-Moebius

Wednesday, January 23rd, 2008
h. 14:00 - Room "Roberta Dal Passo" (1201)
Antonin GUILLOUX (Università di Roma "Tor Vergata", LIEGRITS - ENS Lyon)
"Representations of linear groups: a dynamical point of view - 2"

Abstract: It is possible to study the theory of unitary representations of linear groups - for example SL(2,R) - from a dynamical point of view. And stating some theorems with the language of dynamical systems yields a good understanding of certain groups actions, namely actions of lattices in linear groups - for example the subgroup of matrices with integral entries SL(2,Z).
Here we will present a simple case, due to Margulis. We will introduce the hyperbolic plane and geometry together with the action of the groups SL(2,R) and SL(2,Z). Then we will state some results about unitary representations of SL(2,R) and see their dynamical interpretations to eventually count the cardinality of certain subsets of the hyperbolic plane.
This second lecture will be devoted to unitary representations of linear groups. We will focus on the example of SL(2,R) and then on the dynamical aspects.

Calabi-spin

Monday, January 21st, 2008
h. 14:00 - Room "Roberta Dal Passo" (1201)
Antonin GUILLOUX (Università di Roma "Tor Vergata", LIEGRITS - ENS Lyon)
"Representations of linear groups: a dynamical point of view - 1"

Abstract: It is possible to study the theory of unitary representations of linear groups - for example SL(2,R) - from a dynamical point of view. And stating some theorems with the language of dynamical systems yields a good understanding of certain groups actions, namely actions of lattices in linear groups - for example the subgroup of matrices with integral entries SL(2,Z).
Here we will present a simple case, due to Margulis. We will introduce the hyperbolic plane and geometry together with the action of the groups SL(2,R) and SL(2,Z). Then we will state some results about unitary representations of SL(2,R) and see their dynamical interpretations to eventually count the cardinality of certain subsets of the hyperbolic plane.
This first lecture will be devoted to the hyperbolic plane and the actions of SL(2,R) and SL(2,Z). Moreover, we will state the main result of the course.

rotating-knot

Monday, December 10th, 2007
h. 14:30 - Room 1101
Thomas BLIEM (Università di Roma "Tor Vergata", LIEGRITS)
"On weight multiplicities of complex simple Lie algebras"

Abstract: I will explain parts of a line of thought which eventually leads to complete knowledge of the characters of complex simple Lie algebras, namely: using work by Littelmann, one can express weight multiplicities of simple representations as numbers of points in certain families of polytopes. From this one obtains a presentation of the weight multiplicity function as a vector partition function. Hence, by Sturmfels' structure theorem on vector partition functions, the weight multiplicity function is piecewise quasi-polynomial. The actual domains of quasi-polynomiality and the corresponding quasi-polynomials can be calculated using the method of inverse Laplace transformation and work by De Concini and Procesi on the Jeffrey-Kirwan residue. I will present computations for so(5, C). Parts of the talk will be elementary and accessible to any student having followed an introductory course in complex analysis.

ball-Moebius

Wednesday, June 20th, 2007
h. 14:30 - Room 1101
Giovanni CERULLI IRELLI (Padova)
"Cluster algebras with coefficients and
their relations with tropical geometry"

Abstract: The general definition of a cluster algebra A depends on the choice of a "semifield", in which the group of the "coefficients" of A lies. In many geometric realizations of A this semifield is a "tropical semifield", such as those which are commonly used in tropical geometry.
We shall present the main definitions and results of the recent work "Cluster algebras. IV. Coefficients" [Compos. Math. 143 (2007), 112-164] by S. Fomin and A. Zelevinsky. Moreover, we shall give the example of the rank 2 cluster algebras.
All needed definitions will be given. In particular, no previous knowledge of the theory of cluster algebras is assumed.

Calabi-spin

Thursday, June 14th, 2007
h. 14:30 - Room 1101
Giovanni CERULLI IRELLI (Padova)
"Cluster algebras and quiver representations"

Abstract: Any antisymmetric matrix B is associated to a quiver Q(B) and to a cluster algebra (without coefficients) A(B). In the last years, the study of a quotient category, called "cluster category", of Rep(Q(B)) has been widely developed. The tilting objects of this category correspond to a set of generators of A(B) called "clusters". This correspondence has found an explicit form in terms of "quiver Grassmannians". Without assuming any knowledge of the theory of cluster algebras, we shall provide such a correspondence.

ball-Moebius

Escher-birds

Colloquium di Dipartimento
Thursday, May 31st, 2007
h. 14:30, Room 1201
Louis J. BILLERA (Cornell University")

"Quasisymmetric functions for Bruhat intervals and Kazhdan-Lusztig polynomials"

Abstract: We associate a quasisymmetric function to any Bruhat interval in a general Coxeter group. This association can be seen to give a morphism of Hopf algebras to the subalgebra of all peak functions, leading to an extension of the usual cd-index of the interval to the complete cd-index. We show how the Kazhdan-Lusztig polynomial of the Bruhat interval can be expressed in terms of this complete cd-index and otherwise explicit combinatorially defined polynomials. This is joint work with Franceso Brenti.

Calabi-spin

Monday, May 21st, 2007
h. 14:30 - Room 1103
Filippo VIVIANI (Roma "Tor Vergata" - Djursholm "Mittag-Leffler")
"Restricted simple Lie algebras and their deformations"

Abstract: A Lie algebra over a field of positive characteristic p is called restricted (or a p-Lie algebra) if it is endowed with a p-map which resembles the properties of the map that sends a derivation to its p-th power. This p-map appears naturally when considering the Lie algebra of a group scheme in positive characteristic. And conversely, to every restricted Lie algebra one can associate a finite group scheme with vanishing Frobenius morphism.
A great progress in this theory has been the recent classification of the simple restricted Lie algebras (over an algebraically closed field of positive characteristic different from 2 and 3) by Wilson-Block-Premet-Strade.
In the first part of this talk, I will review this classification.
In the second part, I will consider the problem of deforming these simple restricted Lie algebras as well as their associated finite group schemes.

ball-Moebius

Friday, May 18th, 2007
h. 14:30 - Room 1101
Filippo VIVIANI (Roma "Tor Vergata" - Djursholm "Mittag-Leffler")
"Group schemes and Lie algebras in positive characteristic"

Abstract: The Lie algebra associated to an affine group scheme over a field of positive characteristic p is naturally equipped with a p-operation. In other words, it is naturally a restricted or p-Lie algebra. Moreover, this restricted Lie algebra is closely related to the first Frobenius kernel of the group scheme. In the first part of the talk, I will review these classical constructions. In the second part, I will concentrate on finite group schemes. In particular, I will show that a simple finite group scheme over an algebraically closed field of positive characteristic p corresponds either to a simple (abstract) finite group or to a simple Lie algebra. Both these simple objects have been classified (the latter ones only in the case that p is different from 2 and 3).
If time permits, I will discuss the deformations of these simple finite group schemes.

Calabi-spin

Friday, May 11th, 2007
h. 14:00 - Room 1103
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - XV"

Tuesday, May 8th, 2007
h. 14:00 - Room 1103
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - XIV"

Friday, May 4th, 2007
h. 14:00 - Room 1103
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - XIII"

Wednesday, May 2nd, 2007
h. 14:00 - Room 1200
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - XII"

Friday, April 27th, 2007
h. 14:00 - Room 1103
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - XI"

Tuesday, April 24th, 2007
h. 14:00 - Room 1103
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - X"

Friday, April 20th, 2007
h. 14:00 - Room 1103
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - IX"

Tuesday, April 17th, 2007
h. 14:00 - Room 1103
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - VIII"

Thursday, April 12th, 2007
h. 14:00 - Room 1103
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - VII"

Tuesday, April 10th, 2007
h. 14:00 - Room 1103
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - VI"

Tuesday, April 3rd, 2007
h. 14:00 - Room 1103
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - V"

Monday, April 2nd, 2007
h. 14:00 - Room 1101
David HERNANDEZ (CNRS - Versailles)
"Structure of minimal affinizations of representations of quantum groups"

Abstract: Minimal affinizations of representations of quantum groups are relevant modules for quantum integrable systems. We present new results on their structure: we prove that all minimal affinizations in types A, B, G are "special" in the sense of monomials (an analog property is also proved for a large class in types C, D, F). As an application, the Frenkel-Mukhin algorithm works for these modules, and we prove previously predicted explicit character formulas in types A, B.

Calabi-spin

Friday, March 30th, 2007
h. 14:00 - Room 1103
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - IV"

Wednesday, March 28th, 2007
h. 14:00 - Room 1101
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - III"

Friday, March 23rd, 2007
h. 14:00 - Room 1103
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - II"

Tuesday, March 20th, 2007
h. 14:00 - Room 1103
Ph. D. course
Jerzy WEYMAN (NU Boston - INdAM)
"Quiver representations and applications - I"

Abstract: In recent years there was a renewed interest in representations of quivers. They turned out to be related to Klyachko's solution of Horn conjecture of eigenvalues of Hermitian matrices. In another development the connection of cluster algebras of Fomin and Zelevinsky to certain quivers with relations, so-called cluster-tilted algebras, gave new impulse to the representations of quivers. The area developes very actively now and offers opportunities for further research.
The purpose of the course is to introduce the students to these ideas. The first part will cover the basics of quiver representations, the proof of Gabriel Theorem, Auslander-Reiten theory, generic decompositions and semi-invariants. We will just discuss hereditary case (quivers without reations) so all these notions can be developed without too many technicalities. The second part will deal with connections with cluster algebras, i.e. the cluster categories and the cluster tilted algebras. We will deal mainly with the simplest case of quivers of type A_n which can be made very explicit.

Calabi-spin

Monday, March 7th, 2007
h. 16:00 - Room 1101
Roberto LA SCALA (Bari)
"Costandard modules of Schur superalgebras in characteristic p"

Abstract: In this talk we consider the problem of describing the costandard modules V(l) of a Schur superalgebra S(m|n,r) over a base field K of arbitrary characteristic. Precisely, if G = GL(m|n) is a general linear supergroup and Dist(G) its distribution superalgebra we compute the images of the Kostant Z-form under the epimorphism from Dist(G) to S(m|n,r). Then we describe V(l) as the null-space of some set of superderivations and we obtain an isomorphism between V(l) and the tensor product of V(l₊|0) times V(0|l_-) assuming that l = (l₊|l_-) and l_m = 0 . If char(K) = p, we give a Frobenius isomorphism between V(0|pm) and V(m)^p where V(m) is a costandard module of the ordinary Schur algebra S(n,r). Finally, we provide a characteristic free linear basis for V(l|0) which is parametrized by a set of superstandard tableaux.

ball-Moebius

Monday, March 7th, 2007
h. 14:30 - Room 1101
Andrea BRINI (Bologna)
"Irreducible representations of general linear Lie superalgebras. Algebraic and combinatorial aspects"

Abstract: We provide a brief outline of the basic ideas of Kac's approach to the representation theory of finite dimensional simple Lie superalgebras based upon the notion of highest weights and highest weight vectors and describe in detail the case of general linear Lie superalgebras. The representation theory of these superalgebras is very close to the representation theory of the so-called basic classical simple Lie superalgebras of type I.
The main constructions are, in this context, those of the integral highest weight modules and of the Kac modules relative to dominant integral highest weights. A deep result of Kac states that highest weight modules and Kac modules coincide if and only if the highest weight of the representation is a typical one.
The irreducible module that appear in the theory of letterplace algebra representations are covariant modules. Covariant modules are finite dimensional highest weight representations but they are not, in general, Kac modules, since their highest weights can be atypical.
We provide a detailed combinatorial analysis of covariant modules as highest weight representations, as well as a direct description of their highest weights and highest weight vectors; these results follows at once from the fact that covariant modules are Schur-Weyl modules. We mention that the theory of letterplace representations yields - up to the action of the so-called umbral operator - the decomposition theory of the super-symmetric algebra S²(V) and of the super-antisymmetric algebra of S²(V), recently rediscovered by Cheng and Wang and Sergeev.

Calabi-spin

Monday, March 5th, 2007
h. 14:30 - Room 1103
Riccardo ARAGONA (Roma "Tor Vergata")
"An introduction to the study of quiver representations"

Abstract: We shall describe the category whose objects are the representations of a finite quiver. In particular, we shall consider an action of the general linear group on the set of representations of a quiver Q. We shall define special types of semi-invariants for such an action (Schofield) and we shall show that they generate the ring of semi-invariants when Q has no oriented cycles (Derksen-Weyman). Moreover, we shall generalize this last result to the case of any quiver (Domokos-Zubkov). Finally, from this we shall obtain a description of the ring of invariants for any quiver in characteristic 0 (Le Bruyn-Procesi).

ball-Moebius

Monday, February 26th, 2007
h. 14:30 - Room 1101
Benjamin ENRIQUEZ (Strasbourg)
"Quantization of coboundary Lie bialgebras"

Abstract: We will first recall the theory of Lie bialgebras and their quantizations as quantum groups. The main problems in this theory are "quantization problems". While the central problem (quantization of Lie bialgebras) was solved in 1994 by Etingof and Kazhdan, other problems are still open (e.g., quantization of quasi-Lie bialgebras). In this talk, we will describe our solution (joint with G. Halbout) of the quantization problem of coboundary Lie bialgebras. The main ingredient in this work is the fact that Etingof-Kazhdan quantization is compatible with twists of Lie bialgebras. To prove this, one should prove that the Etingof-Kazhdan approach to quantization is equivalent to another approach (the "sophisticated" approach in the terms of Drinfeld), that of constructing a universal twist killing a given associator.
After a review of the key points of the proof, we will describe one application of our work: the quantization of certain quasi-Poisson homogeneous spaces.

rotating-knot

Wednesday, December 6th, 2006
h. 14:00 - Room 1201
Giovanna CARNOVALE (Università di Padova)
"Spherical conjugacy classes and involutions in the Weyl group"

Abstract: Let G be a simple algebraic group over an algebraically closed field of good characteristic, let B be a Borel subgroup of G and let W be the Weyl group of G. A conjugacy class C is called "spherical" if there exists a dense B-orbit in C. We shall set a relation between the Bruhat decomposition of G and the spherical conjugacy classes, showing that if BwB intersects such a class C, then w is an involution in W. Thanks to this result, we shall prove that if C is a spherical conjugacy class of G, and its dense B-orbit v is contained in BwB, then dim(C) = l(w) + rk(1-w). This extends - to the case of a field of good characteristic - a characterization of the spherical conjugacy classes previously obtained in collaboration with Nicoletta Cantarini and Mauro Costantini. Time permitting, we shall present some applications of these results.

Calabi-spin

May 29-31, 2006
h. 11:00 - Room 1201
Christoph SCHWER (LIEGRITS, Roma "Tor Vergata")
"Combinatorial formulas for weight multiplicities and q-analogs"

Abstract: We want to prove a combinatorial formula for weight multiplicities of a semisimple complex Lie algebra g in terms of galleries. The approach is based on calculating certain q-analogs of these weight multiplicities using the affine Hecke algebra.

ball-Moebius

Tuesday, April 11th, 2006
h. 14:30 - Room 1103
Francesco VACCARINO (Politecnico di Torino)
"Representations, symmetric products and Hilbert schemes II: non commutative case and Cayley-Hamilton rings"

Abstract: Let K be an infinite field or the ring of integers. Let M_n(K) denote the m-tuples of nxn-matrices, and F_m the free associative K-algebra on m generators. Let (TSⁿR)^ab be the abelianization of the symmetric tensors of order n over R, with R a K-algebra.
We first show that algebra of invariants K[M_n^m(K)]^GL_n(K) is isomorphic to (TSⁿF_m)^ab via the composition of an n-dimensional representation of F_m with the determinant.
A Cayley-Hamilton ring is a ring with trace in which a formal analogue to the Cayley-Hamilton Theorem holds. We give a characteristic free approach to Cayley-Hamilton rings via norms, i.e. determinants, and we use the above isomorphism to show that the algebra of invariants M_n(K[M_n^m(K)])^GL_n(K) is free in the category of n-Cayley-Hamilton rings, thus generalizing a result due to Procesi.
Then we study under this light the generalized Hilbert-Chow morphism from the Hilbert scheme of n-codimensional left ideals of R to the spectrum of TSⁿ(R)^ab, which coincides with the Hilbert-Chow morphism when R is commutative and K is algebraically closed.

Calabi-spin

Monday, April 10th, 2006
h. 14:30 - Room 1101
Francesco VACCARINO (Politecnico di Torino)
"Representations, symmetric products and Hilbert schemes I: schemes and morphisms"

Abstract: Let K be an infinite field, and let R be a not necessarily commutative K-algebra.
There are at least three schemes that are directly connected to representation theory of R:
-1) Rep_n(R), that is the coarse moduli space parameterizing the equivalence classes of n-dimensional linear representations of R, i.e. the affine scheme associated to a ring of invariants defined by R;
-2) Hilb_n(R), that is the Hilbert scheme parameterizing the left ideals of codimension n of R;
-3) T_n(R), that is the generalized symmetric product defined by the abelianization of the symmetric tensors of order n over R.
These three objects are connected by suitable morphisms. In this talk I will introduce the above framework, and I will give sketches of some of the results that will be discussed in full details in the next two seminars.

ball-Moebius

Monday, March 27th, 2006
h. 14:00 - Room 1201
Cristoph SCHWER (LIEGRITS, Roma "Tor Vergata")
"Galleries and their q-analogs"

Wednesday, January 24th, 2006
h. 14:30 - Room 1103
Caroline GRUSON (Université de Nancy)
"On the odd nilpotent cone of orthosymplectic Lie superalgebras"

Abstract: We will give a description of the nilpotent cone of osp(m,2n,C) and show a desingularization in the case of osp(2n,2n+1,C).

rotating-knot

Friday, December 16th, 2005
h. 14:30 - Room 1101
Sebastien JANSOU (LIEGRITS, Roma "Tor Vergata")
"Examples of invariant Hilbert schemes"

Abstract: For a connected reductive group G, and a finite dimensional G-module V, Alexeev and M. Brion have built the invariant Hilbert scheme: it parametrizes G-stable closed subschemes of V affording a fixed, multiplicity-finite representation of G in their coordinate ring. We shall describe this scheme in the simplest case, where it parametrizes invariant deformations of the cone of primitive vectors of a simple G-module. The classification we get is related to those of simple Jordan algebras and of wonderful varieties of rank one whose open orbit is affine.

ball-Moebius

Escher-birds

Colloquium di Dipartimento
Friday, November 25th, 2005
h. 15:00, Room 1201
Mina TEICHER (Bar-Ilan University)

"Degenerations, Braid Monodromy type and Fundamental Groups"

Abstract: In this talk we will present old and new topological and complex invariants of algebraic surfaces such as fundamental groups and the Braid Monodromy Type. We will discuss algorithms constructed for computing the above invariants (mathematical and computational) including degenerations of surfaces. Finally we present few examples and unexpected applications to cryptography.

Calabi-spin

Monday, November 14th, 2005
h. 15:00 - Room 1101
Jerzy WEYMAN (Northeastern University, Boston)
"Generic decomposition for quiver representation"

Escher-birds

Colloquium di Dipartimento
Wednesday, October 19th, 2005
h. 15:00, Room 1201
Arun RAM (University of Wisconsin)

"Diagram algebras as tantalizers"

Abstract: The diagram algebras are a family of algebras that can be defined combinatorially in terms of the multiplication of diagrams. Some examples are Brauer algebras, Hecke algebras and group algebras of affine braid groups. These algebras all occur as tantalizers (tensor power centralizers) which means that one can use a generalized Schur-Weyl duality to provide a combinatorial analysis of their representation theory.

Calabi-spin

Tuesday, May 31st, 2005
h. 14:30 - Room 1101
Alessandro D'ANDREA (Roma 1)
"Groups of quaternions with algebraic coefficients"

Abstract: A celebrated theorem by Tits states that a linear group over a field of zero characteristic either has a non abelian free group or possesses a solvable subgroup of finite index. The original proof by Tits is highly nonconstructive, and there has been some recent interest in effective versions of Tits' result in particular cases.
We will focus on a technique which makes it possible to prove Tits' alternative for a large class of groups of quaternions with algebraic coefficients.

ball-Moebius

Escher-birds

Colloquium di Dipartimento
Monday, May 2nd, 2005
h. 14:00, Room 1201
Peter LITTELMANN
(Bergische Universitaet Wuppertal)

"Representation theory, geometry and combinatorics,
from Young tableaux to the loop Grassmannian"

Abstract: A little more than 100 years ago, Issai Schur published his pioneering PhD thesis on the representations of the group of invertible complex (n x n)-matrices. At the same time, Alfred Young introduced what later came to be known as the Young tableau. The tableaux turned out to an extremely useful combinatorial tool in representation theory, but they are also a very convenient instrument for the investigation of geometric problems related to Grassmann varieties, for example in K-theory or in the standard monomial theory. Later, in the connection with quantum groups and crystal bases, once again the Young tableaux turned out to be a natural tool to control the combinatorial aspects of the theory.
This talk will explore a few of these appearances of the ubiquitous Young tableaux and also discuss some more recent generalizations of the tableaux and the connection with the geometry of the loop Grassmannian.

Calabi-spin

April 18-20-22, 2005
h. 14:00 - Room 1301
David HERNANDEZ (LIEGRITS, Roma "Tor Vergata")
"Introduction to the Theory of Representations of Quantum Groups"

Abstract: The aim of this course is to study from scratch some basic and explicit examples of representations of various quantum groups, and to relate them to active and fast developing fiels of research. First, we will focus on simple representations of quantum algebras of type sl(2), and relate them to the colored Jones polynomials of knots and the volume conjecture. Besides we will construct the Kirillov-Reshetikhin modules of sl(2) quantum affine algebras, describe the T-system exact sequence explicitely and relate it to fermionic formulas and the corresponding integrable systems.

ball-Moebius

Wednesday, April 6th, 2005
h. 14:00 - Room 1101
Jesus DE LOERA (University of California at Davis)
"On the computation of Clebsch-Gordan coefficients and the dilation effect"

Monday, March 14th, 2005
h. 14:30 - Room 1101
David HERNANDEZ (LIEGRITS, Roma "Tor Vergata")
"Quantum characters, fusion procedure and applications
to the representation theory of affinized quantum algebras"

Abstract: The class of affinized quantum Kac-Moody algebras includes in particular quantum affine algebras and quantum toroidal algebras. Their representation theory is very rich and has been intensively studied by various people in recent years. In this talk, we will present some recent results for these representations, in particular by focussing on a fusion procedure: in general these algebras have no Hopf algebra structure, however we propose a construction of a fusion product on the Grothendieck group of l-highest weight integrable representations. This new fusion procedure uses a one parameter deformation of the "Drinfeld coproduct" and is closely related to a generalization of Frenkel-Reshetikhin q-characters. Moreover in the case of quantum affine algebras it gives a new interpretation of the usual Grothendieck ring.

ball-Moebius

Monday, March 7th, 2005
h. 14:30 - Room 1103
Rita FIORESI (Bologna)
"Commutation relations of quantum minors
in the quantum matrix bialgebra"

Abstract: In the bialgebra of quantum matrices, one can define the notion of quantum determinant of any minor. Given two such minors, we want to write down the commutation rules among their determinants and, if possible, to describe the ring generated by the determinants of a given subset of minors. As an application of these computations, we show the description of the quantum flag variety in terms of generators and relations and the quantum invariant theory for quantum special linear group.

rotating-knot

Monday, June 7th, 2004
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Damiano TESTA (MIT Boston)
"Spaces of stable maps"

Abstract: I shall talk of an extension of the paper "Enumeration of Rational Curves via Torus Action" by Kontsevich in "The Moduli Space of Curves, R. Dijkgraaf, C. Faber, and G. van der Geer (eds.). I shall start with the classical example of counting the number of lines on a smooth cubic surface in P³. We shall see how it is possible to read such a number as the number of zeroes of a section on a natural fiber bundle on the Grassmannian of lines in the space P³. Inspired by this example, we shall tackle the problem on quintic hypersurfaces in P⁵ in the same way. As a first step, we shall have to generalize the space which characterizes the lines in P³ to a space which parametrizes rational curves of degree d in P³. Once this construction is established, we shall have natural candidates of a vector bundle on such a space and a section whose vanishing can be thought of as the presence of rational curve of degree d on the quintic hypersurface Q. At this stage the equality of the dimension of the space of rational curves and of the rank of the bundle will enable us to state that a generic section of this bundle will have finitely many zeroes, which we shall use as definition of "the number of rational curves of degree d on Q". In order to compute the number of zeroes of a generic section, we shall integrate the Euler class of the bundle over the space of stable maps. We shall exploit the natural action of the diagonal matrices on P⁴ to explicitly compute the integral: this action induces an action on the space of all rational curves of degree d, simply by composition. This will enable us to compute the integral as an equivariant cohomology, and we shall be able to use Bott localization formula to reduce the integral over the whole space to an integral only over the subvariety of fixed points of this action. These fixed points have a combinatorial description in terms of marked graphs, and the last step consists in a computation on the tangent spaces which make it possible to compute the characters of the action of the torus on the normal bundle of fixed points. Gathering together all these information we shall get the result.

Calabi-spin

Monday, May 10th, 2004
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Emanuela PETRACCI (Geneve)
"Classical dynamical Yang-Baxter equation: a special solution"

Abstract: Given a Lie algebra and a Lie subalgebra of it, we have a classical dynamical Yang-Baxter equation. Alekseev and Meinrenken provided a particular solution of it, which is valid for quadratic Lie algebras. This occurs both in the framework of Lie theory and in mathematical physics. We shall give an introduction to the solution and we shall explain how it extends to quadratic Lie superalgebras.

ball-Moebius

Thursday, April 29th, 2004
h. 16:00 - Room 1101
Workshop "TV2004 - The interplay of
representation theory, Poisson geometry and quantization"
Paolo LORENZONI (Milano "Bicocca")
"Deformations of bihamiltonian structures of hydrodynamic type"

Abstract: We discuss a perturbative approach to the classification problem of a certain class of bihamiltonian hierachies of PDEs depending on a small parameter and its applications to the theory of dispersive waves. The r.h.s. of the equations of these hierarchies usually are formal series in the dispersion parameter. Truncating these series one obtains an "approximately integrable systems" since the vector fields of the truncated hierarchy commute up to a certain order in the deformation parameter. This fact suggest that these approximately integrable systems could have, at least for small times, multi-soliton solutions. The numerical experiments we have performed confirm this hypothesis.

Calabi-spin

Thursday, April 29th, 2004
h. 14:00 - Room 1101
Workshop "TV2004 - The interplay of
representation theory, Poisson geometry and quantization"
Gilles HALBOUT (Strasbourg)
"Tamarkin's formality and globalization"

Abstract: I will give the general framework of D. Tamarkin's formality theorem. To do so, I will recall the definition of G_\infty structures, recall Etingof-Kazhdan's quantization-dequantization theorem for Lie bialgebras (and introduce recent works of Enriquez and Enriquez-Etingof), and I will speak about globalization procedure. I will end with a few questions, recent works and works in progress related to that topic:
- globalization of Tamarkin's (and Tamarkin-Tsygan's) formality theorem,
- comparison of the construction of M. Kontsevich and D. Tamarkin,
- application to the problem of star-representations on coisotropic submanifolds,
- quantization of quasi-Poisson manifolds.

ball-Moebius

Thursday, April 29th, 2004
h. 11:00 - Room 1101
Workshop "TV2004 - The interplay of
representation theory, Poisson geometry and quantization"
Alessandro D'ANDREA (Roma "La Sapienza")
"Conformal algebras and pseudoalgebras"

Abstract: I will provide a well-motivated introduction to the study of conformal algebras and of pseudoalgebras. In particular, I will describe their relationship with vertex algebras, linearly compact Lie algebras, representation theory, Poisson algebras of hydrodynamical type.

Calabi-spin

Thursday, April 29th, 2004
h. 9:00 - Room 1101
Workshop "TV2004 - The interplay of
representation theory, Poisson geometry and quantization"
Alberto S. CATTANEO (Universitat Zurich)
"Deformation quantization of coisotropic submanifolds (2)"

Abstract: Coisotropic submanifolds play a fundamental role in symplectic and Poisson geometry as they describe systems with symmetries ("first-class constraints") and provide a method to generate new symplectic or Poisson spaces ("symplectic or Poisson reduction"). Though often singular, these reduced spaces possess anyway a Poisson algebra of functions that is defined as a quotient from the Poisson algebra of the original Poisson manifold. Then an interesting question is whether it is possible to quantize these Poisson algebras (i.e., to deform the underlying commutative algebras in the direction of the Poisson bracket) even when the spaces are singular. In this second talk, I will first discuss how coisotropic submanifolds are related to the possible boundary conditions for the Poisson sigma model and how to use it to get a generalization of Kontsevich's formula in the presence of coisotropic submanifolds. Then I will present the relevant formality theorem and from it I will derive the possible obstructions to the deformation quantization of a reduced Poisson manifold. An interpretation in terms of supermanifolds and the relation with the Batalin-Fradkin formalism may also be given. Time permitting, I will discuss how to associate to two intersecting coisotropic submanifolds a bimodule for the deformed algebras of the two reduced spaces. This suggests that deformation quantization should be regarded as a (partially defined?) functor.

ball-Moebius

Wednesday, April 28th, 2004
h. 16:30 - Room 1101
Workshop "TV2004 - The interplay of
representation theory, Poisson geometry and quantization"
Andrea MAFFEI (Roma "La Sapienza")
"Modular representations of Lie algebras and geometry of Springer fibres"

Abstract: The goal of this lecture is to provide an introduction to the ideas in the paper by Bezrukavnikov, Mirkovic and Rumynin about representations of Lie algebras of semisimple Lie groups in characteristic p. Their methods work splits into two subsequent steps. The first one is an analogue, in characteristic p, of the theorem of Beilinson and Bernstein on the localization of g-modules, and "reduces" the study of the representation theory of Lie algebras (with Harish-Chandra character 0 and Frobenius character X) to the study of the D-modules on the flag variety supported onto a formal neighbourhood of the Springer fibre defined by X. The second step instead reduces the study of D-modules to that of coherent sheaves on the Springer fibre. As an application of these methods one proves Lusztig's conjecture on the number of irreducible representations with fixed character, and one gives a new proof of Kac-Weisfeiler's conjecture on the dimension of irreducible representations.

Calabi-spin

Wednesday, April 28th, 2004
h. 14:30 - Room 1101
Workshop "TV2004 - The interplay of
representation theory, Poisson geometry and quantization"
Davide FATTORI (Padova)
"An introduction to conformal (super)algebras"

Abstract: In this talk we will provide an overview based on examples of the theory of finite Lie conformal (super)algebras. Formal distributions will be introduced as a motivation. The notion of locality will then lead to the notion of a formal distribution Lie (super)algebra. Next, we will define Lie conformal (super)algebras and illustrate some of their features on examples. Also, we will discuss central extensions, physical Virasoro pairs and their connection to superconformal algebras.

ball-Moebius

Wednesday, April 28th, 2004
h. 11:30 - Room 1101
Workshop "TV2004 - The interplay of
representation theory, Poisson geometry and quantization"
Alberto S. CATTANEO (Universitat Zurich)
"Deformation quantization of coisotropic submanifolds (1)"

Wednesday, April 28th, 2004
h. 9:30 - Room 1101
Workshop "TV2004 - The interplay of
representation theory, Poisson geometry and quantization"
Alessandro D'ANDREA (Roma "La Sapienza")
"An introduction to Lie algebra representations in characteristic p"

Abstract: The aim of this talk is to provide an introduction to the subsequent one by Andrea Maffei. I shall describe some introductory results of the representation theory in characteristic p, stressing the main differences with the case of characteristic 0.

ball-Moebius

Monday, April 19th, 2004
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Fabrizio CASELLI (Lyon 1)
"(Co-)Invarianti algebras and greater indices for classical Weyl groups"

Abstract: The study of suitable actions of the classical Weyl groups on the ring of polynomials, and of the Hilbert series and of the corresponding algebras of invariants, leads to a natural extension of the major index also for Weyl groups of type B and D, this also being equidistributed with the legth function. One introduces a monomial basis for the coinvariant algebra, indexed by the elements of the associated Weyl group, in which the degree of a an element of the basis is equal to the major index of the corresponding element. This basis leads in a natural way to the definition of a new family of descent representations, which decompose the homogeneous components of the coinvariant algebra itself. Moreover, the multiplicity of the irreducible characters within the characters of these representations can be described in terms of tableaux.

Calabi-spin

Monday, March 15th, 2004
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Dmitrij KOZLOV (KTH Stokholm)
"Topological obstructions to graph colorings"

Abstract: For any two graphs G and H, Lovasz has defined a cell complex Hom(G,H) having in mind the general program that the algebraic invariants of these complexes should provide obstructions to graph colorings. Here we announce the proof of a conjecture of Lovasz concerning these complexes with G a cycle of odd length. More specifically, we show that if Hom(C_(2r+1),G) is k-connected, then χ(G) >= k+4. Our actual statement is somewhat sharper, as we find obstructions already in the non-vanishing of powers of certain Stiefel-Whitney characteristic classes.

ball-Moebius

Monday, February 16th, 2004
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Gabriele MONDELLO (Roma "La Sapienza")
"Towards a deformation theory of dg-schemes"

Abstract: The structure of differential graded Lie algebra (DGLA) naturally shows up in many deformation problems. It is the case, for instance, of Khaler varieties and of affine algebraic schemes over a zero characteristic field. The talk will consist in an introduction to the theory of deformations through DGLAs, and in a sketch of some of many open problems.

Calabi-spin

Monday, January 12th, 2004
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Alberto DE SOLE (Harvard)
"Finitely generated conformal vertex algebras"

Abstract: Vertex algebras give a rigorous mathematical definition of the chiral part of a 2-dimen-sional quantum field theory. It is an interesting problem, both from a mathematical and a physical point of view, to classify finitely generated vertex algebras which contain a Virasoro field. I will discuss a way to appoach this problem, based on the notion of "non-linear" Lie conformal algebra.

rotating-knot

Tuesday, December 9th, 2003
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Enrico SBARRA (Trieste)
"Local cohomology and Castelnuovo-Mumford regularity"

Abstract: The first part ot the talk is meant to be an introduction to the concept of Castelnuovo-Mumford regularity. We recall the definition and we discuss some of the methods used to study it, with a special concern about lexicographic and generic ideals, and Local Cohomology. The second part is a brief communication about a joint work with Giulio Caviglia of the University of Kansas: we discuss a newly proven characteristic-free bound for the Castelnuovo-Mumford regularity that generalizes a previous result of Bayer and Mumford.

ball-Moebius

Monday, November 24th, 2003
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Simone BORGHESI (SNS Pisa)
"Homotopy theory and algebraic geometry"

Abstract: In classical homotopy theory one studies good topological spaces and maps among them "up to homotopy". The outcome is that two topological s[aces are identified if they share the "same homotopy type". After V. Voevodsky's work (and partially also F. Morel's) the same can be done in algebraic geometry. Hence one obtains a category in which occur homotopy theory invariants, algebraic invariants and curious relations among them, whom - today - we probably see only the top of the iceberg of. In the talk we shall discuss such an example: how to produce cohomological theories (algebraic Morava K-theories) defined, in particular, on varieties over a perfect field and obtained from iterated extensions (in the sense of triangulated categories) of higher Chow groups. Homotopy theory (and computations by Voevodsky) provides the tools to extend in a non-trivial way these cohomological theories. This non-triviality is at the basis of the proof of surprising formulas (the so-called degree formulas) which relate some characteristic numbers of two smooth and projective algebraic varieties - homotopic invariantis - and the degree of any map between them, whenever the target variety of the map satdisfy a suitable algebraic condition.

Calabi-spin

Thursday, June 19th, 2003
h. 14:00 - Room 1201
Jovo JARIC (Belgrade)
"On the decomposition of symmetric tensors into traceless symmetric tensors"

Abstract: There are many problems concerning formulation constitutive equations in continuum mechanics which can be reduced to the determination of an irreducible integrity basis of invariants for given set of tensors under a given group of transformations. The way in which such problems arise is examined by Rivlin and Pipkin. However, Spencer was the first to consider the important case of orthogonal tensors in which the transformation group is the full or proper in the three dimensions. The generation of these integrity bases is simplified if tensors are, whenever possible, decomposed into simpler tensors with fewer independent components. The purpose of this talk is to give the explicit method of effecting decomposition of symmetric tensors into traceless symmetric tensors of finite order in n-dimensional Euclidean space. We generalize the definition of traceless tensors in order to include into traceless tensors tensors of zero and first order. Also, we intend to give complete procedure instead of deriving formulae for tensors of particular order. Final results are so general that they can be presented by table. As a conclusion there are two things to be emphasized. First, the procedure involves only elementary algebra and makes no use of group theory. Second, the procedure presented here is not unique.

ball-Moebius

Wednesday, June 18th, 2003
h. 16:00 - Room 1103
Neda BOKAN (Belgrade)
"Grassmann manifolds and Grassmann structures"

Wednesday, June 18th, 2003
h. 15:00 - Room 1103
Zoran RAKIC (Belgrade)
"Lagrangian aspects of quantum dynamics on a noncommutative space"

Abstract: In order to evaluate the Feynman path inegral in noncommutative quantum mechanics, we consider properties of a Lagrangian related to a quadratic Hamiltonian with noncommutative spatial coordinates. A quantum-mechanical system with noncommutative coordinates is equivalent to another one with commutative coordinates. We found a connection between quadratic classical Lagrangians of these two systems. We also show that there is a subclass of quadratic Lagrangians, which include harmonic oscillator and particle in a costant field, whose connection between ordinary and noncommutative regimes can be expressed as a linear change of position in terms of a new position and velocity.

ball-Moebius

Monday, June 16th, 2003
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Lorenzo CORNALBA (Amsterdam)
"Noncommutative Geometry in String Field Theory"

Abstract: String theory, though being a consistent theory of gravity, is always described as a power-series expansion around classical solutions of the equations of motion, called string backgrounds. It therefore lacks a "global" definition, based possibly on new geome-trical principles and, surely, on principles of symmetry. One of the characteristics of string theory is that, contrary to a classical theory of fields, is not described by non-linear local partial diffe-rential equations, but by non-local equations which involve deriva-tives of the fields to all orders. Therefore, a description based on classical manifolds, fields and their derivatives is at best an appro-ximation to the correct structure of the theory, which is most certainly not based on classical geometry. Attempts to understand the structure of the full theory have been based in the past on re-summing the perturbation series, using the independence from the background expansion point. Unfortunately, the complexity of the theory and of the formal proof of independence from the expansion point have always been an obstacle to a deeper understanding of the general structure which hopefully underlies string theory. In a quite different direction, recently, it has been shown that, in certain regimes, string theory can be described by field theories which live on non-commutative spaces, most notably spaces defined by algebras of functions on an underlying ordinary manifold, with multiplication law given by associative *-products based, à la Kontsevitch, on Poisson structures on the manifold itself. These theories can be written as non-local field theories, are given as power-series expansions around classical solutions, and have a property which is quite similar to the property of independence of the expansion from the classical chosen solution. They have therefore some of the complexity of the full theory. On the other hand they are simple enough to give hope that a complete re-summation is possible.
In this talk I will describe this program in detail, both the results and the open questions. I will show that we can rewrite the theory quite algebraically as a theory written formally in terms of cyclic words built from (non-commuting) symbols X^a which represent the coordinates on the space. The correct theories then correspond to formal power-series in the X^a, which must represent "constant functions on this non-commutative space", with additional properties describing the independence from the chosen background. In some cases the classification of these functions is possible, and I will describe these solutions in some detail; in the general case it is still an open question.

Calabi-spin

Monday, May 5th, 2003
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Michele GRASSI (Pisa)
"Toric varieties and mirror symmetry"

Abstract: In the seminar we describe (part of) the content of our paper "Mirror symmetry and self-dual manifolds", math.AG/0301014. The main result that we shall describe is a way to interpolate geometrically between the large Kahler structure limit point in the Kahler moduli space of the anticanonical divisor in projective space of dimension n and a large complex structure limit point in the complex structure moduli space of its mirror partner. The interpolation is achieved by constructing a two dimensional family of smooth manifolds of real dimension 3(n-1)+2; for instance, for the quintic threefold we obtain a two dimensional family of 11 dimensional smooth manifolds. These manifolds are endowed with a structure, which we call a weakly self-dual structure (or WSD structure for brevity). The definition involves a Riemannian metric and three smooth 2-forms. The manifolds depend on two parameters. Qualitatively, what happens is that fixing the second parameter determines the "shape" of the limiting manifold, while if we let the first one go to infinity we get the large Kahler structure limit, and if we let it go to zero we get the large complex structure limit. Moreover, as the second ("shape") parameter goes to infinity, the limiting manifolds approach in a normalized Gromov-Hausdorff sense the anticanonical divisors of projective space of dimension n and their mirror duals.
The interpolating manifolds are constructed via a procedure which has a toric flavor to it, and starts from the reflexive polytope associated to projective space. The construction is completely explicit and in some sense "algebraic", although there is no clear algebraic analog to the notion of self-dual manifold (yet). The toric nature of the construction is reflected in the fact that the resulting manifolds have a free action by a real torus. Moreover, the limiting procedure involves a geometric deformation (reflected in a rescaling of the first parameter) which implies the rescaling of the metric on one of the two fibrations by a factor, and on the other fibration by the inverse of the same factor. As mentioned before, depending on the fact that we let the parameter go to zero or to infinity, we approach one or the other limit point of the deformation space. This description of mirror symmetry has some similarity with the conjectu-ral description of the mirror involution contained in the paper "Mirror symmetry is T-duality" by Strominger, Yau and Zaslow, although in a (possibly) unexpected way. Indeed, we do not build special La-grangian fibrations on the Calabi-Yau manifolds themselves near the limit points, as one would expect from that paper, but we end up with "special" tori fibrations on (higher dimensional) WSD manifolds, which approximate the Calabi-Yau ones only in Gromov-Hausdorff sense. The idea that this could be a way to avoid the complications associated with building special Lagrangian fibrations in the geometric approach to mirror symmetry is what led us to the definition of self-dual manifolds in the first place. The picture of the deformation space for our self-dual manifolds has a striking similarity with the con-jectural picture of the moduli space of superconformal field theories described by Kontsevich and Soibelman. This agreement is in accordan-ce with a more general conjectural picture, in which (weakly) self-dual manifolds can be used to build superconformal field theories via a process similar to a sigma-model construction. However, such a procedure has not yet been established in a mathematically rigorous way even for the more classical Calabi-Yau manifolds.

ball-Moebius

Monday, April 7th, 2003
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Gilberto BINI (Amsterdam)
"Galois rings and application to code theory"

Abstract: Error-correcting codes have always had a basic role in digital communication. Traditionally, their definition was based upon the algebra of finite fields; in the last years, howevere, the study of finite rings has shown several and, in some cases, unexpected applications to code theory. In this talk we shall talk of the properties of some finite rings, namely Galois rings. In particular, we shall discuss the possibility to construct new families of codes using techniques of algebraic geometry on these rings.

Calabi-spin

Monday, March 3rd, 2003
h. 14:30 - Room 1101
YOUNG ALGEBRA SEMINAR
Roberto INCITTI (IHES Paris)
"Two examples of growth function"

Abstract: We present two examples where the rate of growth of a formal language L gives information on an algebraic object associated to L. The first example is the growth of a finitely generated group G. If E is a finite generating subset of G, the length of an element g of G is the minimum length of a representation of g as a product of generators and f_E(n) as the function that gives the number of elements of G with length is less or equal to n. Gromov proved that if f_E(n) is bounded by a polynomial, then the group is nilpotent by finite. We will present this result and an outline of Gromov's proof, and an alternative (partial) combinatorial proof too. In the second part we will talk about the growth of the context-free languages. If L is a formal language, g_L(n) is the function that gives the number of elements of L whose length is less of equal to n; if L is context-free, then the generating series g_L(z) of the g_L(n)'s is algebraic. Flajolet showed that if g_L(z) is algebraic, then the growth function of L is either polynomial or exponential, and raised the question as to whether there exist context-free languages of intermediate growth, that is, greater than any polynomial function and smaller that any exponential one. We gave a negative answer to the question of Flajolet. We will make a survey on the problem and give an idea of the proof.

ball-Moebius

Monday, February 3rd, 2003
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Marina MONSURRÒ (EPF Lausanne)
"Adjoint groups and involution algebras"

Abstract: The deep links between linear algebraic groups and simple central algebras with involution date back - essentially - to two main trends. In a basic article (1960), A. Weil proved that the connected component in the automorphism group of an involution algebra can be identified, up to some restrictions we shall see in detail, with a semisimple linear algebraic group of adjoint type. Conversely, every semisimple linear algebraic group of adjoint type (but the ecceptional groups and the triality forms of D₄) can be obtained in this way. On the other hand, studying the representations of a semisimple linear algebraic group G, Tits (1966) obtained, by descent, representations of G in the group GL(A) of the invertible elements of a simple central algebra A, which is uniquely determined and endowed with a canonical involution. During the talk we shall see how this "correspondence" enables us to apply some recent results about involution algebras to rationality problems over adjoint groups.

Calabi-spin

Monday, January 13th, 2003
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Marco ZUNINO (Strasbourg)
"Turaev G-categories and their applications in topology and algebra"

Abstract: Recently Turaev introduced some new homotopic invariants for 3-dimensional manifolds. These invariants generalize the topological ones introduced by Reshetikhin and Turaev, constructed using knot theory and the theory of braided categories (usually categories of representations of quasitriangular Hopf algebras). A key step of the new theory is the notion of crossed G-coalgebra (where G is a group). This is a family of algebras {H_g}_{g in G} endowed with sort of an overall comultiplication and of isomorpims among members of the family indexed by elements which are conjugate within the group (along with some consistency axioms). In this framework one can generalize the notions of R-matrix (quasitriangular G-coalgebra) and of ribbon. However, the category of reresentation of a quasitriangular G-coalgebra does not have the structure of braided category, but satisfies instead the more general axioms of a crossed G-category.
After a brief description of the topological invariants of Reshetikhin and Turaev and of the techniques they use (Kirby moves, braided categories, quantum groups, etc.), we shall shortly introduce the new homotopical invariants, hence fe shall focus upon the corresponding algebraic and categorial structures. The talk will finish with a brief description of the results on the generalizzation of Drinfeld's double, and also sketching some of the many problems which still stand open.

rotating-knot

Monday, December 16th, 2002
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Lorenzo TORTORA DE FALCO (Roma Tre)
"On the logic structure of computation"

Abstract: Starting from the Curry-Howard correspondence between proofs and programs, we shall explain how logical proofs are a model of computation. Then we shall define the linear logic (introduced by J.-Y. Girard in 1987), which provides a "logic" status to the structure rules (i.e. to the operations of duplication and deletion). This change of viewpoint has had surprising consequences: among these, one of the most important is the introduction of proof-nets, which provide a "geometrical" representation of the computation. We shall introduce the proof-nets, and we shall present some contributions of linear logic to the (wide) area of research which is called "Logic in Computer Science". In particular, we shall explain how the proof-nets enables to characterize in a purely logical way the class of functions which are computable in elementary time and the class of functions which are computable in polynomial time (by a deterministic Turing machine).

ball-Moebius

Monday, November 25th, 2002
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Clara FRANCHI (U. C. Brescia)
"Permutation groups of finite type"

Abstract: Let G be a group of permutations on a (not necessarily a finite) set X. If every non-trivial element of G moves all points of X but for (at most) a finite number, then one says that G is a cofinitary group. The set K of the numbers of fixed points of the non-trivial elements of G is called "the type" of G. If K is a finte set, G is said to be finite. The main problem in the study of finite type permutation groups is to get information on the structure of the group out of knowing the type. For instance, it is known that if the type is the set {0}, then the group is regular.
After a review of the most interesting results obtained in the last years on this topic, we shall dwell more in detail upon the case when the type is given by only one element, that is to say all non-trivial elements have the same number of fixed numbers. For these groups, we shall state some structure theorems, both in the finite and infinite case. In particular we shall completely classify the finite permutation groups in which every non-trivial element has the same number of fixed points and the number of orbits is minimal.

Calabi-spin

Wednesday, June 19th, 2002
h. 15:00 - Room 1201
Zoran RAKIC (Belgrade)
"Quantum dynamics on a noncommutative space with quadratic Lagrangian"

Abstract: We consider a quantum mechanical system on a non-commutative 3-dimensional space, described by a full quadratic Lagrangian. We use the Feynman's path integral method to find a connection between the propagator of our non-commutative quantum mechanical system and the propagator of the naturally associated quantum mechanical system on the corresponding commutative space.

ball-Moebius

Monday, May 13th, 2002
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Flavio D'ALESSANDRO (Roma "La Sapienza")
"Questions of algebraic combinatorics in finite type monoids"

Abstract: In the theory of formal codes, some problems of optimality are strictly related to the following conjecture by Schutzenberger (from the late '50's): every formal code can be converted into a prefix code which bears the same cardinality, up to permuting the letters within the words of the code. The conjecture is true for some important families of codes, like that of very pure codes, but in general is false, as proved by P. Shor in 1984; nevertheless, it stands open for finite and maximal codes. One can then formulate the conjecture as follows:
Conjecture: Let X be a finite maximal code. Then there exists a prefix code X' which is commutatively equivalent to X.
In this talk we shall present some important results about Schutzenberger's conjecture, and the links of the latter with other open problems, like - for instance - that of decidibility of the completion of a finite code.

Calabi-spin

Monday, April 8th, 2002
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Marina AVITABILE (L'Aquila)
"Thin graded Lie algebras and thin pro-p-groups"

Abstract: A graded Lie algebra L, whose i-th homogeneous will be denoted by L_i, is said to be "thin" if L₁ has dimension two and the following covering property holds: for every positive integer i and every non-zero v in L_i, one has [v, L_i] = L_i+1.
Thin algebras have been initially introduced as algebras associated to thin pro-p-groups, with respect to their lower central series. A pro-p-group is said to be "thin" if the quotient with respect to the Frattini subgroup has order p², and moreover every normal subgroup is contained between two suitable consecutive terms of the lower central series. An important examle of thin pro-p-group is the so-called Nottingham group.
The aim of this talk is to present the framework in which thin algebras and thin pro-p-groups fit in. Moreover, I shall offer an overview of the main results obtained for thin algebras of infinite dimension over positive characteristic field.

ball-Moebius

Monday, March 11th, 2002
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Giovanna CARNOVALE (Padova)
"Brauer groups of a Hopf algebra"

Abstract: The definition of Brauer grup of a field has been generalized in several ways: the Brauer group of a commutative ring, the Brauer group of a Z-graded ring or of a Z₂-graded ring, or in general of a G-graded ring, with G being a finite Abelian group. There exists a construction by F. Van Oystaeyen and Y. Zhang which embodies all the others, that is the Brauer group of a braided category. In particular, we shall present the case when the above categoy is a category of modules of a finite-dimensional quasitriangular Hopf algebra. The functoriality of the construction guarantees that some equivalences between categories of Hopf algebra representations induce isomorphisms betweeen the corresponding Brauer groups. We shall show how this can be used in order to specifically computate the Brauer group of some Hopf algebras.

Calabi-spin

Monday, February 11th, 2002
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Mario MARIETTI (Roma "La Sapienza")
"Polinomi di Kazhdan-Lusztig per riflessioni booleane in sistemi di Coxeter lineari"

Abstract: In order to study certain representations of the Hecke algebra associated to a Coxeter system (W,S), Kazhdan and Lusztig introduced two families of polynomials indixed by pairs of elements in W, the R and the P polynomials. The former are important mainly because their knowledge yields information about the latter, which are the very Kazhdan-Lusztig polynomials and from which one can construct some W-graphs and hence the KL-representations. However, the explicit computation of the polynomial P_u,v is very difficult, and it makes use of a recursive formula which depends on the whole interval [u,v] as a poset with the Bruhat order, on the group algebra and on the Kazdhan-Lusztig polynomials indexed by pairs of elements in the interval. After giving the definitions and the basic results of the theory, I shall give a closed formula for R_u,v and one for P_u,v when v is not greater than a boolean reflection t. These two formulas respectively generalize a conjecture by Brenti and one by Haiman; also, they enable to prove, in the case of elements which are not greater than boolean reflections, the combinatorial invariance conjectured by Dyer and by Lusztig.

ball-Moebius

Monday, January 15th, 2002
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Francesco CHIERA (Roma "La Sapienza")
"Some aspects of the theory of theta series"

Abstract: The aim of this talk is to elementary recall some aspects of the theory of theta series. In particular, I shall point out the relevance of theta series associated to positive definite quadratic forms in the framework of the theory of (Siegel) modular forms. More in detail, I shall discuss some questions connecteed to the so-called "basis problem". The latter, which is undoubtedly one of the basic problems of the theory, consists (in very rough terms) in characterizing the modular forms which can be obtained as linear combination of theta series. Finally, I shall present some of the interesting relations which hold between the theory of theta series and that of error-correcting codes.

rotating-knot

Monday, December 10th, 2001
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Domenico FIORENZA (Roma "Tor Vergata")
"Graphs, algebras and PDE's"

Abstract: How many rational curves of degree d passe through 3 d - 1 points of the projective plane?
The first cases are simple: only one straight line passes through 2 points, and only one conic through 5 points. But the case d = 3 already presents a much less trivial answer: trhough 8 points of the plane there pass 12 rational cubics. In order to answer the question for any degree d, we shall follow - try to follow - Kontsevich and Manin in an area of mathematics where to a graph it corresponds a differential equation, which in turns defines a field of algebras. The talk will be completely elementary, and can be attended by any student who had basic knowledge of homology and cohomology.

Calabi-spin

Wednesday, December 5th, 2001
h. 14:30 - Room 1201
Aleksandar LIPKOVSKI (Belgrade)
"Newton polyhedra: history, combinatorics, applications"

Monday, November 26th, 2001
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Robert AEHLE (Bern)
"Degenerations for self-injective algebras"

Abstract: Let A be a connected representation finite self-injective algebra. According to Zwara, some canonical partial orders on the isomorphism classes of d-dimensional A-modules can only differ if the stable Auslander-Reiten quiver of A is of Dynkin type D_3m for some m greater than 1.. We study the minimal degenerations of M which are not given by extensions in these exceptional cases.

Calabi-spin

Monday, June 25th, 2001
h. 14:30 - Room 1201
Alessandra FRABETTI (Lausanne)
"Feynman diagrams, trees and series"

Abstract: During the last fifty years, the perturbative quantum field theory has been coping with the problem of renormalization of integrals associated to Feynman diagrams, with analytical methods connected to the famous BPHZ formula. Recently, Connes and Kreimer found an algebraic interpretation in the case of scalar fields, in terms of commutative Hopf algebra directly built upon Feynman diagrams. The dual group is strictly related to that of formal series under composition. For scalar field theories, the Hopf algebra can no longer be commutative, and the renormalization is equivalent to an unexpected non-commutative version of the algebra of polynomial functions on the group of formal series. Moreover, in the case of quantum electrodynamics there exists another perturbative method, based upon planar binary trees instead of Feynman diagrams. The renormalization, in this case, yields a non-commutative Hopf algebra over trees. What can be said then about series expanded over trees? This talk will address, among others, this question.

ball-Moebius

Friday, June 22nd, 2001
h. 16:00 - Room 1101
Jovo JARIC (Belgrade)
"Some recent advances in continuum mechanics"

Wednesday, June 6th, 2001
h. 16:00 - Room 1101
Dragana TODORIC (Belgrade)
"On the KPRV determinants"

Abstract: In 1961, Kostant proved freeness of universal enveloping algebra over its centre. He also obtained certain multiplicity formula which led Partha-sarathy, Ranga Rao and Varadarajan (1967) to define and factorize a family of determinants, which we call PRV determinants. Shortly afterwards Kostant (1971) described certain analogues of PRV determinants, which we call KPRV determinants, by replacing a Cartan subalgebra with a Borel subalgebra. This had important applications to the question of the irreducibility of principal series modules. The goal of the talk is to present more recent approach to classical KPRV determinants and analogous results related to quantized universal enveloping algebras. This research is due to Joseph, Letzer and Todoric. Note that Gorelik and Lanzmann completed similar research for reductive Lie superalgebras.

ball-Moebius

Wednesday, May 30th, 2001
h. 11:30 - Room 1201
Neda BOKAN (Belgrade)
"Induced representations of some holonomy groups"

Abstract: The holonomy group of a manifold M is a very powerful tool for studying the curvature tensor, corresponding to either a Riemannian metric g on M or a torsion free connection D defined on M. Moreover, the holonomy group carries a considerable amount of information about the differential geometry and the topology of the manifold. The main purpose of this lecture is to give an overview on the previously mentioned facts. Especially, we pay attention to the consequences of induced representations of holonomy groups into vector space of the corresponding curvature tensors. We include in this considerations holonomy groups of Riemannian metrics, as well as of torsion free connections.

Calabi-spin

Monday, May 14th, 2001
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Emanuela DE NEGRI (Genova)
"Groebner bases, Pfaffian ideals and Koszul algebras"

Abstract: The theory of Groebner bases came out of computational exigences, but in the last years it has found wider and wider applications to several topics of Commutative Algebra. The aim of this talk is to give the basic notions of this theory, and to show how it applies to the study of ideals generated by Pfaffians, and more in general of the determinantal rings. The latter have already been widely studied with other methods, but the application of Groebner bases made it possible to obtain new results. Koszul algebras have recently drawn the attention of well known researchers for the relevant applications and relations they have in Commutative Algebra, Computational Algebra, Algebraic Geometry and in the theory of Quantum Groups. The use of Groebner bases permits to find wide classes of Koszul algebras.

ball-Moebius

Saturday, May 5th, 2001
h. 9:30 - Room 1201
Workshop "TV2001 - Quantum Groups and their neighborhoods"
Domenico FIORENZA (Pisa)
"Feynman diagrams"

Friday, May 4th, 2001
h. 15:00 - Room 1201
Workshop "TV2001 - Quantum Groups and their neighborhoods"
Nicola CICCOLI (Perugia)
"Lie algebroids"

Friday, May 4th, 2001
h. 11:30 - Room 1201
Workshop "TV2001 - Quantum Groups and their neighborhoods"
Concettina GALATI (Roma "Tor Vergata")
"Quantum groups and link invariants"

Abstract: We shall give a brief account of Turaev's method (1988) to generate knot and link invariants starting from solutions of Yang-Baxter equations.

Calabi-spin

Friday, May 4th, 2001
h. 9:30 - Room 1201
Workshop "TV2001 - Quantum Groups and their neighborhoods"
Giovanna CARNOVALE (Padova)
"Classification of triangular Hopf algebras"

Thursday, May 3rd, 2001
h. 15:00 - Room 1201
Workshop "TV2001 - Quantum Groups and their neighborhoods"
Rita FIORESI (Bologna)
"Quantum groups and deformation-quantization"

Thursday, May 3rd, 2001
h. 11:30 - Room 1201
Workshop "TV2001 - Quantum Groups and their neighborhoods"
Federico DE VITA (Firenze)
"Deformation quantization following Kontsevich"

Abstract: We shall give a brief overview of Kontsevitch's first paper (1997) on deformation quantization.

ball-Moebius

Thursday, May 3rd, 2001
h. 9:30 - Room 1201
Workshop "TV2001 - Quantum Groups and their neighborhoods"
Paolo CARESSA (Firenze):
"Poisson, Hochschild (and cyclic?) homology"

Monday, April 23rd, 2001
h. 15:45 - Room 1201
Tzachi BEN-ITZHAK (Bar-Ilan)
"Introduction to braid groups and their applications"

Abstract: Braid groups, the subject of this talk, are a natural generalization of Coxeter groups: their importance is increased by the fact that in last years they proved very interesting for applications in different areas of mathematics. The talk will be essentially an introductory one.

ball-Moebius

Monday, April 9th, 2001
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Riccardo MURRI (SNS Pisa)
"Kontsevich model for 2D gravity"

Abstract: Witten conjectured that the generating series of the intersection numbers of the moduli space of stable curves satisfies a suitable hierarchy of differential equations. Kontsevich has related this generating series with the asymptotic expansion of a matrix integral. The proof of this fact mainly involves the asymptotic expansion of matrix integrals by means of Feynman diagrams and a particular triangulation of the moduli space of smooth n-pointed curves obtained by exploiting the properties of quadratic differentials of Riemann surfaces.

Calabi-spin

Monday, March 12th, 2001
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Fabio STUMBO (Ferrara)
"Cohomology of Artin groups"

Abstract: Artin group generalize the classical braid group: thus they form a widely studied category of groups in the mathematical literature. Among their properties under exam, there is of course their cohomology. In this talk we shall cope with this topic.
In particular, let us consider the ring R of rational Laurent polynomials in one indeterminate q, and the Artin group G_W associated to an irreducible Coxeter group W. We shall show what is the cohomology of G_W with coefficients in R_q, where R_q is the G_W-module given by the action on R defined by applying every standard generator of G_W in the multiplication by -q.

ball-Moebius

Monday, February 12th, 2001
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Nicoletta CANTARINI (Padova)
"Z-graded Lie superalgebras of finite growth and infinite depth"

Abstract: The theory of Lie superalgebras is essentially due to Victor Kac, who in 1977 classified the simple Lie superalgebras of finte dimension over an algebraically closed field of zero characteristic, and in 1998 classified the Z-graded Lie superalgebras of infinite dimension and finite depth. The goal of this talk is to introduce the construction of Z-graded Lie superalgebras of finite growth and infinite depth, to present two classes of examples, and to describe the problem of classification of these superalgebras in relation with the already known case of the contragradient superalgebras.

Calabi-spin

Monday, January 15th, 2001
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Marco D'ANNA (Catania)
"Analitically unramified one-dimensional rings"

Abstract: Let R be a one-dimensional, reduced, semilocal Noetherian ring; let R' be the integral closure of R in its total fraction ring, and let R" be its completion for the topology induced by the Jacobson radical. The ring R is called analitically unramified if R" or, equivalently, if R' is a finite type R-module. Under these assumptions, one can associate to R a semigroup (contained in N^d, where d is the number of minimal primes of R"): from the study of this semigroup one can get many information about the initial ring (if in addition R is residually rational).
An example of the usefulness of these techniques is given by the study of multiplicity sequences of R (via the - more subtle - notion of multiplicity forest), obtained from subsequent blowups of the Jacobson radical of R and of the extension rings thus obtained. This study is motivated by the fact that the multiplicity sequences provide an important system of invariants in the study of singularities of algebraic curves. In particular, it is possible to give a numerical characterization of the forests of vectors in N^d which are multiplicity forests associated to a ring. All these results come from a joint work with V. Barucci and R. Froeberg.

rotating-knot

Monday, December 4th, 2000
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Alessandro D'ANDREA (Roma "La Sapienza")
"An introduction to pseudoalgebras"

Abstract: The structure of (Lie) pseudoalgebra is an axiomatization of the singular part of the OPE (=operator product expansion) of chiral fields in a field theory. The language of pseudoalgebras enables also to describe in a compact manner the structure of a special class of infinite-dimensional Lie algebras.
We shall give an introduction to the theory of pseudoalgebras, explaining their origin and what are the basic tools and results in the study of their structure.

ball-Moebius

Monday, November 13th, 2000
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Daniele GEWURZ (Padova)
"Parker vectors of permutation groups"

Abstract: We shall discuss some methods to study a permutation group (thought of a a subgroup of a symmetric group) starting from the cyclic structures of its elements.
Inspired by questions of computational Galois theory, people realized that it is interesting to consider the action of a finite permutation group over the set of the cycles which occur in the decomposition of its elements into disjoint cycles. The numbers of orbits on the set of 1-cycles, of 2-cycles etc. form the "Parker vector" of the group. We shall show some properties of Parker vectors, examples of the computation of these vectors for some groups or classes of groups, and what one can recover about the group from the sole knowledge of the vector (for instance, the Prker vector characterizes the symmetric and the alternating groups). We shall also present some tentative generalizations, regarding the definition of the vector or the extension of this concept to infinite groups.

Calabi-spin

Monday, May 8th, 2000
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Francesca TARTARONE (BNL Multiservizi S.p.A.)
"Proprietà moltiplicative di alcuni anelli di polinomi"

Abstract: Let D be a domain with quotients field K. one calls "ring of integer-valued polynomials over D" the set of all polynomials (in one indeterminate) with coefficients in K which take values in D when evaluated on D itself. Very simple examples of these polynomials are Newton binomials. I shall give an overview on the hystory of these polynomials, and on how their study have followed several different directions in the last years, talking more precisely about the problems concerning commutative algebra.

ball-Moebius

Monday, April 10th, 2000
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Rocco CHIRIVÌ (SNS Pisa)
"LS algebras and Schubert varieties"

Abstract: LS algebras are a generalization of doset algebras and Hodge algebras. The idea of a basis of monomials and "straightening relations" dates back to Hodge's study of Grassmann variety and Schubert cycles. From the use of these straightening relations applied to Schubert cycles and determinantal varieties De Concini, Einsenbud and Procesi isolated the axioms for a Hodge algebra. Next comes the definition of doset algebras introduced to prove Cohen-Macaulayness and normality for Schubert varieties of a semisimple, simply connected Chevalley group and a maximal parabolic subgroup of classic type. The restriction of classical type limits the bonds, some sort of ``multiplicity'', to 2 whereas Hodge algebras have all such multiplicities equal to 1.
Being able to handle arbitrary multiplicities has taken almost twenty years and the starting point for this development was the introduction by Littelmann of Lakshmibai-Seshadri paths basis for representations of Kac-Moody algebras. Then Littelmann, Lakshmibai and Magyar have found some relations for LS paths monomials in the coordinate ring of (the cone over) a Schubert variety. These relations have proved compliant with our straightening relations for LS algebras and have enabled the application to Schubert varieties. Our main result is that any Schubert variety (for any parabolic subgroup) admits a flat deformation to a union of normal toric varieties. We give a new proof of Cohen-Macaulayness and normality in the spirit of the standard monomial theory. Finally we compute the degree of the embedding of the Schubert varieties with a formula generalizing a classical Seshadri result.

Calabi-spin

Monday, March 13th, 2000
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Elena SOVERCHIA (SNS Pisa)
"Relative structure of the rings of integers"

Abstract: Let N be a finite extension of an algebraic number field k, and let O_N andO_k respectively be the rings of integers of N and of k. The ring O_N is a torsion-free O_k-module of rank [N:k], hence there exists a fractional ideal I_N in k such that O_N is isomorphic to the direct sum of O_k^[N:k]-1 and I_N.
The class [I_N] in the class group Cl(O)_k is called the Steinitz class of O_N. The ring O_N is a free O_k-module if and only if the ideal I_N is principal. In this case we say that the ring O_N has a relative integral basis (RIB) over O_k.
In the first part of the talk we give sufficient and/or necessary conditions so that an extension N/k has a RIB. We also expose the principal results obtained for extensions of small degree.
In the second part, we fix a number field k and a finite group G. A classical problem consists in characterizing the set of those classes in Cl(O)_k which are Steinitz classes of Galois extensions N/k whose Galois groups are isomorphic to G. In particular one is interested in studying if this set is a group. We expose the principal results obtained for the abelian extensions and we describe the few known results for the non abelian ones.

ball-Moebius

Monday, February 14th, 2000
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Federico DE VITA (Firenze)
"Deformation theory and frobenius manifolds"

Abstract: We recall the basic concepts of deformation theory in the terms in which it has been recently studied (we refer to works of M. Kontsevich and M. Manetti), i.e. using differential graded Lie algebras. We then introduce the concept of a Frobenius supermanifold both in the geometric and in the formal case, and show how such a manifold is a deformation of an algebra structure that preserves a given metric. We find the DGLA governing these deformations. In this context the problem of semisimplicity arises, in connection with the Virasoro conjecture. We therefore study how semisimple superalgebras behave and deduce some implications for semisimple Frobenius supermanifolds.

Calabi-spin

Monday, January 17th, 2000
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Paolo CARESSA (Firenze)
"The algebra of Poisson brackets"

Abstract: We shall discuss the Hamiltonian formalism from the algebraic point of view, defining Poisson brackets and Hamiltonian fields on associative algebras. The examples will abstract ones, yet looking at Geometry. We shall also introduce the bases of differential calculus on Poisson algebras and the corresponding invariants, which besides of their geometrical applications are also interesting in themselves (like Poisson cohomology).

rotating-knot

Monday, December 13th, 1999
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Andrea MAFFEI (Roma "La Sapienza")
"Quiver varieties"

Abstract: Quiver varieties have been introduced and used by H. Nakajima to give a geometric construction of the integrable representations of Kac-Moody algebra with symmetric Cartan matrix. Descriptions of this kind have proved very useful in other cases to understand some problems in pure representation-theoretic terms. In this case, for instance, the construction of Nakajima provides a distinguished, canonical basis of the representations. But the geometry of these varieties so far has been only partially understood. In this talk I shall give an overview of the main results.

ball-Moebius

Thursday, June 14th, 1999
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Gilles HALBOUT (Strasbourg)
"Formality conjectures and deformation"

Abstract: Let M be a symplectic manifold. Connes, Flato and Sternheimer constructed an invariant \phi in the cyclic cohomology of M for any closed star-product. They compute this invariant in the de Rham complex of M when M=T*V. We complete this result by computing the image of \phi in the de Rham complex for any symplectic manifold and any star-product and we show: 1) that this invariant is a complete invariant of star-products; 2) how this invariant is related to the general classification of Kontsevich. The proof uses the Riemann-Roch theorem for periodic cyclic chains of Nest-Tsygan. Finally, we show that generalisation of this invariant to any star-product over a Poisson manifold can be made by generalizing Kontsevitch formality conjectures.

Calabi-spin

Friday, May 14th, 1999
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Georges PAPADOPOULO (Basel)
"Combinatorics associated to modular representations"

Abstract: The study of finite dimensional GL_n(k)-modules is still a very open problem, when the characteristic of k is positive. There is a conjectural formula due to Lusztig when n is not less than p, which is proved by Andersen-Jantzen-Soergel for p much greater that n, and which is valid for all the finite dimensional modules.
In this talk, I shall give a character formula (joint work with O. Mathieu) for some finite dimensional simple GL_n(k)-modules. Contrary to the case of Lusztig's formula, this one is a combinatorial in nature (that is, the weight spaces are given as a sum of positive numbers): in fact, the combinatorics is described in terms of semi-standard Young tableaux. Moreover, this formula is stable, that is, it gives results even when n tends to infinity, but only for a family of finite dimensional simple modules.
After having presented the formula, we will define the category of tilting modules (in the wider context of quantum groups) and give its main properties. The proof of the formula is based on a tensor product formula (Verlinde formula) for tilting modules due to Georgiev-Mathieu in the algebraic context. We will shortly describe the proof of the formula and give some knew reformulation of related known conjectures on tilting modules (joint work with H.H. Andersen). We will eventually present some applications to the character of infinite gl_n(C)-simple modules or discuss about some related problems in representation theory.

ball-Moebius

Monday, April 12th, 1999
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Aldo CONCA (Genova)
"Koszul algebras"

Abstract: Let K be a field, and let R = K[x₁,\dots,x_n]/I be a standard K-algebra, with I homogeneous for the grading such that the x_i's have degree 1. When studying R-free resolutions of graded R-modules, the resolution of K as an R-module plays a central role. For instance, every (graded, fintely generated) R-module has a finte resolution over R if and only if K-anto has a finite resolution over R, and this occurs if and only if R is regular (which in the present case means that R is a ring of polynomials). When R is not regular, one can consider some numerical invariants, related to the resolution of K as an R-module, which in some sense describe the complexity degree of the resolution, hence giving an estimate of how far they are from the regular case.
One of these invariants is the Poincaré series of R. In the '60's J. P. Serre conjectured that this series be always rational. In the early '80's D. Anik proved that the conjecture is false, and recently J. E. Roos and B. Sturmfels have proved that the non-rationality of the Poincaré series can show up even in relatively "simple" rings such as the coordinate rings of monomial curves.
Another measure of the complexity of the resolution of K is given by the degree of the syzygies. The algebra R is called Koszul if all its syzygies are linear, or - more precisely - generated by degree one elements. Koszul algebras have been introduce in the early '70's by S. Priddy, and have been intensively studied since then. One of their more important properties is to have a rational Poincaré series. In general, the problem of determining whether an algebra is Koszul or not is very hard, and it cannot be solved via symbolic computation.
In this talk I shall present a new approach to the study of Koszul algebras, as a result of joint work with De Negri, Rossi, Trung and Valla. I shall introduce the concept of Koszul filtration, and I shall explain how this enables to prove that some classes of algebras are Koszul. I shall talk about relations among some special filtrations - the so-called "Koszul flags" - and Groebner bases, and of the problem of classification the universally Koszul algebras. Moreover, I shall present the problem of dettermining whether the ring defined by the apolar forms to a cubic be Koszul (or not).

Calabi-spin

Monday, March 8th, 1999
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Giovanni GAIFFI (SNS Pisa)
"De Concini-Procesi models of arrangements and symmetric group actions"

Abstract: Recently, De Concini and Procesi described a method to construct model of a subspace arrangement in Cⁿ. This construction is carried on through a series of blow-ups of varieties which also are models of arrangements. This allows to compute in a recursive way the cohomology ring of the model - which is free Z-module - and to construct a Z-basis, which can be described in purely combinatorial terms introducing a family of oriented graphs.
In this talk we shall consider in particular the arrangement of braids, that is the arrangeament in Cⁿ given by the coordinate hyperplanes {x_i - x_j = 0}. The geometrical importance of it comes from the fact that one possible projectivization of it is isomorphic to the moduli space M_0,n+1 of (n+1)-pointed curves of genus 0. Moreover, one of the projective models of De Concini-Procesi associated to the braid arrangement is isomorphic to the Mumford-Deligne compactification of M_0,n+1, i.e. to the moduli space M_0,n+1 of stable, (n+1)-pointed curves of genus 0.
Thus we have two geometrical interpretations of the space M_0,n+1; this enables us to study the cohomology rings H^*(M_0,n+1, Z) and H^*(M_0,n+1, Z) from two different viewpoints: the point of view of model theory points out an action of the symmetric group S_n which permutes the elements of the Z-basis; the modular interpretation reveals an extended action of the symmetric group S_n+1.
Using together these two actions, one can describe H^*(M_0,n+1, Z) and H^*(M_0,n+1, Z) as S_n-modules and as S_n+1-modules, translating the geometrical properties in combinatorial terms and studying particular functions defined over graphs.

ball-Moebius

Monday, February 8th, 1999
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Anna GUERRIERI (L'Aquila)
"Multilinear forms, Jacobian ideals and primary decompositions"

Abstract: Since the classical work of Emmy Noether, one of the basic problems in Commutative Algebra has been to find the primary decomposition of ideals in a Noetherian ring R. Indeed, finding primary decompositions is a step towards identifying zero divisors, ideal containment, projective dimension and Rees valuations; it is also a step towards building regular sequences, determining Cohen-Macaulayness, and understanding the homological properties of modules and ideals.
We study the primary composition of the Jacobian ideals of generic trilinear forms.
For three-dimensional arrays, this amounts to studying the Jacobian ideal of a form in three sets of variables which is of degree one in each set. Recall that a Jacobian ideal is the ideal generated by all the partial derivatives with respect to each of the variables.
This type of analysis bridges to the theory of hyperdeterminants. In fact, it is well-known that the primary decomposition of a Jacobian ideal determines whether the hyperdeterminant of the corresponding tensor given by the coefficients of the form is non-zero.
The first study in this direction was done by Boffi, Bruns and Guerrieri, who obtained the minimal associated primes of the Jacobian ideals of a large class of trilinear forms satisfying some combinatorial relations.
In this talk we shall show that we can relax the combinatorial restrictions, and yet we still obtain the minimal primes of the Jacobian ideals of the corresponding trilinear form. Furthermore, we obtain the minimal components and the radical of the Jacobian ideal. In some cases we find the complete primary decomposition (some suggestive calculations are emerging also by using the symbolic computer algebra package Singular).
Our methods do not use any combinatorics. Instead, our main tool is given by the concept of 1-generic matrices, a generalization of Eisenbud's 1-generic matrices. We develop a basic theory of 1-generic matrices in this more generalized sense.
The structure of the Jacobian ideal under observation actually depends upon the properties of the three matrices of linear forms obtained by taking the mixed second derivatives of the trilinear form. When the underlying field is algebraically closed the 1-genericity of either one of these matrices allows us to deduce information on the associated primes of the Jacobian ideal. The 1-genericity itself can be detected by computing the height of the maximal minors of some of these matrices.
Further it can be observed that the hyperdeterminant of a three-dimensional tensor of boundary format is non-zero if and only if the corresponding trilinear form satisfies the 1-generic conditions mentioned above.
The family of trilinear forms described by Boffi, Bruns and Guerrieri is just one example of this new environment. In fact, when the underlying field is algebraically closed, there exists a non-empty Zariski-open set where the coefficients of the trilinear form can vary while the form keeps satysfying the 1-genericity conditions.
It seems natural to try to expand these lines of research towards the multilinear case, involving techniques proper of the theory of quivers.

Calabi-spin

Monday, January 11th, 1999
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Alessandra FRABETTI (Strasbourg)
"Leibniz Algebras and Dialgebras"

Abstract: The Chevalley-Eilenberg boundary operator on the exterior powers of a Lie algebra g can be lifted to the tensor powers, yielding a new chain complex. The homology of this new complex, called LEIBNIZ HOMOLOGY, produces new invariants for Lie algebras and, more generally, it makes sense on a `non-antisymmetric' version of Lie algebras, called LEIBNIZ ALGEBRAS.
A powerful tool to investigate the Lie homology of a Lie algebra g is to reduce it to the homology of associative algebras, using the functor Lie --> Alg, which maps g to its universal enveloping algebra U(g), and its adjoint functor Alg --> Lie which defines a Lie bracket [a,b] = a b - b a from an associative product. For Leibniz algebras there exists as well an "associative" version, called DIALGEBRAS, and results similar to the classical ones are obtained to relate Leibniz and dialgebra homology theories. We shall present some examples of Leibniz algebras and dialgebras, and a summary of the main results on Leibniz and dialgebra homology theories.

rotating-knot

Monday, December 14th, 1998
h. 14:00 - Room 1201
YOUNG ALGEBRA SEMINAR
Giuseppe JURMAN (Trento)
"Graded modular Lie algebras of maximal class"

Abstract: Lie methods play an essential role to develop classification results in the study of p-groups of bounded coclass (and , in particular, of maximal class), hence it makes sense to consider analogous problems for Lie algebras. Several years ago, it has been proved that there are many Lie algebras of maximal class in zero characteristic, while of one moves to graded algebras there exists, in practice, only one. In the modular case, for every prime p there exist non-countably infinitely many graded algebras of maximal class. Nevertheless, for these algebras one can obtain a classification, which turns to be different according to whether the characteristic of the ground field is odd or not.

(top of the page)

- ARTS - Algebra and Representation Theory Seminar

(ART home page) in Roma "Tor Vergata" (ART home page)

Friday, December 5th, 2025 h. 16:00 - Room "Roberta Dal Passo" Valerio MELANI [t.b.c.] (Università di Firenze) "TBA"

Friday, December 5th, 2025 h. 14:30 - Room "Roberta Dal Passo" Chris MILIONIS (Newcastle University) "TBA"

Friday, November 21th, 2025 h. 16:00 - Room "Roberta Dal Passo" Bernard LECLERC (Université de Caen) "TBA"

Friday, November 21th, 2025 h. 14:30 - Room "Roberta Dal Passo" Matilde MACCAN (Ruhr-Universität Bochum) "TBA"

Friday, November 7th, 2025 h. 14:30 - Room "Roberta Dal Passo" Lucia BAGNOLI ("Sapienza" Università di Roma) "TBA"

Friday, November 7th, 2025 h. 14:30 - Room "Roberta Dal Passo" Alessio CIPRIANI (Università di Verona) "TBA"

Friday, October 24th, 2025 h. 16:00 - Room "Roberta Dal Passo" Giovanni CERULLI IRELLI ("Sapienza" Università di Roma) "TBA"

Friday, October 24th, 2025 h. 14:30 - Room "Roberta Dal Passo" Antonio MITI ("Sapienza" Università di Roma) "Construction and Reduction of the Lie infinity Algebra of Observables associated with a BV-Module"

Friday, October 10th, 2025 h. 16:00 - Room "Roberta Dal Passo" Jiefeng LIU (Northeast Normal University, Changchun) "Cohomology of restricted Poisson algebras in characteristic 2"

Friday, October 10th, 2025 h. 14:30 - Room "Roberta Dal Passo" Luca CASARIN ("Sapienza" Università di Roma) "The factorizable Feigin-Frenkel center"

Friday, September 26th, 2025 h. 16:00 - Room "Roberta Dal Passo" Francesca PAGANELLI ("Sapienza" Università di Roma) "Quantum cluster algebras and representations of shifted quantum affine algebras"

Friday, September 26th, 2025 h. 14:30 - Room "Roberta Dal Passo" (joint session with the "Topology Seminar") Alberto AVITABILE (Università di Roma "Tor Vergata") "E∞-algebra structure on singular cochains"

Friday, June 20th, 2025 h. 16:00 - Room "Roberta Dal Passo" Nicolas RESSAYRE (Université Lyon 1) "An algorithm to compute the moment cones"

Friday, June 20th, 2025 h. 14:30 - Room "Roberta Dal Passo" Thomas GERBER (Université Lyon 1) "Atomic length on Weyl groups"

Thursday, June 5th, 2025 (( beware of the change of day and room!!! )) h. 14:30 - Room 1200 (Biblioteca Storica) N.B.: joint session with the "Topology Seminar" Waclaw MARZANTOWICZ (University of Poznań) "Reeb graphs and description of homomorphisms onto the free groups"

Friday, May 23rd, 2025 h. 14:30 - Room "Roberta Dal Passo" Santosha Kumar PATTANAYAK (Indian Institute of Technology - Kanpur) "Uniqueness of branching and unique factorization of tensor products of typical representations of Lie superalgebras"

Friday, April 11th, 2025 h. 14:30 - Room "Roberta Dal Passo" Tommaso SCOGNAMIGLIO (Universität Heidelberg) "PGL(2)-character varieties and Langlands duality over finite fields"

Friday, March 28th, 2025 h. 14:30 - Room "Roberta Dal Passo" Filippo AMBROSIO (Friedrich-Schiller-Universität Jena) "Étale geometry of Jordan classes closures"

Friday, March 14th, 2025 h. 14:30 - Room "Roberta Dal Passo" Lydia GÖSMANN (Ruhr-Universität Bochum) "Nakajima varieties of quivers"

Friday, February 28th, 2025 h. 16:00 - Room "Roberta Dal Passo" Damien SIMON (Université Paris-Saclay) "Chiral differential operators on a reductive group and representation theory"

Friday, February 28th, 2025 h. 14:30 - Room "Roberta Dal Passo" N.B.: joint session with the "Topology Seminar" Andrea PIZZI (Università di Roma "Tor Vergata") "(Multi-)Simplicial methods for Configuration Space Recognition"

Friday, February 14th, 2025 h. 16:00 - Room "Roberta Dal Passo" Andrea GUIDOLIN (University of Southampton) N.B.: joint session with the "Topology Seminar" "Algebraic Wasserstein distances and stable homological invariants of data"

Friday, February 14th, 2025 h. 14:30 - Room "Roberta Dal Passo" Alessandro CAROTENUTO (Università di Parma) "Complex geometry of the full quantum flag manifold of quantum SU(3)"

Friday, January 17th, 2025 h. 14:30 - Room "Roberta Dal Passo" Frédéric PATRAS (Université Côte d'Azur) "How to recognize free Lie algebras?"

Friday, December 13th, 2024 h. 16:00 - Room "Roberta Dal Passo" Paolo ANTONINI (Università del Salento) "Optimal Transport between Algebraic Hypersurfaces"

Friday, December 13th, 2024 h. 14:30 - Room "Roberta Dal Passo" N.B.: joint session with the "Topology Seminar" Maxime RAMZI (Universität Münster) "Induced character formulae and the Becker-Gottlieb transfer"

Friday, November 29th, 2024 h. 16:00 - Room "Roberta Dal Passo" Özgür CEYHAN (Université du Luxembourg) "Tropical Neural Networks"

Friday, November 29th, 2024 h. 14:30 - Room "Roberta Dal Passo" Connor MALIN (Max Planck Institute) "A scanning map for the En operad"

Friday, November 15th, 2024 h. 16:00 - Room "Roberta Dal Passo" Alfonso TORTORELLA (Università di Salerno) "Deformations of Symplectic Foliations via Dirac Geometry and L∞-Algebra"

Friday, November 15th, 2024 h. 14:30 - Room "Roberta Dal Passo" Marco MORASCHINI (Università di Bologna) "An introduction to amenability in bounded cohomology"

Friday, October 25th, 2024 h. 16:00 - Room "Roberta Dal Passo" Anne MOREAU (Université Paris-Saclay) "On a series of simple affine VOAs arising from rank one 4D SCFTs"

Friday, October 25th, 2024 h. 14:30 - Room "Roberta Dal Passo" Linda HOYER (RWTH Aachen University) "Orthogonal Determinants of Finite Groups"

Friday, October 11th, 2024 h. 14:30 - Room "Claudio D'Antoni" N.B.: mind the change of room! Thibault JUILLARD (Université Paris-Saclay) "Reduction by stages for affine W-algebras"

Friday, September 27th, 2024 h. 16:00 - Room "Roberta Dal Passo" Gastón Andrés GARCÍA (Universidad Nacional de La Plata / CONICET) "Hopf algebras and finite simple groups of Lie type"

Friday, September 27th, 2024 h. 14:30 - Room "Roberta Dal Passo" Giulio RICCI "A brief introduction to Bruhat-Tits theory and its applications"

Friday, September 13th, 2024 h. 16:00 - Room "Roberta Dal Passo" Sabino DI TRANI ("Sapienza" Università di Roma) "Graph Cohomologies, Matroids and Colorings"

Friday, September 13th, 2024 h. 14:30 - Room "Roberta Dal Passo" Gastón Andrés GARCÍA (Universidad Nacional de La Plata / CONICET) "On the representation theory of generalized small quantum groups"

Monday, July 29th, 2024 h. 14:30 - Room "Roberta Dal Passo" Marianne DÉGLISE (ENS Paris Saclay) "Combinatorial study of coefficients of the decomposition theorem for universal linear degenerations"

Friday, July 5th, 2024 - ARTS Midsummer Day Edition h. 16:00 - Room "Roberta Dal Passo" Ping XU (Pennsylvania State University) "Derived differentiable manifolds"

Friday, July 5th, 2024 - ARTS Midsummer Day Edition h. 14:30 - Room "Roberta Dal Passo" Mathieu STIÉNON (Pennsylvania State University) "Formal geometry of groupoids"

Friday, June 7th, 2024 h. 14:30 - Room "Roberta Dal Passo" Paolo ASCHIERI (Università del Piemonte Orientale - Alessandria) "Bundles on quantum projective varieties and their differential geometry"

Friday, May 24th, 2024 h. 16:00 - Room "Claudio D'Antoni" N.B.: mind the change of room! Ulrich KRÄHMER (UT Dresden) "The ring of differential operators on a monomial curve is a Hopf algebroid"

Friday, May 24th, 2024 h. 14:30 - Room "Claudio D'Antoni" N.B.: (1) mind the change of room! (2) joint session with the department "Topology Seminar" Giovanni PAOLINI (Università di Bologna) "Dual Coxeter groups of rank three"

Friday, April 19th, 2024 h. 16:00 - Room "Roberta Dal Passo" N.B.: joint session with the department "Topology Seminar" Gabriele VEZZOSI (Università di Firenze) "Analogs of Beilinson-Drinfeld's Grassmannian on a surface"

Friday, May 10th, 2024 h. 14:30 - Room "Roberta Dal Passo" Stefano MARINI (Università di Parma) "On finitely Levi nondegenerate closed homogeneous CR manifolds"

Friday, April 19th, 2024 h. 16:00 - Room "Roberta Dal Passo" Elisabetta MASUT (Università di Padova) "Non-existence of integral Hopf orders for certain Hopf algebras"

Friday, April 19th, 2024 h. 14:30 - Room "Roberta Dal Passo" Martina COSTA CESARI (Università di Bologna) "Jordan classes and Lusztig strata in non-connected algebraic groups"

Friday, April 12th, 2024 h. 16:00 - Room "Roberta Dal Passo" Grant BARKLEY (University of Harvard) "Hypercube decompositions and combinatorial invariance for elementary intervals"

Friday, April 12th, 2024 h. 14:30 - Room "Roberta Dal Passo" Willem DE GRAAF (Università di Trento) "Classifying orbits of complex and real Vinberg representations"

Friday, March 15th, 2024 h. 16:00 - Room "Roberta Dal Passo" Christophe HOHLWEG (Université du Quebec à Montréal) "Shi arrangements in Coxeter groups"

Friday, March 15th, 2024 h. 14:30 - Room "Roberta Dal Passo" Anna MICHAEL (Universität Magdeburg) "Folded galleries - a museum tour through 192 years of math history"

Friday, March 1st, 2024 h. 16:00 - Room "Roberta Dal Passo" Matteo MICHELI ("Sapienza" Università di Roma) "Degenerations of the classical Grassmannians and their isotropic subvarieties"

Friday, March 1st, 2024 h. 14:30 - Room "Roberta Dal Passo" Timm PEERENBOOM (Ruhr-Universität - Bochum) "CoHas of extended Dynkin quivers"

Friday, February 16th, 2024 h. 16:00 - Room "Roberta Dal Passo" Loic FOISSY (LMPA-ULCO Calais) "Cointeracting bialgebras and applications to graphs"

Friday, February 16th, 2024 h. 14:30 - Room "Roberta Dal Passo" Rui XIONG (University of Ottawa) "Pieri rules over Grassmannians"

Friday, February 2nd, 2024 h. 16:00 - Room "Roberta Dal Passo" Giovanni CERULLI IRELLI ("Sapienza" Università di Roma) "Specialization map for quiver Grassmannians"

Friday, February 2nd, 2024 h. 14:30 - Room "Roberta Dal Passo" Roberto FRINGUELLI ("Sapienza" Università di Roma) "Zastava Spaces via non-degenerate maps"

Friday, January 19th, 2024 h. 16:00 - Room "Roberta Dal Passo" Tommaso ROSSI (Università di Roma "Tor Vergata") "Homology operations for gravity algebras"

Friday, January 19th, 2024 h. 14:30 - Room "Roberta Dal Passo" Riccardo ARAGONA (Università de L'Aquila) "Normalizer chain, modular idealizer chain and partitions"

Friday, December 15th, 2023 h. 16:00 - Room "Roberta Dal Passo" Tiziano GAIBISSO (Imperial College, London) "Nakajima quiver varieties"

Friday, December 15th, 2023 h. 14:30 - Room "Roberta Dal Passo" Chetan VUPPULURY ("Sapienza" Università di Roma) "Higher projective representations and higher central extensions"

Friday, December 1st, 2023 h. 16:00 - Room "Roberta Dal Passo" Sophie CHEMLA (Université Sorbonne - Paris Cité) "Duality properties for induced and coinduced representations in positive characteristic"

Friday, December 1st, 2023 h. 14:30 - Room "Roberta Dal Passo" Victoria SCHLEIS (University of Tübingen) "Tropical Quiver Grassmannians"

Friday, November 17th, 2023 h. 16:00 - Room "Roberta Dal Passo" Andrea RIVEZZI (Università di Milano "Bicocca") "Universal quantizations and the Drinfeld-Yetter algebra"

Friday, November 17th, 2023 h. 14:30 - Room "Roberta Dal Passo" Francesca PRATALI (Université Sorbonne - Paris Nord) "A tree-like approach to linear infinity operads"

Colloquium di Dipartimento Friday, November 3rd, 2023 h. 16:00 - Room "Roberta Dal Passo" Victor REINER (University of Minnesota)

"Combinatorics of configuration spaces - recent progress"

Friday, October 20th, 2023 h. 16:00 - Room "Roberta Dal Passo" Misha FEIGIN (University of Glasgow) "Quasi-invariants and free multi-arrangements"

Friday, October 20th, 2023 h. 14:30 - Room "Roberta Dal Passo" Luca FRANCONE (Université "Claude Bernard" Lyon 1) "Minimal monomial lifting of cluster algebras and branching problems"

Friday, October 6th, 2023 h. 16:00 - Room "Roberta Dal Passo" Francesco D'ANDREA (Università di Napoli "Federico II") "Operator Algebras That One Can See"

Friday, October 6th, 2023 h. 14:30 - Room "Roberta Dal Passo" Ryan AZIZ (Université Libre de Bruxelles) "Generalized Yetter-Drinfeld modules and the center construction of bimodule categories"

Friday, June 9th, 2023 h. 16:00 - Room "Roberta Dal Passo" Alessandro ZAMPINI (Università di Napoli "Federico II") "Derivation based differential calculus for a class of noncommutative spaces"

Friday, June 9th, 2023 h. 14:30 - Room "Roberta Dal Passo" Rosanna LAKING (Università di Verona) "Cosilting complexes and asymptotic triangulations of the annulus"

Friday, May 26th, 2023 h. 16:00 - Room "Roberta Dal Passo" Gleb NENASHEV (Brandeis University) "Schubert Calculus and bosonic operators"

Friday, May 26th, 2023 h. 14:30 - Room "Roberta Dal Passo" Arun RAM (University of Melbourne) "Boson-Fermion correspondence for Macdonald polynomials"

Friday, May 12th, 2023 h. 16:00 - Room "Roberta Dal Passo" Emanuele LATINI (Università di Bologna) "Higher conformal Yang-Mills equation"

Friday, May 12th, 2023 h. 14:30 - Room "Roberta Dal Passo" Thomas WEBER (Università di Torino) "Noncommutative differential geometry with Hopf algebra symmetry"

Friday, April 28th, 2023 h. 16:30 - Aula Magna "P. Gismondi" “Roberto Petronzio Lecture 2023” Giorgio PARISI (Accademia dei Lincei & "Sapienza" Università di Roma) "Multiple Equilibria"

- ARTS -
Algebra and Representation Theory Seminar

Friday, December 5th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Valerio MELANI [t.b.c.]
(Università di Firenze)

"TBA"

Friday, December 5th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Chris MILIONIS
(Newcastle University)

"TBA"

Friday, November 21th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Bernard LECLERC
(Université de Caen)

"TBA"

Friday, November 21th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Matilde MACCAN
(Ruhr-Universität Bochum)

"TBA"

Friday, November 7th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Lucia BAGNOLI
("Sapienza" Università di Roma)

"TBA"

Friday, November 7th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Alessio CIPRIANI
(Università di Verona)

"TBA"

Friday, October 24th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Giovanni CERULLI IRELLI
("Sapienza" Università di Roma)

"TBA"

Friday, October 24th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Antonio MITI
("Sapienza" Università di Roma)

"Construction and Reduction of the Lie infinity Algebra of Observables associated with a BV-Module"

Friday, October 10th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Jiefeng LIU
(Northeast Normal University, Changchun)

"Cohomology of restricted Poisson algebras in characteristic 2"

Friday, October 10th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Luca CASARIN
("Sapienza" Università di Roma)

"The factorizable Feigin-Frenkel center"

Friday, September 26th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Francesca PAGANELLI
("Sapienza" Università di Roma)

"Quantum cluster algebras and representations of shifted quantum affine algebras"

Friday, September 26th, 2025
h. 14:30 - Room "Roberta Dal Passo"
(joint session with the "Topology Seminar")

Alberto AVITABILE
(Università di Roma "Tor Vergata")

"E_∞-algebra structure on singular cochains"

Friday, June 20th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Nicolas RESSAYRE
(Université Lyon 1)

"An algorithm to compute the moment cones"

Friday, June 20th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Thomas GERBER
(Université Lyon 1)

"Atomic length on Weyl groups"

Thursday, June 5th, 2025
(( beware of the change of day and room!!! ))
h. 14:30 - Room 1200 (Biblioteca Storica)
N.B.: joint session with the "Topology Seminar"

Waclaw MARZANTOWICZ
(University of Poznań)

"Reeb graphs and description of homomorphisms onto the free groups"

Friday, May 23rd, 2025
h. 14:30 - Room "Roberta Dal Passo"

Santosha Kumar PATTANAYAK
(Indian Institute of Technology - Kanpur)

"Uniqueness of branching and unique factorization of tensor products of typical representations of Lie superalgebras"

Friday, April 11th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Tommaso SCOGNAMIGLIO
(Universität Heidelberg)

"PGL(2)-character varieties and Langlands duality over finite fields"

Friday, March 28th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Filippo AMBROSIO
(Friedrich-Schiller-Universität Jena)

"Étale geometry of Jordan classes closures"

Friday, March 14th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Lydia GÖSMANN
(Ruhr-Universität Bochum)

"Nakajima varieties of quivers"

Friday, February 28th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Damien SIMON
(Université Paris-Saclay)

"Chiral differential operators on a reductive group and representation theory"

Friday, February 28th, 2025
h. 14:30 - Room "Roberta Dal Passo"
N.B.: joint session with the "Topology Seminar"

Andrea PIZZI
(Università di Roma "Tor Vergata")

"(Multi-)Simplicial methods for Configuration Space Recognition"

Friday, February 14th, 2025
h. 16:00 - Room "Roberta Dal Passo"

Andrea GUIDOLIN
(University of Southampton)
N.B.: joint session with the "Topology Seminar"

"Algebraic Wasserstein distances and stable homological invariants of data"

Friday, February 14th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Alessandro CAROTENUTO
(Università di Parma)

"Complex geometry of the full quantum flag manifold of quantum SU(3)"

Friday, January 17th, 2025
h. 14:30 - Room "Roberta Dal Passo"

Frédéric PATRAS
(Université Côte d'Azur)

"How to recognize free Lie algebras?"

Friday, December 13th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Paolo ANTONINI
(Università del Salento)

"Optimal Transport between Algebraic Hypersurfaces"

Friday, December 13th, 2024
h. 14:30 - Room "Roberta Dal Passo"
N.B.: joint session with the "Topology Seminar"

Maxime RAMZI
(Universität Münster)

"Induced character formulae and the Becker-Gottlieb transfer"

Friday, November 29th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Özgür CEYHAN
(Université du Luxembourg)

"Tropical Neural Networks"

Friday, November 29th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Connor MALIN
(Max Planck Institute)

"A scanning map for the E_n operad"

Friday, November 15th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Alfonso TORTORELLA
(Università di Salerno)

"Deformations of Symplectic Foliations via Dirac Geometry and L_∞-Algebra"

Friday, November 15th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Marco MORASCHINI
(Università di Bologna)

"An introduction to amenability in bounded cohomology"

Friday, October 25th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Anne MOREAU
(Université Paris-Saclay)

"On a series of simple affine VOAs arising from rank one 4D SCFTs"

Friday, October 25th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Linda HOYER
(RWTH Aachen University)

"Orthogonal Determinants of Finite Groups"

Friday, October 11th, 2024
h. 14:30 - Room "Claudio D'Antoni"
N.B.: mind the change of room!

Thibault JUILLARD
(Université Paris-Saclay)

"Reduction by stages for affine W-algebras"

Friday, September 27th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Gastón Andrés GARCÍA
(Universidad Nacional de La Plata / CONICET)

"Hopf algebras and finite simple groups of Lie type"

Friday, September 27th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Giulio RICCI

"A brief introduction to Bruhat-Tits theory and its applications"

Friday, September 13th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Sabino DI TRANI
("Sapienza" Università di Roma)

"Graph Cohomologies, Matroids and Colorings"

Friday, September 13th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Gastón Andrés GARCÍA
(Universidad Nacional de La Plata / CONICET)

"On the representation theory of generalized small quantum groups"

Monday, July 29th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Marianne DÉGLISE
(ENS Paris Saclay)
"Combinatorial study of coefficients of the decomposition theorem for universal linear degenerations"

Friday, July 5th, 2024 - ARTS Midsummer Day Edition
h. 16:00 - Room "Roberta Dal Passo"

Ping XU
(Pennsylvania State University)
"Derived differentiable manifolds"

Friday, July 5th, 2024 - ARTS Midsummer Day Edition
h. 14:30 - Room "Roberta Dal Passo"

Mathieu STIÉNON
(Pennsylvania State University)
"Formal geometry of groupoids"

Friday, June 7th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Paolo ASCHIERI
(Università del Piemonte Orientale - Alessandria)
"Bundles on quantum projective varieties and their differential geometry"

Friday, May 24th, 2024
h. 16:00 - Room "Claudio D'Antoni"
N.B.: mind the change of room!

Ulrich KRÄHMER
(UT Dresden)
"The ring of differential operators on a monomial curve is a Hopf algebroid"

Friday, May 24th, 2024
h. 14:30 - Room "Claudio D'Antoni"
N.B.: (1) mind the change of room!
(2) joint session with the department "Topology Seminar"

Giovanni PAOLINI
(Università di Bologna)
"Dual Coxeter groups of rank three"

Friday, April 19th, 2024
h. 16:00 - Room "Roberta Dal Passo"
N.B.: joint session with the department "Topology Seminar"

Gabriele VEZZOSI
(Università di Firenze)
"Analogs of Beilinson-Drinfeld's Grassmannian on a surface"

Friday, May 10th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Stefano MARINI
(Università di Parma)
"On finitely Levi nondegenerate closed homogeneous CR manifolds"

Friday, April 19th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Elisabetta MASUT
(Università di Padova)
"Non-existence of integral Hopf orders for certain Hopf algebras"

Friday, April 19th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Martina COSTA CESARI
(Università di Bologna)
"Jordan classes and Lusztig strata in non-connected algebraic groups"

Friday, April 12th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Grant BARKLEY
(University of Harvard)
"Hypercube decompositions and combinatorial invariance for elementary intervals"

Friday, April 12th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Willem DE GRAAF
(Università di Trento)
"Classifying orbits of complex and real Vinberg representations"

Friday, March 15th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Christophe HOHLWEG
(Université du Quebec à Montréal)
"Shi arrangements in Coxeter groups"

Friday, March 15th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Anna MICHAEL
(Universität Magdeburg)
"Folded galleries - a museum tour through 192 years of math history"

Friday, March 1st, 2024
h. 16:00 - Room "Roberta Dal Passo"

Matteo MICHELI
("Sapienza" Università di Roma)
"Degenerations of the classical Grassmannians and their isotropic subvarieties"

Friday, March 1st, 2024
h. 14:30 - Room "Roberta Dal Passo"

Timm PEERENBOOM
(Ruhr-Universität - Bochum)
"CoHas of extended Dynkin quivers"

Friday, February 16th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Loic FOISSY
(LMPA-ULCO Calais)
"Cointeracting bialgebras and applications to graphs"

Friday, February 16th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Rui XIONG
(University of Ottawa)
"Pieri rules over Grassmannians"

Friday, February 2nd, 2024
h. 16:00 - Room "Roberta Dal Passo"

Giovanni CERULLI IRELLI
("Sapienza" Università di Roma)
"Specialization map for quiver Grassmannians"

Friday, February 2nd, 2024
h. 14:30 - Room "Roberta Dal Passo"

Roberto FRINGUELLI
("Sapienza" Università di Roma)
"Zastava Spaces via non-degenerate maps"

Friday, January 19th, 2024
h. 16:00 - Room "Roberta Dal Passo"

Tommaso ROSSI
(Università di Roma "Tor Vergata")
"Homology operations for gravity algebras"

Friday, January 19th, 2024
h. 14:30 - Room "Roberta Dal Passo"

Riccardo ARAGONA
(Università de L'Aquila)
"Normalizer chain, modular idealizer chain and partitions"

Friday, December 15th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Tiziano GAIBISSO
(Imperial College, London)
"Nakajima quiver varieties"

Friday, December 15th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Chetan VUPPULURY
("Sapienza" Università di Roma)
"Higher projective representations and higher central extensions"

Friday, December 1st, 2023
h. 16:00 - Room "Roberta Dal Passo"

Sophie CHEMLA
(Université Sorbonne - Paris Cité)
"Duality properties for induced and
coinduced representations in positive characteristic"

Friday, December 1st, 2023
h. 14:30 - Room "Roberta Dal Passo"

Victoria SCHLEIS
(University of Tübingen)
"Tropical Quiver Grassmannians"

Friday, November 17th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Andrea RIVEZZI
(Università di Milano "Bicocca")
"Universal quantizations and the Drinfeld-Yetter algebra"

Friday, November 17th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Francesca PRATALI
(Université Sorbonne - Paris Nord)
"A tree-like approach to linear infinity operads"

Colloquium di Dipartimento
Friday, November 3rd, 2023
h. 16:00 - Room "Roberta Dal Passo"
Victor REINER
(University of Minnesota)

Friday, October 20th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Misha FEIGIN
(University of Glasgow)
"Quasi-invariants and free multi-arrangements"

Friday, October 20th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Luca FRANCONE
(Université "Claude Bernard" Lyon 1)
"Minimal monomial lifting of cluster algebras and branching problems"

Friday, October 6th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Francesco D'ANDREA
(Università di Napoli "Federico II")
"Operator Algebras That One Can See"

Friday, October 6th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Ryan AZIZ
(Université Libre de Bruxelles)
"Generalized Yetter-Drinfeld modules and the center construction of bimodule categories"

Friday, June 9th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Alessandro ZAMPINI
(Università di Napoli "Federico II")
"Derivation based differential calculus for a class of noncommutative spaces"

Friday, June 9th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Rosanna LAKING
(Università di Verona)
"Cosilting complexes and asymptotic triangulations of the annulus"

Friday, May 26th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Gleb NENASHEV
(Brandeis University)
"Schubert Calculus and bosonic operators"

Friday, May 26th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Arun RAM
(University of Melbourne)
"Boson-Fermion correspondence for Macdonald polynomials"

Friday, May 12th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Emanuele LATINI
(Università di Bologna)
"Higher conformal Yang-Mills equation"

Friday, May 12th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Thomas WEBER
(Università di Torino)
"Noncommutative differential geometry with Hopf algebra symmetry"

Friday, April 28th, 2023
h. 16:30 - Aula Magna "P. Gismondi"
“Roberto Petronzio Lecture 2023”

Giorgio PARISI
(Accademia dei Lincei & "Sapienza" Università di Roma)
"Multiple Equilibria"

Friday, April 28th, 2023
h. 14:30 - Room "Roberta Dal Passo"

Ernesto SPINELLI
("Sapienza" Università di Roma)
"Hartley's Conjecture and development arising"

Friday, April 14th, 2023
h. 16:00 - Room "Roberta Dal Passo"

Filippo VIVIANI
(Università di Roma "Tor Vergata")
"On the Picard group of the stack of G-bundles on families of curves"